The area of a cyclic quadrilateral ABCD is 3√34 . The radius of the circle circumscribing cyclic quadrilateral is 1.If AB=1 and BD=√3, then BC⋅CD is equal to
Step by step video & image solution for The area of a cyclic quadrilateral ABCD is (3sqrt3)/4 . The radius of the circle circumscribing cyclic quadrilateral is 1.If AB =1 and BD =sqrt3, then BC*CD is equal to by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Similar Questions
The area of a cyclic quadrilateral ABCD is 3√34 . The radius of the circle circumscribing cyclic quadrilateral is 1.If AB=1 and BD=√3, then BC⋅CD is equal to
View Solution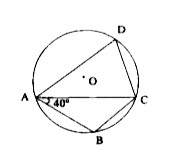
In the figure given above, O is the centre of a circle circumscribing a quadrilateral ABCD. If AB=BC and ∠BAC=40∘, then what is ∠ADC equal to ?
View Solution
Knowledge Check
ABCD is a cyclic quadrilateral such that AB is the diameter of the circle circumscribing it and ∠ADC=150∘. Then. ∠BAC is equal to:
A40∘B60∘C38∘D50∘ABCD is a cyclic quadrilateral such that AB is a diameter of the circle circumscribing it and angle ADC = 140∘, ∠BAC is equal to:
ABCD एक चक्रीय चतुर्भज है जो इस प्रकार है कि AB इसे घेरने वाले वृत्त का व्यास है तथा कोण ADC = 140∘, है | कोण ∠BAC किसके बराबर है?A38∘B40∘C50∘D60∘ABCD is a cyclic quadrilateral such that AB is a diameter of the circle circumscribing it and angle ADC=146∘.∠BAC is equal to:
A18∘B24∘C72∘D56∘
If the sides of a cyclic quadrilateral are 3,3,4,4, show that a circle be inscribed in it.
View SolutionIf the sides of a cyclic quadrilateral are 3,3,4,4, show that a circle be inscribed in it.
View SolutionIn cyclic quadrilateral ABCD, AD∣∣BC. Prove that AB = CD.
View SolutionABCD is a cyclic quadrilateral such that AB is a diameter of the circle circumscribing it and angle ADC=130∘ . Then angle BAC is equal to:
View SolutionABCD is a cyclic quadrilateral such that AB is diameter of the circle circumscirbing it and angle ADC=126∘.∠BAC is equal to
View Solution
