A conducting spherical shell having inner radius a and outer radius b carries a net charge Q. If a point charge q is placed at the centre of this shell, then the surface charge density on the outer surface of the shell is given as:
Q−p4πb2
Q+q4πb2
Q−q4πb2
Zero
To find the surface charge density on the outer surface of a conducting spherical shell with inner radius a and outer radius b, which carries a net charge Q and has a point charge q placed at its center, we can follow these steps:
Step 1: Understand the Charge Distribution
When a point charge q is placed at the center of a conducting spherical shell, it induces a charge on the inner surface of the shell. Since the shell is conducting, the electric field inside the conducting material must be zero. Thus, a charge of −q will be induced on the inner surface of the shell.
Hint: Remember that in a conductor, the electric field inside the conducting material is zero, leading to induced charges.
Step 2: Calculate the Charge on the Outer Surface
The total charge on the shell is Q. Since −q is induced on the inner surface, the charge on the outer surface of the shell can be calculated as follows:
Charge on outer surface=Q+q
Hint: The total charge on the shell is conserved; any induced charge on the inner surface affects the outer surface.
Step 3: Determine the Surface Charge Density
The surface charge density σ on the outer surface of the shell is defined as the charge per unit area. The area of the outer surface of the shell is given by the formula for the surface area of a sphere:
Area=4πb2
Thus, the surface charge density σ can be expressed as:
σ=Charge on outer surfaceArea of outer surface=Q+q4πb2
Hint: Surface charge density is calculated by dividing the total charge on the surface by the area of that surface.
Final Answer
The surface charge density on the outer surface of the shell is given by:
σ=Q+q4πb2
When a point charge q is placed at the center of a conducting spherical shell, it induces a charge on the inner surface of the shell. Since the shell is conducting, the electric field inside the conducting material must be zero. Thus, a charge of −q will be induced on the inner surface of the shell.
The total charge on the shell is Q. Since −q is induced on the inner surface, the charge on the outer surface of the shell can be calculated as follows:
Charge on outer surface=Q+q
The surface charge density σ on the outer surface of the shell is defined as the charge per unit area. The area of the outer surface of the shell is given by the formula for the surface area of a sphere:
Area=4πb2
Thus, the surface charge density σ can be expressed as:
σ=Charge on outer surfaceArea of outer surface=Q+q4πb2
The surface charge density on the outer surface of the shell is given by:
σ=Q+q4πb2
Topper's Solved these Questions
- ELECTROSTATICSBOOK - PHYSICS GALAXY - ASHISH ARORACHAPTER - ELECTROSTATICSEXERCISE - Numerical MCQs Single options Correct
64 Videos
- ELECTROSTATICSBOOK - PHYSICS GALAXY - ASHISH ARORACHAPTER - ELECTROSTATICSEXERCISE - Advance MCQs
40 Videos
- ELECTROSTATICSBOOK - PHYSICS GALAXY - ASHISH ARORACHAPTER - ELECTROSTATICSEXERCISE - Discussion Question
25 Videos
- ELECTROMAGNETIC INDUCTION AND ALTERNATING CURRENTBOOK - PHYSICS GALAXY - ASHISH ARORACHAPTER - ELECTROMAGNETIC INDUCTION AND ALTERNATING CURRENTEXERCISE - Advance MCQs
33 Videos
- GEOMETRICAL OPTICSBOOK - PHYSICS GALAXY - ASHISH ARORACHAPTER - GEOMETRICAL OPTICSEXERCISE - Unsolved Numerical Problems
107 Videos
Similar Questions
A spherical conducting shell of inner radius r1 and outer radius r2 has a charge Q.
(a) A charge q is placed at the centre of the shell. What is the surface charge density on the inner and outer surfaces of the shell ?
View SolutionA spherical conducting shell of inner radius r1 and outer radius r2 has a charge Q.
A charge q is placed at the centre of the shell. Find out the surface charge density on the inner and outer surface of the shell.
Is the electric field inside a cavity (with no charge ) zero. Independent of the fact wheather the shell is spherical or not ? Explain.
View Solution
Knowledge Check
A solid spherical conducting shell has inner radius a and outer radius 2a. At the center of the shell is located a point charge +Q. What must the excess charge of the shell be in order for the charge density on the inner and outer surface of the shell to be exactly equal?
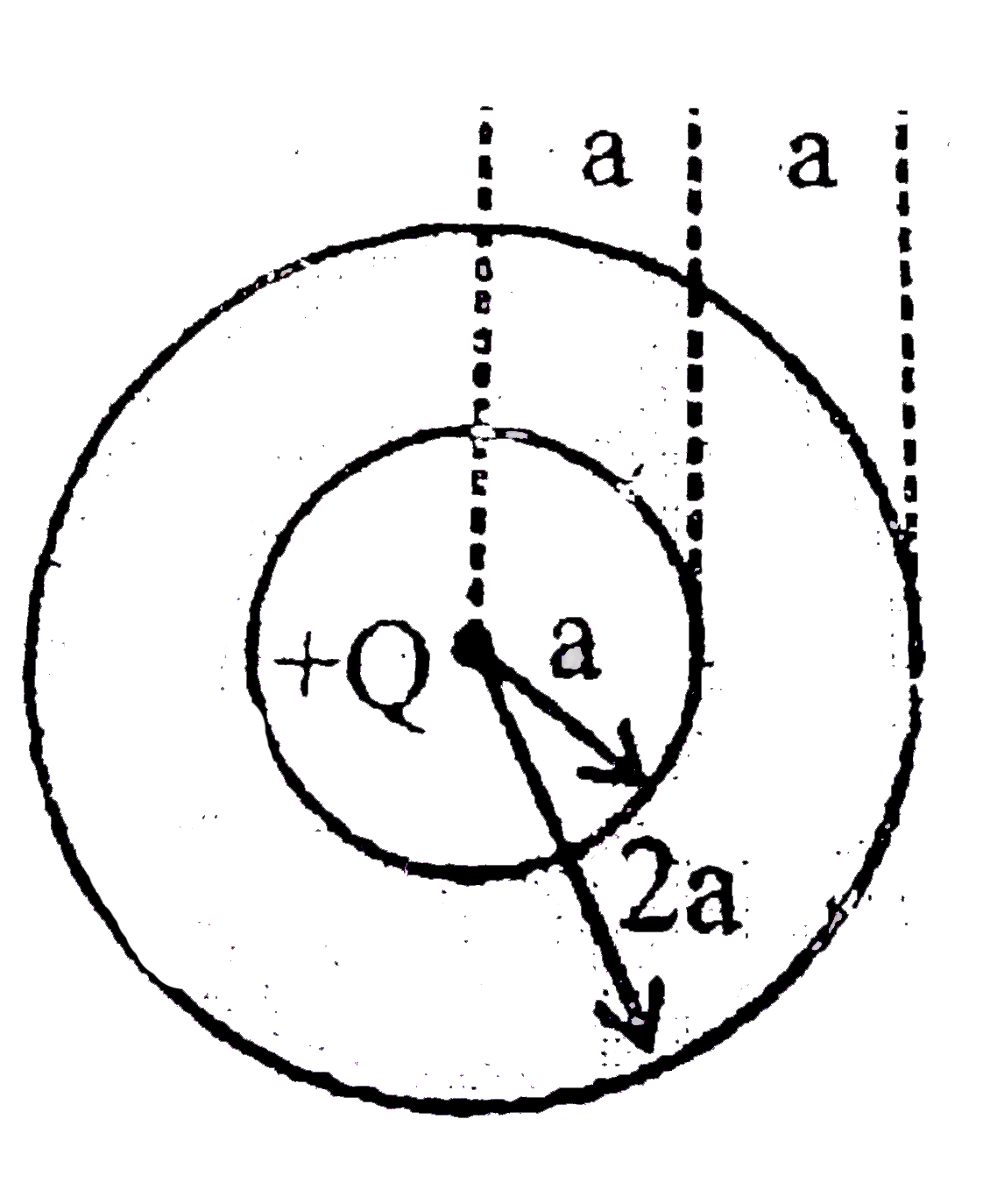 A−5QB+3QC−4QD+4Q
A−5QB+3QC−4QD+4Q
A spherical conducting shell of inner radius r1 and outer radius r2 has a charge Q:
(a) A charge q is placed at the centre of the shell. What is the surface charge density on the inner and outer surfaces of the shell?
(b) Is the electric field inside a cavity (with no charge) zero even if the shell is not spherical, but has any irregular shape? Explain.
View SolutionA spherical conducting shell of inner radius r1 and outer radius r2 has a charge Q.
(a) A charge q is placed at the center of the shell. What is the surface charge density on the inner and outer surfaces of the shell ?
(b) Is the electric field intensity inside a cavity (with no charge) zero, even if the shell is not spherical, but has any irregular shape ? Explain.
View SolutionA spherical conducting shell of inner radius r1 and outer radius r2 has a charges 'Q' A charges 'q' is placed at the centre of the shell.
What is the surface charges density on the (i) inner surface (ii) outer surface of the shell?
(b) A spherical conducting shell of inner radius r1 and outer radius r2 has a charges 'Q' A charges 'q' is placed at the centre of the shell.
Write the expression for the electric field at a point x>r2 from the centre of the shell.
View SolutionA spherical conducting shell of inner radius r1 and outer radius r2 ahs a charges 'Q' A charges 'q' is placed at the centre of the shell.
(a) What is the surface charges density on the (i) inner surface (ii) outer surface of the shell?
(ii) Write the expression for the electric field at a point x>r2 from the centre of the shell.
View SolutionA metallic spherical shell has an inner radius R1 and outer radius R2. A charge is placed at the centre of the spherical cavity. The surface charge density on the inner surface is

View SolutionA metalic spherical shell has an inner radius R1and outer radius R2 . A charge Q is placed at the centre of the spherical cavity. What will be surface charge density on (i) the inner surface and (ii) the outer surface?
View SolutionA metal spherical shell has an inner radius R1 and outer radius R2. A charge Q is placed at the center of the spherical cavity. What will be surface charge density on (i) the inner surface, and (ii) the outer surface ?
View Solution
