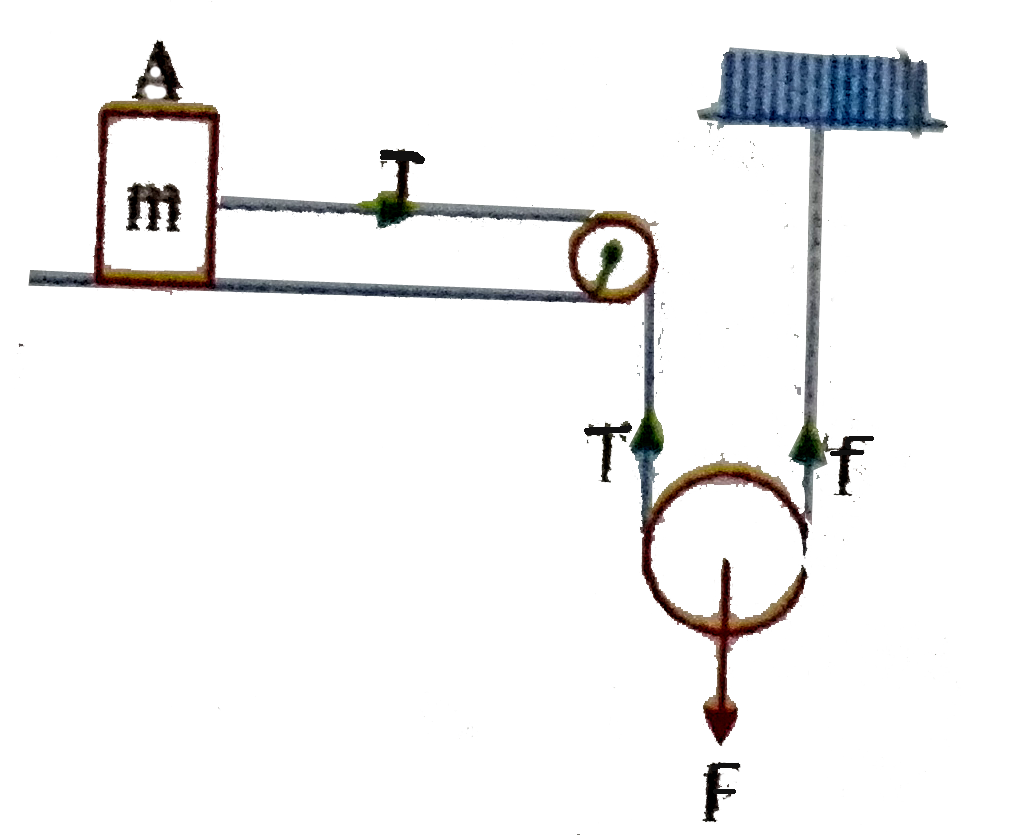
Effect of friction between pulley and thread :
In ideal cases i.e., when pulley and strings are massless and no friction exists at any contact surface, then tension in the string is constant throughout its length. But consider a, massless pulley and massless string but friction exists between pulley and string With coefficient . Then tension at the two ends of the pulley will be different. As shown in figure, consider an element of string :
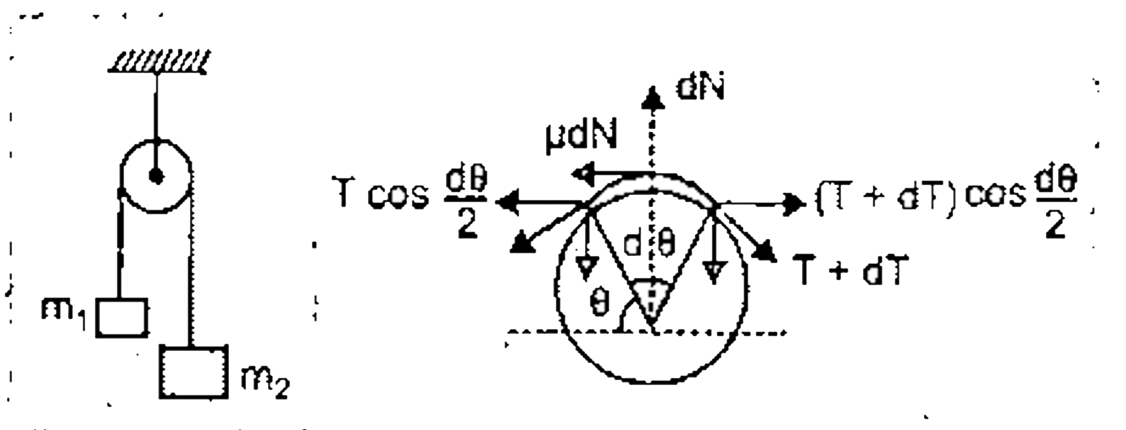
(massless strings)
int_(T_(1))^(T_(2)) (d T)/T=int _(0)^(pi)mu d theta rArr ln(T_(2)/T_(1))=mu pi rArrT_(2)/T_(1)=e^(mu pi)mu =1/pi
The tension on side ofheavier\nass will be:
A
B
C
D
Text Solution
The correct Answer is:c
|
Topper's Solved these Questions
- FORCE ANALYSISBOOK - ANURAG MISHRACHAPTER - FORCE ANALYSISEXERCISE - Matching type
13 Videos
- FORCE ANALYSISBOOK - ANURAG MISHRACHAPTER - FORCE ANALYSISEXERCISE - Level-2
73 Videos
- DESCRIPTION OF MOTIONBOOK - ANURAG MISHRACHAPTER - DESCRIPTION OF MOTIONEXERCISE - Level-3
34 Videos
- IMPULSE AND MOMENTUMBOOK - ANURAG MISHRACHAPTER - IMPULSE AND MOMENTUMEXERCISE - matching
3 Videos
Similar Questions
Knowledge Check
Effect of friction between pulley and thread :
In ideal cases i.e., when pulley and strings are massless and no friction exists at any contact surface, then tension in the string is constant throughout its length. But consider a, massless pulley and massless string but friction exists between pulley and string With coefficient μ. Then tension at the two ends of the pulley will be different. As shown in figure, consider an element of string :
dN=(T+dT)sindθ2+Tsindθ2
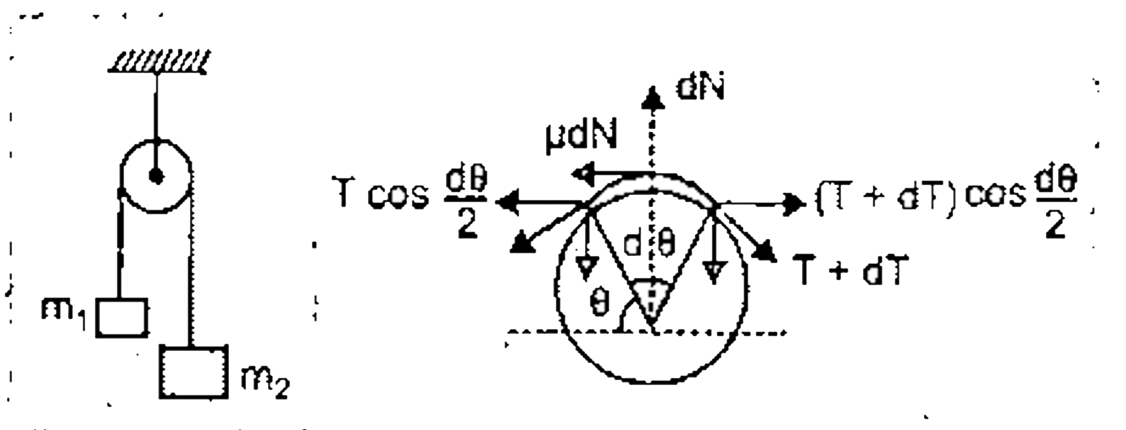
(T+dT)cosdθ2−Tcosdθ2−μdN=dra=0 (massless strings)
dTcosdθ2=μdN
dTcosdθ2=μ[(T+dt)sindθ2+Tsindθ2]
dt.cosdθ2=μ[Tsindθ2+dT.sindθ2+Tsindθ2]
dT=μ[T.dθ2+0+Tdθ2]=μTcosθ
int_(T_(1))^(T_(2)) (d T)/T=int _(0)^(pi)mu d theta rArr ln(T_(2)/T_(1))=mu pi rArrT_(2)/T_(1)=e^(mu pi)<br>Supposecofficientofictionbetweenthestr∈gandp≤––––yismu =1/pi
The tension on side of lighter mass will be:Am1gBm2gC2m2g3D4m1g3Effect of friction between pulley and thread :
In ideal cases i.e., when pulley and strings are massless and no friction exists at any contact surface, then tension in the string is constant throughout its length. But consider a, massless pulley and massless string but friction exists between pulley and string With coefficient μ. Then tension at the two ends of the pulley will be different. As shown in figure, consider an element of string :
dN=(T+dT)sindθ2+Tsindθ2
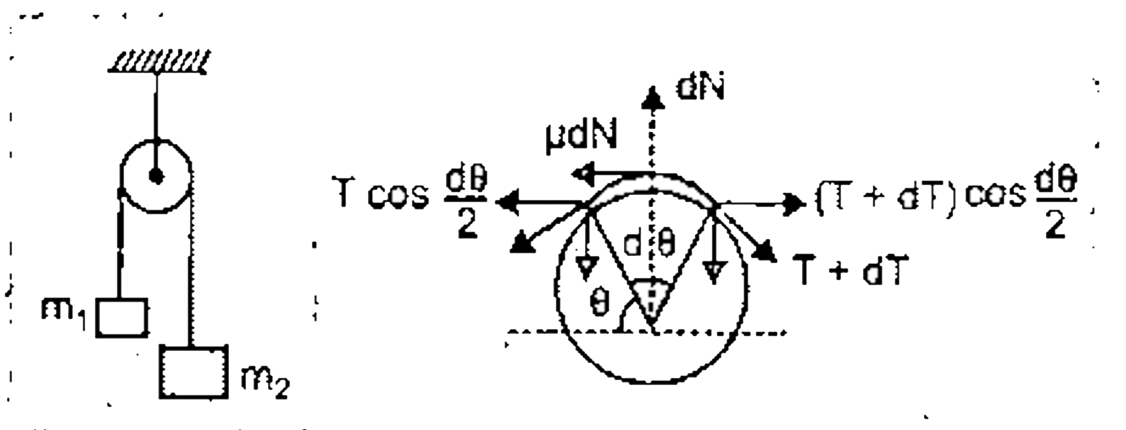
(T+dT)cosdθ2−Tcosdθ2−μdN=dra=0 (massless strings)
dTcosdθ2=μdN
dTcosdθ2=μ[(T+dt)sindθ2+Tsindθ2]
dt.cosdθ2=μ[Tsindθ2+dT.sindθ2+Tsindθ2]
dT=μ[T.dθ2+0+Tdθ2]=μTcosθ
int_(T_(1))^(T_(2)) (d T)/T=int _(0)^(pi)mu d theta rArr ln(T_(2)/T_(1))=mu pi rArrT_(2)/T_(1)=e^(mu pi)<br>Supposecofficientofictionbetweenthestr∈gandp≤––––yismu =1/pi<br>Ifm_(2)=2em then acceleration of each mass is :AgBg/3Ceg/3DzeroEffect of friction between pulley and thread :
In ideal cases i.e., when pulley and strings are massless and no friction exists at any contact surface, then tension in the string is constant throughout its length. But consider a, massless pulley and massless string but friction exists between pulley and string With coefficient μ. Then tension at the two ends of the pulley will be different. As shown in figure, consider an element of string :
dN=(T+dT)sindθ2+Tsindθ2
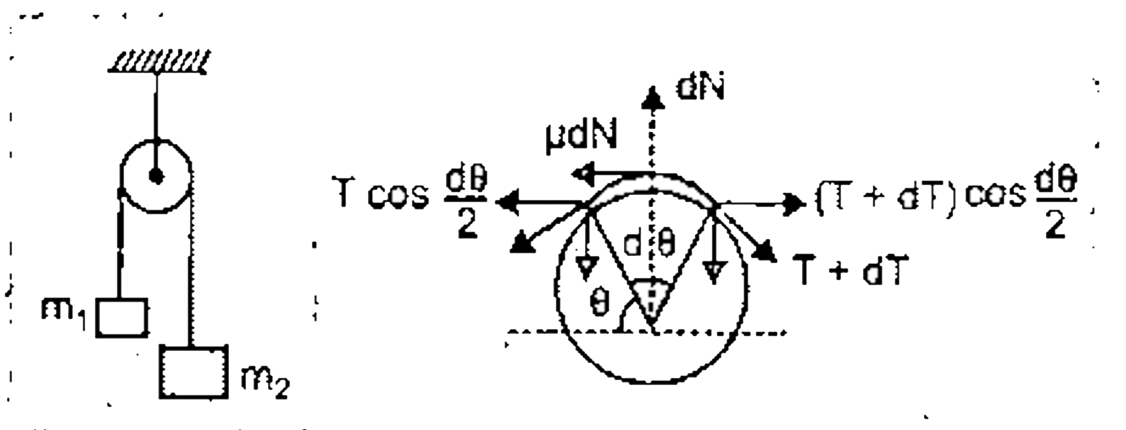
(T+dT)cosdθ2−Tcosdθ2−μdN=dra=0 (massless strings)
dTcosdθ2=μdN
dTcosdθ2=μ[(T+dt)sindθ2+Tsindθ2]
dt.cosdθ2=μ[Tsindθ2+dT.sindθ2+Tsindθ2]
dT=μ[T.dθ2+0+Tdθ2]=μTcosθ
int_(T_(1))^(T_(2)) (d T)/T=int _(0)^(pi)mu d theta rArr ln(T_(2)/T_(1))=mu pi rArrT_(2)/T_(1)=e^(mu pi)<br>Supposecofficientofictionbetweenthestr∈gandp≤––––yismu =1/pi
What should be the ratio of heavier mass to lighter mass for no motion ?AeB1eCeLDeR
Find the acceleration and tension in the string in the following cases . The pulley and string are massless.
(a)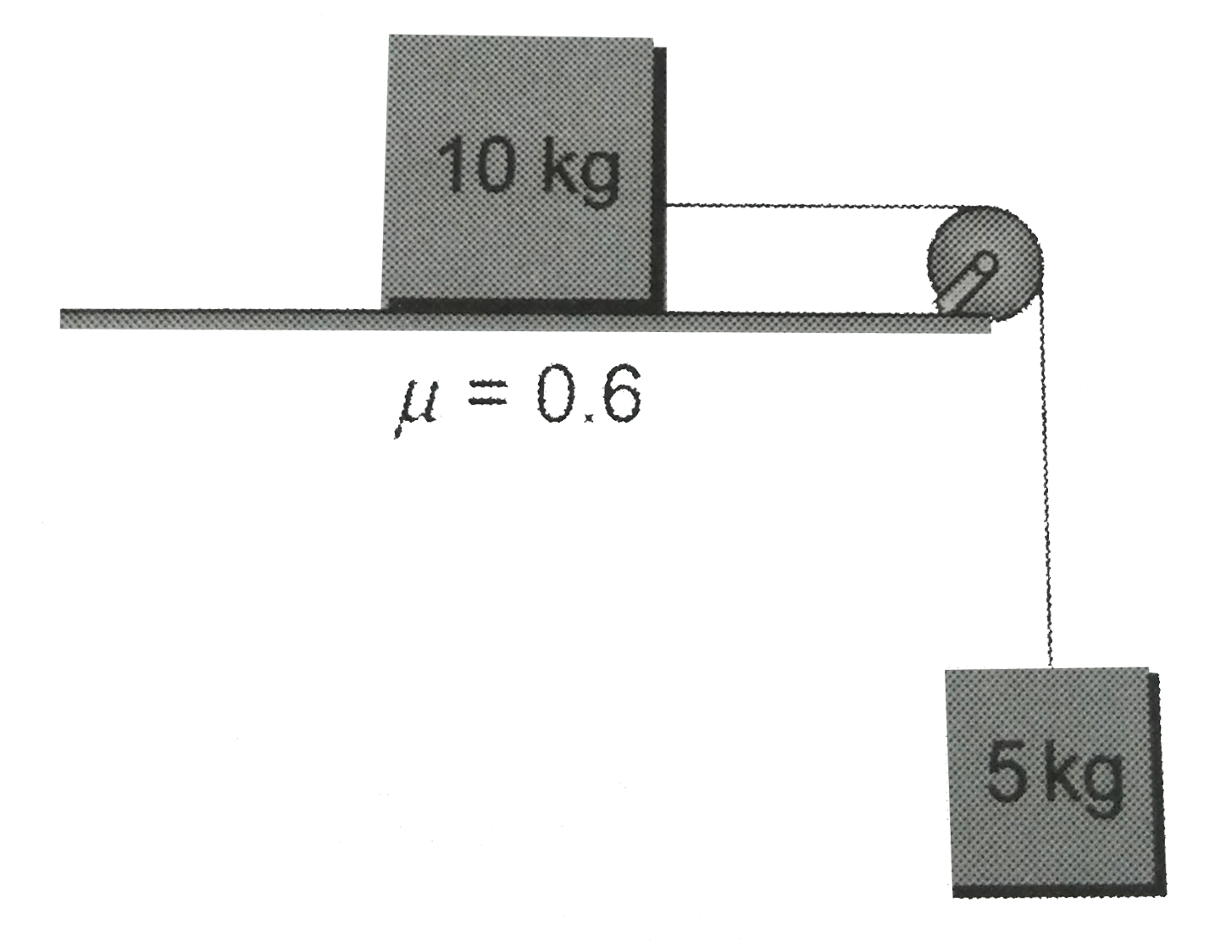
(b)
View SolutionIn the arrangement shown, the pulleys and strings are massless. The acceleration of block B is :
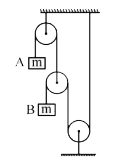
View SolutionThe tension in the string in the pulley system shown in
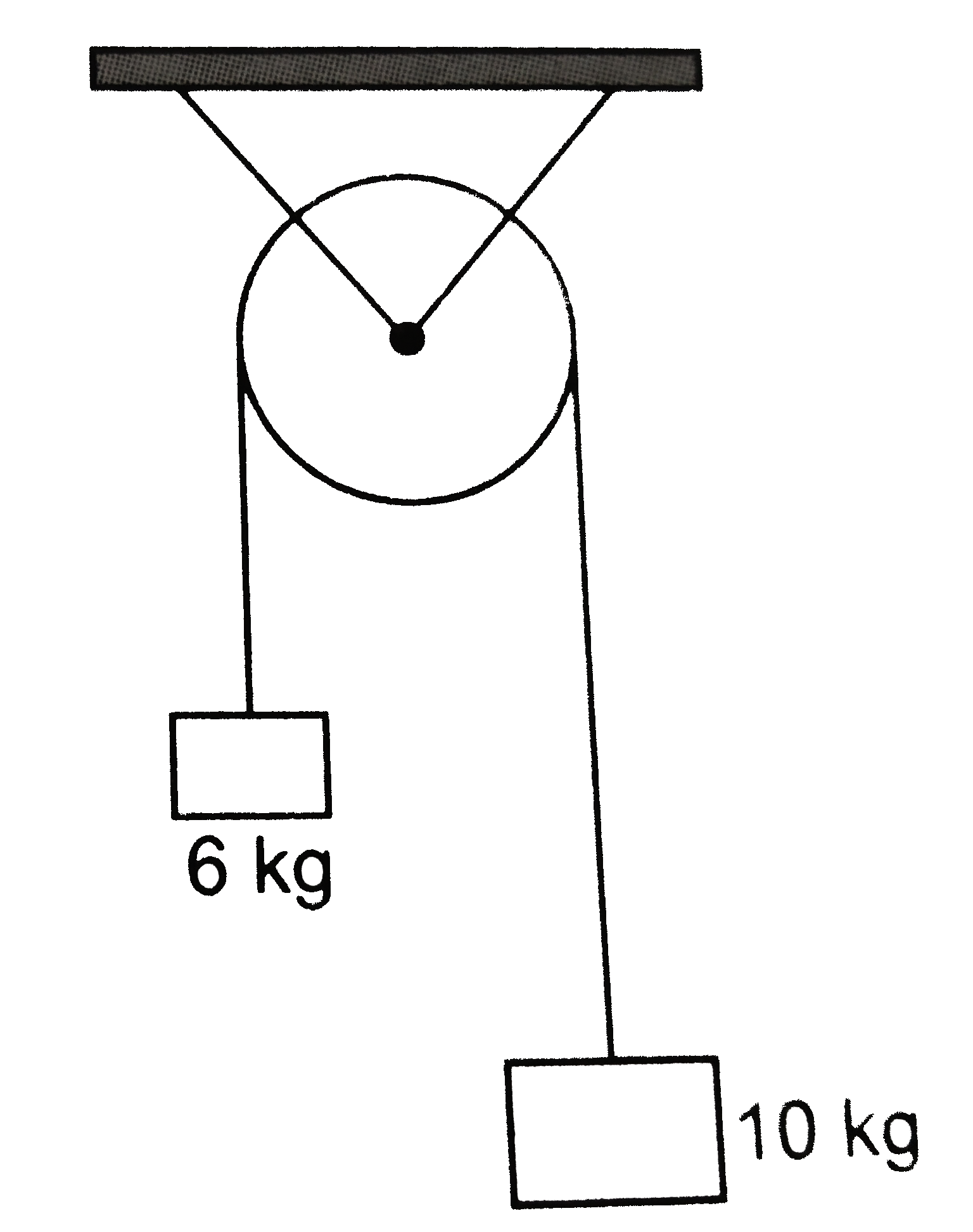 .
.
View SolutionPulleys and strings are massless. The horizontal surface is smooth. What is the acceleration of the block
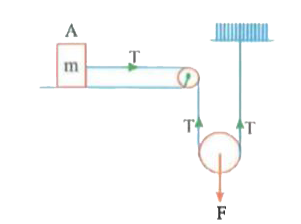
View SolutionPulleys and strings are massless.The horizontal surface is smooth.What is the acceleration of the block
View Solution

