Of the following lists of numbers , which has the largest standard deviation ?
2,7,15
3,7,14
5,7,12
10,11,12
The correct Answer is:A
To determine which of the given lists of numbers has the largest standard deviation, we will follow these steps for each list:
1. Calculate the Mean (μ): The mean is calculated by summing all the numbers in the list and dividing by the total count of numbers.
2. Calculate the Squared Differences: For each number in the list, subtract the mean and square the result.
3. Sum the Squared Differences: Add all the squared differences together.
4. Calculate the Variance: Divide the sum of squared differences by the number of values in the list.
5. Calculate the Standard Deviation: Take the square root of the variance.
Now, let's apply these steps to each of the four sets of numbers.
Set 1: {2, 7, 15}
1. Calculate the Mean (μ):
μ=2+7+153=243=8
2. Calculate the Squared Differences:
- (2−8)2=(−6)2=36
- (7−8)2=(−1)2=1
- (15−8)2=(7)2=49
3. Sum the Squared Differences:
36+1+49=86
4. Calculate the Variance:
Variance=863≈28.67
5. Calculate the Standard Deviation:
σ=√28.67≈5.36
Set 2: {3, 7, 14}
1. Calculate the Mean (μ):
μ=3+7+143=243=8
2. Calculate the Squared Differences:
- (3−8)2=(−5)2=25
- (7−8)2=(−1)2=1
- (14−8)2=(6)2=36
3. Sum the Squared Differences:
25+1+36=62
4. Calculate the Variance:
Variance=623≈20.67
5. Calculate the Standard Deviation:
σ=√20.67≈4.55
Set 3: {5, 7, 12}
1. Calculate the Mean (μ):
μ=5+7+123=243=8
2. Calculate the Squared Differences:
- (5−8)2=(−3)2=9
- (7−8)2=(−1)2=1
- (12−8)2=(4)2=16
3. Sum the Squared Differences:
9+1+16=26
4. Calculate the Variance:
Variance=263≈8.67
5. Calculate the Standard Deviation:
σ=√8.67≈2.94
Set 4: {10, 11, 12}
1. Calculate the Mean (μ):
μ=10+11+123=333=11
2. Calculate the Squared Differences:
- (10−11)2=(−1)2=1
- (11−11)2=(0)2=0
- (12−11)2=(1)2=1
3. Sum the Squared Differences:
1+0+1=2
4. Calculate the Variance:
Variance=23≈0.67
5. Calculate the Standard Deviation:
σ=√0.67≈0.82
Summary of Standard Deviations:
- Set 1: σ≈5.36
- Set 2: σ≈4.55
- Set 3: σ≈2.94
- Set 4: σ≈0.82
Conclusion:
The set with the largest standard deviation is Set 1: {2, 7, 15}.
1. Calculate the Mean (μ):
μ=2+7+153=243=8
- (2−8)2=(−6)2=36
- (7−8)2=(−1)2=1
- (15−8)2=(7)2=49
36+1+49=86
Variance=863≈28.67
σ=√28.67≈5.36
1. Calculate the Mean (μ):
μ=3+7+143=243=8
- (3−8)2=(−5)2=25
- (7−8)2=(−1)2=1
- (14−8)2=(6)2=36
25+1+36=62
Variance=623≈20.67
σ=√20.67≈4.55
1. Calculate the Mean (μ):
μ=5+7+123=243=8
- (5−8)2=(−3)2=9
- (7−8)2=(−1)2=1
- (12−8)2=(4)2=16
9+1+16=26
Variance=263≈8.67
σ=√8.67≈2.94
1. Calculate the Mean (μ):
μ=10+11+123=333=11
- (10−11)2=(−1)2=1
- (11−11)2=(0)2=0
- (12−11)2=(1)2=1
1+0+1=2
Variance=23≈0.67
σ=√0.67≈0.82
- Set 1: σ≈5.36
- Set 2: σ≈4.55
- Set 3: σ≈2.94
- Set 4: σ≈0.82
The set with the largest standard deviation is Set 1: {2, 7, 15}.
Topper's Solved these Questions
Similar Questions
Knowledge Check
- Question 1 - Select One
Which of the following expression has the largest value?
A(34)13B[(330)12]110C330+330+330D4(351)
What is the following sets has the larger standard deviation?
A={1,2,3,4,5}
B={1,4,15,21,27}View SolutionWhich of the following species has the largest bond angle?
View SolutionFor the distribution given in the following table, find the mean and the standard deviation by the step deviation method.
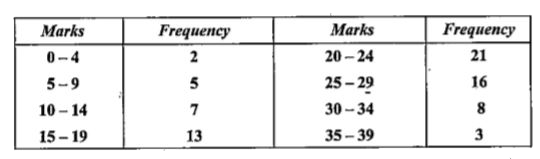 View Solution
View SolutionThe standard deviation of 25 numbers is 40. If each of the numbers in increased by 5, then the new standard deviation will be -
View SolutionWhich of the following has largest number of isomers?
View SolutionWhich among the following elements has the largest atomic radii ?
View SolutionWhich out of the following has the largest size ?
View Solution

