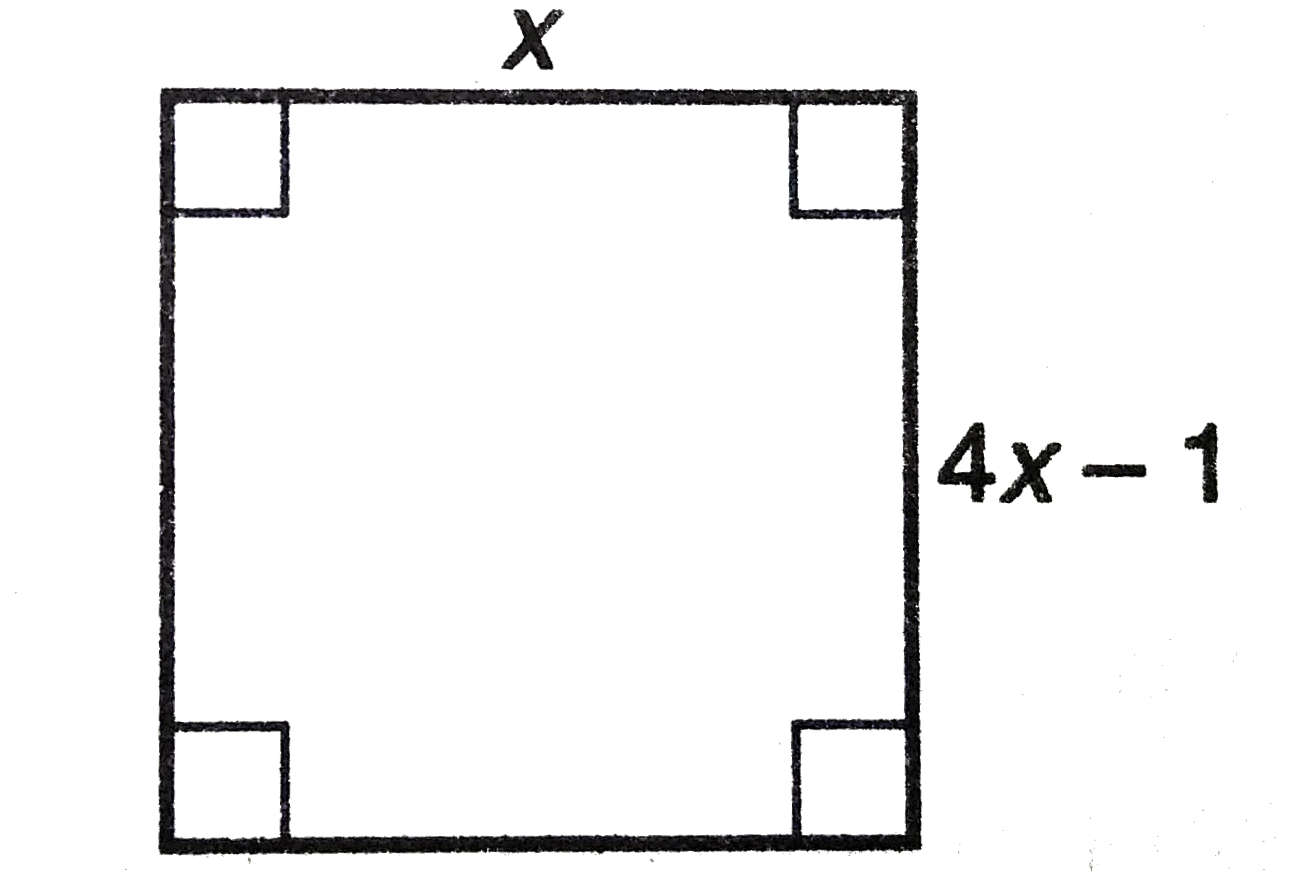
What is the area of square with an diagonal of ?
The correct Answer is:B
To find the area of a square with a diagonal of √2, we can follow these steps:
Step 1: Understand the relationship between the side and diagonal of a square.
The diagonal d of a square is related to its side length a by the formula:
d=a√2
Step 2: Set up the equation using the given diagonal.
We are given that the diagonal d is √2. Therefore, we can set up the equation:
a√2=√2
Step 3: Solve for the side length a.
To find a, we can divide both sides of the equation by √2:
a=√2√2=1
Step 4: Calculate the area of the square.
The area A of a square is given by the formula:
A=a2
Substituting a=1 into the area formula:
A=12=1
Conclusion:
The area of the square is 1.
---
The diagonal d of a square is related to its side length a by the formula:
d=a√2
We are given that the diagonal d is √2. Therefore, we can set up the equation:
a√2=√2
To find a, we can divide both sides of the equation by √2:
a=√2√2=1
The area A of a square is given by the formula:
A=a2
Substituting a=1 into the area formula:
A=12=1
The area of the square is 1.
Topper's Solved these Questions
- ADDITIONAL TOPICS IN MATHBOOK - ENGLISH SATCHAPTER - ADDITIONAL TOPICS IN MATHEXERCISE - Grib-In
32 Videos
- ADDITIONAL TOPICS IN MATHBOOK - ENGLISH SATCHAPTER - ADDITIONAL TOPICS IN MATHEXERCISE - EXERCISE
45 Videos
- ADDITIONAL TOPICS IN MATHBOOK - ENGLISH SATCHAPTER - ADDITIONAL TOPICS IN MATHEXERCISE - EXERCISE
45 Videos
- ADDITIONAL TOPICS IN MATH BOOK - ENGLISH SATCHAPTER - ADDITIONAL TOPICS IN MATH EXERCISE - PRACTICE TEST
24 Videos
- COMPLEX NUMBERSBOOK - ENGLISH SATCHAPTER - COMPLEX NUMBERSEXERCISE - EXERCISE
6 Videos
Similar Questions
Knowledge Check
If the perimeter of a square is 28, what is the length of the diagonal of the square?
A2√14B7√2C7√3D14
What is the diagonal of a square with an area of 25?
View SolutionWhat is the area of a square whose sides are doubled?
View SolutionFind the area and the perimeter of a square with diagonal 24 cm.
[Take √2=1.41].
View SolutionWhat is the area, in square units, of an isosceles right triangle with a hypotenous of 2?
View SolutionThe side of an equilatetral triangle has the same length as the diagonal of a square. What is the area of the square?
(1) The height of the equilateral triangle is equal to 6√3.
(2) The area of the equilateral triangle is equal to 36√3.
View SolutionIf the coordinates of the endpoints of a diagonal of square are (−2,−3)and(5,4), what is the number of square units in the area of the square?
View SolutionA planet travels in an elliptical orbit about a star as shown. At what pair of points is the speed of the planet the same?
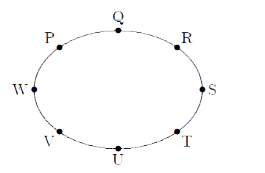
View Solution