A proton of mass $m$ and charge e is projected from a very large distance towards an $α$ particle with velocity $v$ . Intially, particle is at rest, but it is free to move. If gravity is neglected, then the minimum separation along the straight line of their motion will be

A

$e^{2} / 4 π ε_{0} m v^{2}$

B

$5 e^{2} / 4 π ε_{0} m v^{2}$

C

$2 e^{2} / 4 π ε_{0} m v^{2}$

D

$4 e (2) / 4 π ε m v^{2}$

Text Solution

Verified by Experts

The correct Answer is:B

|

Updated on:21/07/2023

Class 12 PHYSICS QUESTION BANK

Topper's Solved these Questions

BOOK - KVPY PREVIOUS YEARCHAPTER - KVPY 2021EXERCISE - PART I (Chemistry)
2videos
KVPY 2021

Similar Questions

Knowledge Check

Question 1 - Select One
A particle of mass m and charge $- q$ is projected from the origin with a horizontal speed v into an electric field of intensity E directed downward. Choose the wrong statement. Neglect gravity

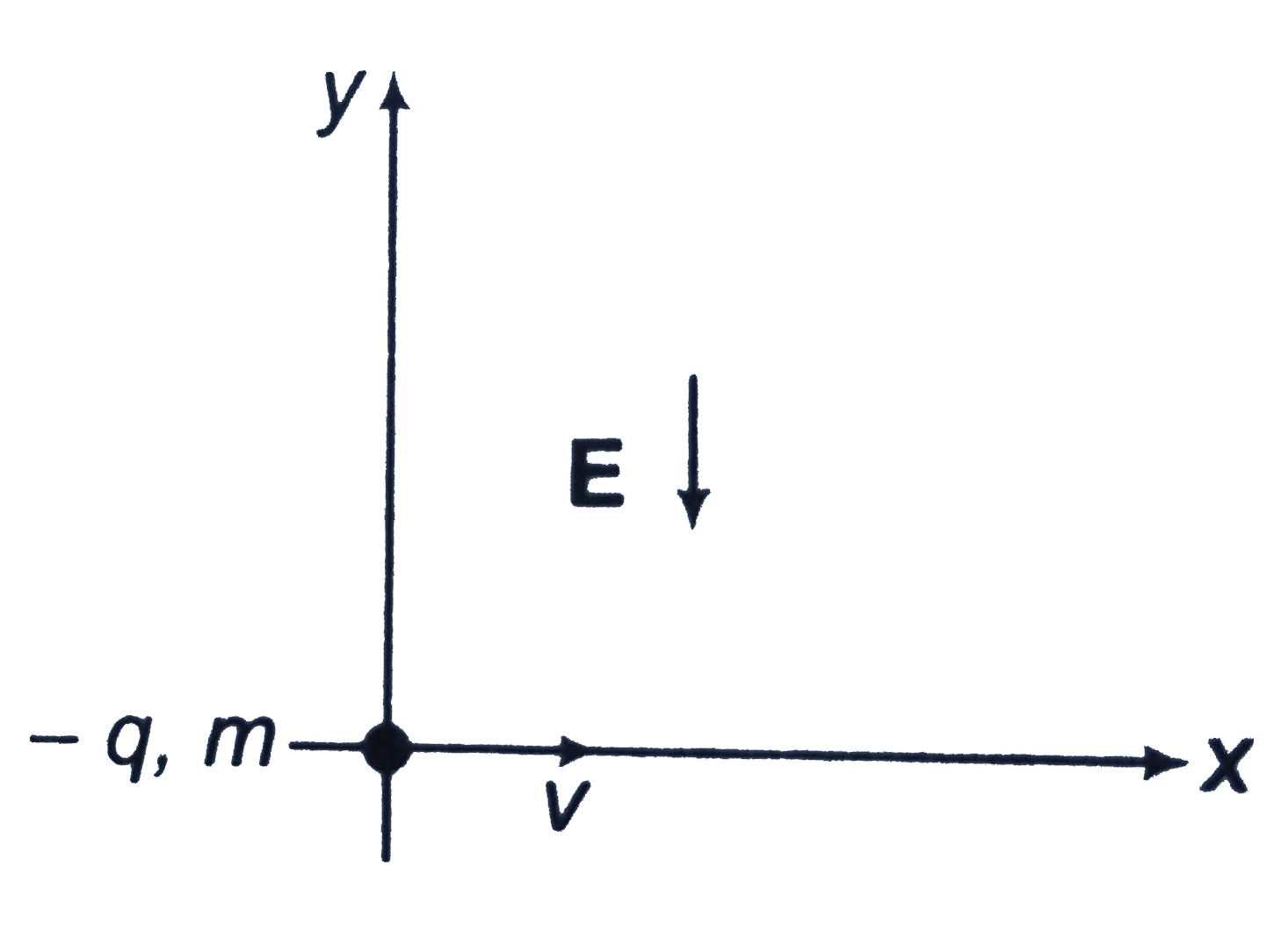AThe kinetic energy after a displacement y is $q E y$
BThe horizontal and vertical components of acceleration of $a_{x} = 0, a_{y} = \frac{q E}{m}$
Cthe equation of trajectory is $y = \frac{1}{2} (\frac{q E x^{2}}{m v^{2}})$
DThe horizontall and verticasl displacements x and y after a time t x=vt and $y = \frac{1}{2} a_{y} t^{2}$
Question 2 - Select One
A particle of mass m is projected upword with velocity $v = \frac{v_{e}}{2}$ ( $v_{e})$ escape of the particle is
A $- \frac{G M m}{2 R}$
B $- \frac{G M m}{4 R}$
C $- \frac{3 G M m}{4 R}$
D $- \frac{2 G M m}{3 R}$
Question 3 - Select One
The closed distance of approach of an $α -$ particle travelling with velocity V towards a stationary nucleus is $d$ .For the closest distance to become $\frac{d}{3}$ towards a stationary nucleus of double of the charge,the velocity of projection of the $α -$ particle has to be
A6V
B $\sqrt{6} V$
C $\frac{V}{56}$
D $\frac{3 V}{2}$

KVPY PREVIOUS YEAR-KVPY-All Questions

Home
Ask Doubt
Profile