A particle of mass m is projected upword with velocity v=ve2 (ve) escape of the particle is
−GMm2R
−GMm4R
−3GMm4R
−2GMm3R
The correct Answer is:C
To solve the problem of finding the potential energy of a particle projected upward with a velocity of v=ve2, where ve is the escape velocity, we can follow these steps:
Step 1: Understand Escape Velocity
The escape velocity ve is given by the formula:
ve=√2GMR
where G is the universal gravitational constant, M is the mass of the Earth, and R is the radius of the Earth.
Step 2: Substitute for Given Velocity
In the problem, the particle is projected with a velocity:
v=ve2=12√2GMR=√GM2R
Step 3: Calculate Initial Kinetic Energy
The initial kinetic energy KE of the particle can be calculated using the formula:
KE=12mv2
Substituting for v:
KE=12m(√GM2R)2=12m⋅GM2R=mGM4R
Step 4: Calculate Initial Potential Energy
The initial potential energy PE of the particle when it is at the surface of the Earth is given by:
PE=−GMmR
Step 5: Total Initial Energy
The total initial energy Ei is the sum of kinetic and potential energy:
Ei=KE+PE=mGM4R−GMmR
To combine these, we need a common denominator:
Ei=mGM4R−4mGM4R=mGM4R−4mGM4R=−3mGM4R
Step 6: Maximum Height and Final Energy
At the maximum height, the velocity of the particle becomes zero, meaning all the initial energy has converted into potential energy. Therefore, the potential energy at maximum height PEmax is equal to the total initial energy:
PEmax=Ei=−3mGM4R
Conclusion
Thus, the potential energy of the particle at its maximum height is:
PEmax=−3GMm4R
Answer
The potential energy of the particle at maximum height is:
−3GMm4R
This corresponds to option C.
---
The escape velocity ve is given by the formula:
ve=√2GMR
where G is the universal gravitational constant, M is the mass of the Earth, and R is the radius of the Earth.
In the problem, the particle is projected with a velocity:
v=ve2=12√2GMR=√GM2R
The initial kinetic energy KE of the particle can be calculated using the formula:
KE=12mv2
Substituting for v:
KE=12m(√GM2R)2=12m⋅GM2R=mGM4R
The initial potential energy PE of the particle when it is at the surface of the Earth is given by:
PE=−GMmR
The total initial energy Ei is the sum of kinetic and potential energy:
Ei=KE+PE=mGM4R−GMmR
To combine these, we need a common denominator:
Ei=mGM4R−4mGM4R=mGM4R−4mGM4R=−3mGM4R
At the maximum height, the velocity of the particle becomes zero, meaning all the initial energy has converted into potential energy. Therefore, the potential energy at maximum height PEmax is equal to the total initial energy:
PEmax=Ei=−3mGM4R
Thus, the potential energy of the particle at its maximum height is:
PEmax=−3GMm4R
The potential energy of the particle at maximum height is:
−3GMm4R
This corresponds to option C.
Topper's Solved these Questions
Similar Questions
A particle of mass m is projected upwards with velocity v=ve2, where ve is the escape velocity then at the maximum height the potential energy of the particle is : (R is radius of earth and M is mass of earth)
View SolutionA particle of mass'm' is projected with velocity v0 at an angle 'α' with the horizontal. The coefficient of restitution for any of its impact with the smooth ground is e.
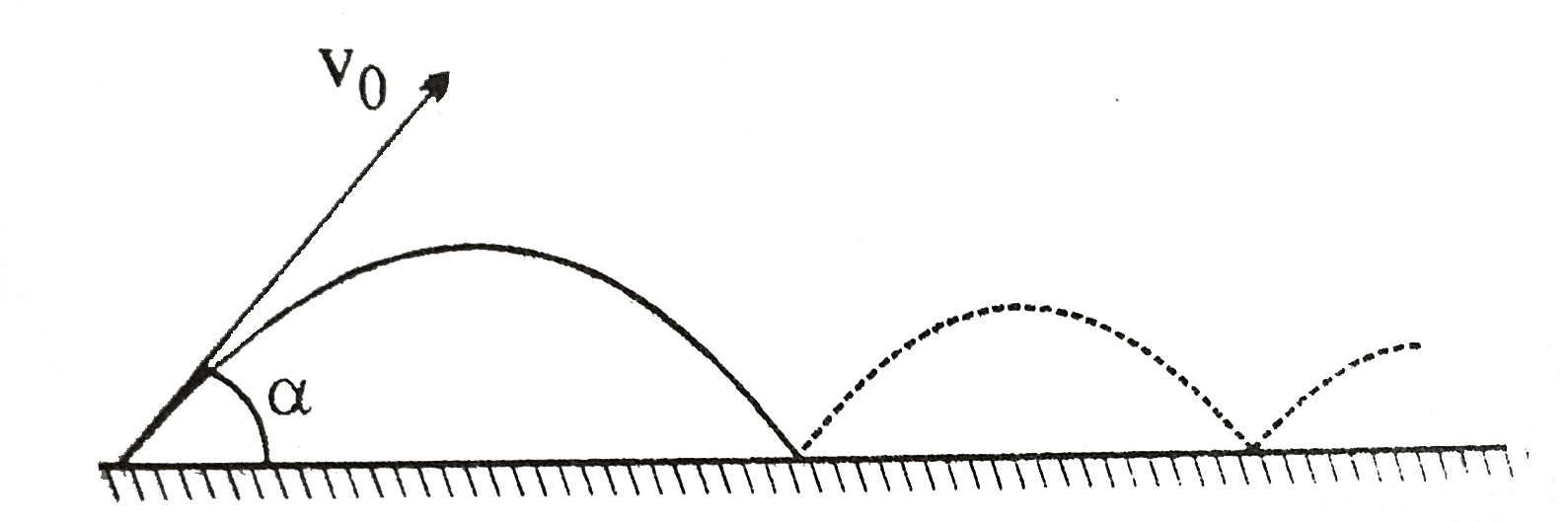
Find total time taken by the particle before it stops moving vertically?
View SolutionTwo spheres of masses mand2m are separated by distance d. A particle of mass m5 is projected straight from 2m towards m with a velocity v0. Which of the following statements is correct ?
View SolutionA particle is projected with velocity v0 along x - axis. The deceleration on the particle is proportional to the square of the distance from the origin i.e. a=−x2. The distance at which particle stops is -
View SolutionA particle of mass m is projected with a velocity v at an angle of θ with horizontal. The angular momentum of the particle at the highest point of its trajectory is equal to :
View SolutionA particle of mass m is projected with a velocity v making an angle of 45∘ with the horizontal. The magnitude of the angular momentum of the projectile abut the point of projection when the particle is at its maximum height h is.
View SolutionA particle of mass m is projected with velocity v moving at an angle of 45∘ with horizontal. The magnitude of angular momentum of projectile about point of projection when particle is at maximum height, is
View SolutionA positively charged particle of charge q and mass m is suspended from a point by a string of length l. In the space a uniform horizontal electric field E exists . The particle is drawn aside so that it is projected horizontally with velocity v such that the particle starts to move along a circle with same constant speed v. Find the speed v.
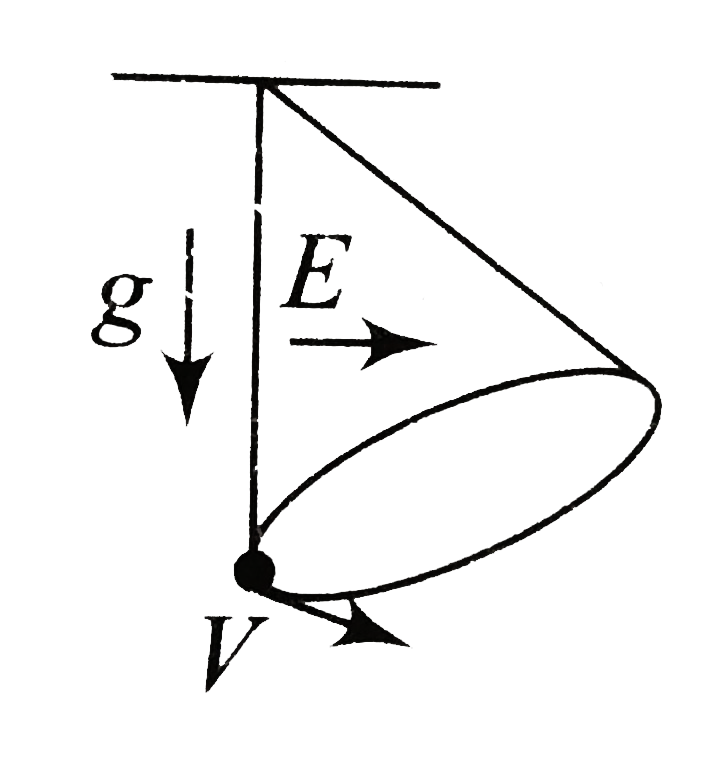
View SolutionA particle of mass 'm' is projected from the surface of earth with velocityυ=2υe, where υe is the value of escape velocity from the surface of earth . Find velocity of the particle on reaching to interstellar space (at infinity) in terms of υe.
View SolutionA particle of mass m1 is moving with a velocity v1 and another particle of mass m2 is moving with a velocity v2. Both of them have the same momentum but their different kinetic energies are E1 and E2 respectively. If m1>m2 then
View Solution
