In a newly developed city, it is estimated that the voting population (in thousands) will increase according to V(t)=30+12t2−t3,0≤t≤8 where t is the time in years. Find the approximate change in voters for the time change from 4 to 4(16) year. Solution in Tamil
Step by step video & image solution for In a newly developed city, it is estimated that the voting population (in thousands) will increase according to V(t) = 30 + 12t^(2)-t^(3), 0 le t le 8 where t is the time in years. Find the approximate change in voters for the time change from 4 to 4(1/6) year. by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- DIFFERENTIALS AND PARTIAL DERIVATIVES BOOK - SURA PUBLICATIONCHAPTER - DIFFERENTIALS AND PARTIAL DERIVATIVES EXERCISE - EXERCISE 8.3
7 Videos
- DIFFERENTIALS AND PARTIAL DERIVATIVES BOOK - SURA PUBLICATIONCHAPTER - DIFFERENTIALS AND PARTIAL DERIVATIVES EXERCISE - EXERCISE 8.4
10 Videos
- DIFFERENTIALS AND PARTIAL DERIVATIVES BOOK - SURA PUBLICATIONCHAPTER - DIFFERENTIALS AND PARTIAL DERIVATIVES EXERCISE - 5 MARKS
4 Videos
- COMPLEX NUMBERSBOOK - SURA PUBLICATIONCHAPTER - COMPLEX NUMBERSEXERCISE - ADDITIONAL QUESTIONS (5 MARKS )
6 Videos
- DISCRETE MATHEMATICS BOOK - SURA PUBLICATIONCHAPTER - DISCRETE MATHEMATICS EXERCISE - 5 MARKS
2 Videos
Similar Questions
Evaluate lim(x,y)→(1,2), g(x,y) , if the limit exist where g(x,y) =3x2−xyx2+y2+3
View SolutionFind the partial derivatives of the functions at the indicated point
f(x,y)=3x2−2xy+y2+5x+2,(2,−5)
View SolutionIf u=tan−1(x3+y3x−y), Prove that x∂u∂x+y∂u∂y=sin2u.
View SolutionIf 1, ωω2 are the cube roots of unity then show that (1+5ω2+ω4)(1+5ω+ω2)(5+ω+ω5) = 64.
View SolutionLet S be a non-empty set and 0 be a binary operation on s defined by x 0 y =x, x, y∈S. Determine whether 0 is commutative and association.
View SolutionA particle starts moving rectilinearly at time t=0 such that its velocity v changes with time t according to the equation v=t^(2)-t , where t is in seconds and v in s^(-1) . Find the time interval for which the particle retards.
View SolutionA particle starts moving rectilinearly at time t = 0 such that its velocity(v) changes with time (t) as per equation – v = (t2 – 2t) m//s for 0 lt t lt 2 s = (–t^(2) + 6t – 8) m//s for 2 le te 4 s (a) Find the interval of time between t = 0 and t = 4 s when particle is retarding. (b) Find the maximum speed of the particle in the interval 0 le t le 4 s .
View SolutionSuppose that a particle mvoes on a straight line so that is velocity at time t is v(t)=(t^(2)-2t)m//s . (a). Find the displacement of the particle during the time interval 0 le t le 3 (b). Find the distance traveled by the particle during the time interval 0 le t le 3 .
View Solution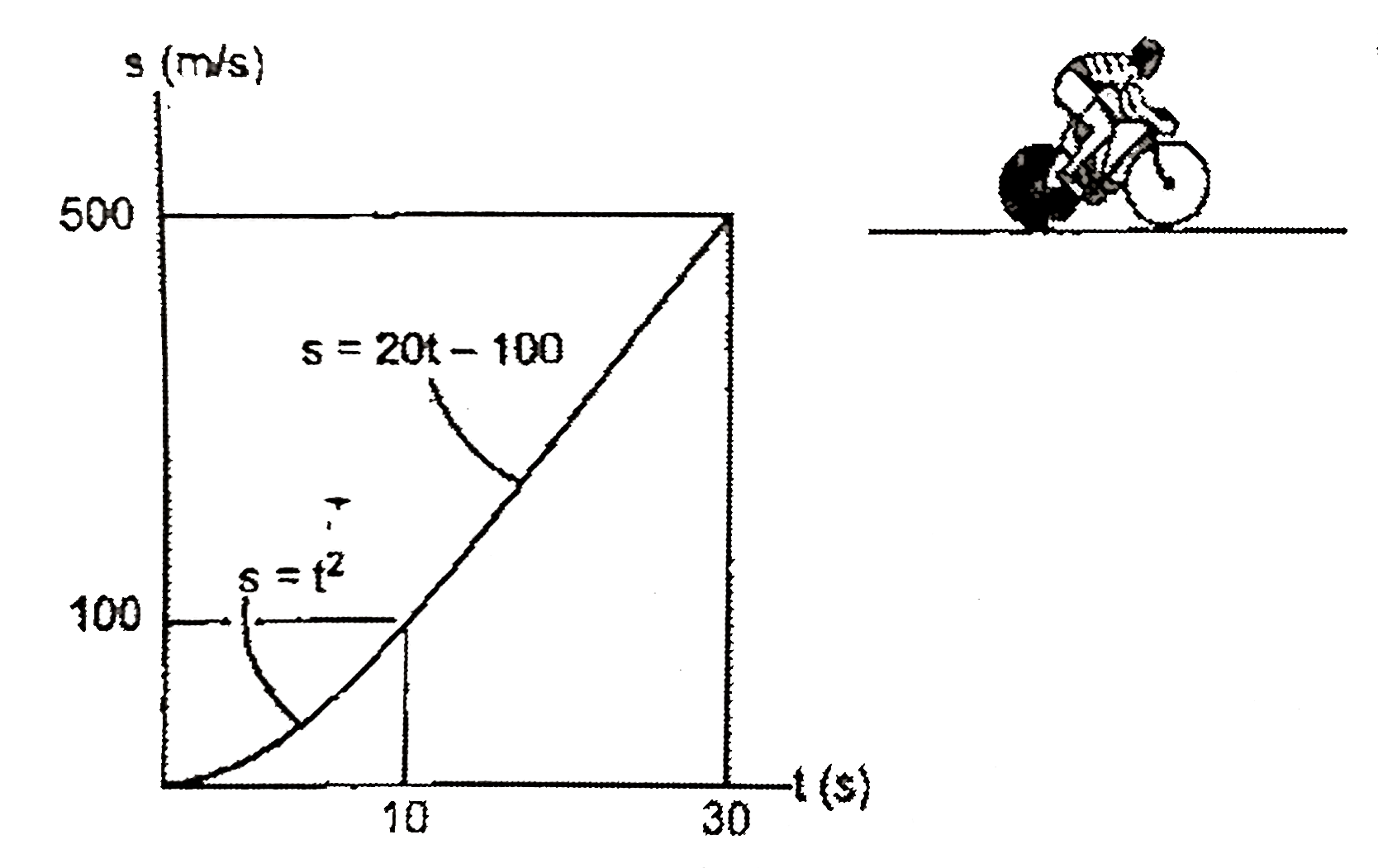
A bicycle moves along a stragith road such that its construct the v-t and a-t graphs for 0≤t≤30.
View SolutionIf the rate of increase in population of is r% per year, the population after t years is p, Find the population that was t years before.
View SolutionThe population of a city increase at a rate proportional to the population at that time. In 40 years the population is increased from 30,000 to 40,000 then after time t, the population is
View SolutionThe population of a city increases at the rate 3% per year. If at time t the population of city is p, then find equation of p in time t.
View Solutionபுதிதாக உருவாக்கப்பட்ட ஒரு நகரத்தின் வாக்காளர்களின் எண்ணிக்கையின் (ஆயிரங்களில்) அதிகரிப்பு V(t)=30+12t2−t3,0≤t≤8 என்பதால் மதிப்பிடப்படுகின்றது. இங்கு t என்பது ஆண்டுகளை குறிக்கின்றது காலம் 4-இலிருந்து 416வருடமாக இருக்கும் போது ஏற்படும் தோராய வாக்காளர்களின் எண்ணிக்கை மாற்றத்தைக் காண்க.
View SolutionThe motion of a particle along the x-axis is described by the following velocity function v(t)={:{((t^(3)+t^(2)+1),0 le t lt 3,"sec"),((2t+5),3 le t lt 5,"sec"),((t^(2)-6t),5 le t,"sec"):} where v is in m/sec and t is in second The chagne in acceleration of particle from t=3 sec to t=4 sec is
View SolutionThe population of a city increases at the rate 3% per year.
If at time t the population of city is p, then find equation of p in time t.
View Solution
