Two particle of mass m and 2m, connected by a massless rod, slide on the insider of a smooth circular ring of radius r, as shown in Fig. 3E.44 (a). If the assembly is released from rest when θ=0, determine
(a) the velocity of the particle when the rod passes the horizontal position,
(b) the maximum velocity vmax of the particles.

Step by step video & image solution for Two particle of mass m and 2m, connected by a massless rod, slide on the insider of a smooth circular ring of radius r, as shown in Fig. 3E.44 (a). If the assembly is released from rest when theta = 0, determine (a) the velocity of the particle when the rod passes the horizontal position, (b) the maximum velocity v_("max") of the particles. by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Topper's Solved these Questions
- WORK AND ENERGYBook:ANURAG MISHRAChapter:WORK AND ENERGYExercise:Level 1
Explore 53 Videos
- WORK AND ENERGYBook:ANURAG MISHRAChapter:WORK AND ENERGYExercise:Level 2
Explore 18 Videos
- WORK AND ENERGYBook:ANURAG MISHRAChapter:WORK AND ENERGYExercise:Matching types problems
Explore 14 Videos
- UNIT AND DIMENSIONSBook:ANURAG MISHRAChapter:UNIT AND DIMENSIONSExercise:Matching Type Problem
Explore 4 Videos
Similar Questions
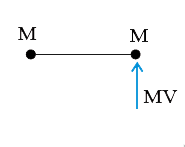
Two particles each of mass M are connected by a massless rod. The rod is lying on the smooth horizontal surface. If one of the particle is given an impulse MV as shown in the figure then angular velocity of the system would be:
View SolutionA block of mass M with a semi - circular track of radius R rests on a smooth floor. A sphere of mass m and radius r is released from rest from A. Find the velocity of sphere and track , when the sphere reaches B.

View Solution
Knowledge Check
Two beads of mass 2m and m, connected by a rod of length l and of negligible mass are free to move in a smooth vertical circular wire frame of radius l as shown. Initially the system is held in horizontal position ( Refer figure )
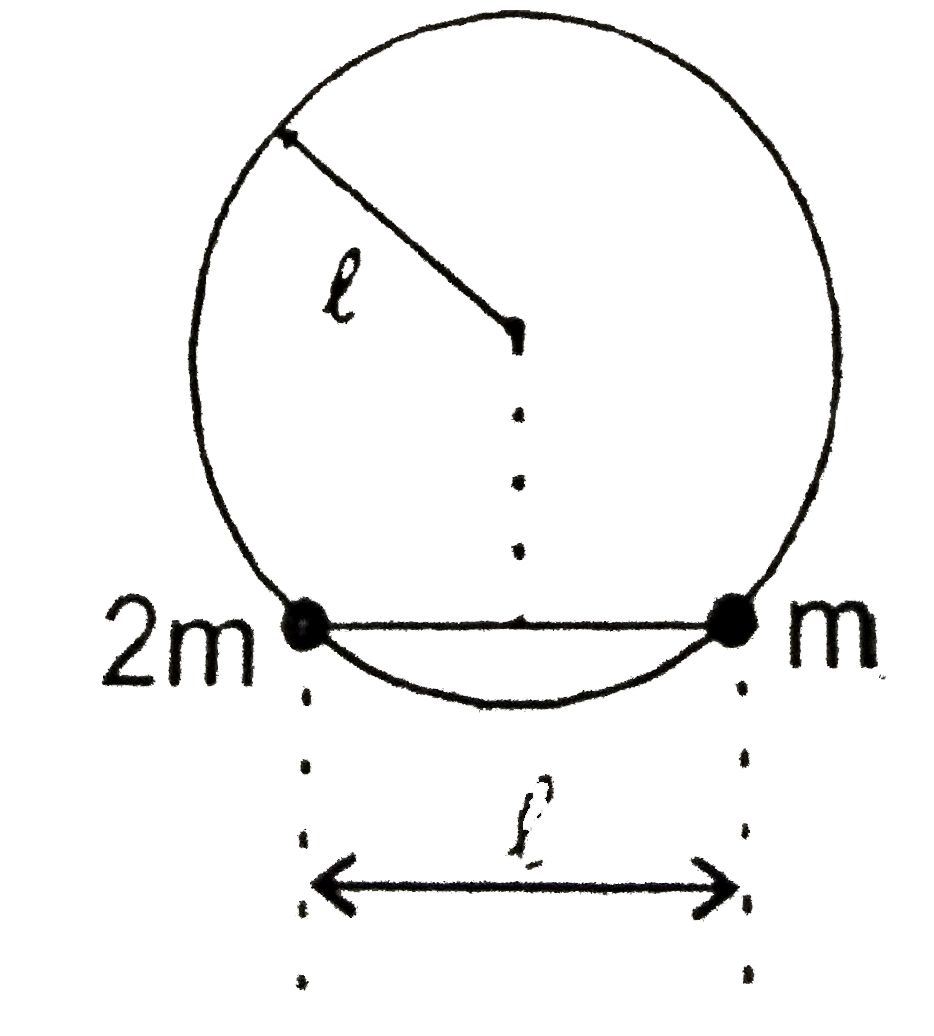
The velocity that should be given to mass 2m( when rod is in horizontal position ) in counter − clockwise direction so that the rod just becomes vertical is :A√5gl3B ⎷(3√3−13)glC√32glD√52glTwo particles A and B connected by a rigid rod AB. The rod slides along two perpendicular rails as shown. The velocity of A to the left is 10 m/s. What is the velocity of B, when angle θ is 37∘ ?
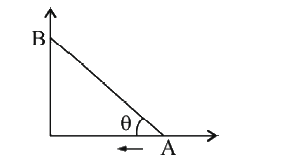 A5.8m/sB9.8m/sC11.2m/sD13.3m/s
A5.8m/sB9.8m/sC11.2m/sD13.3m/s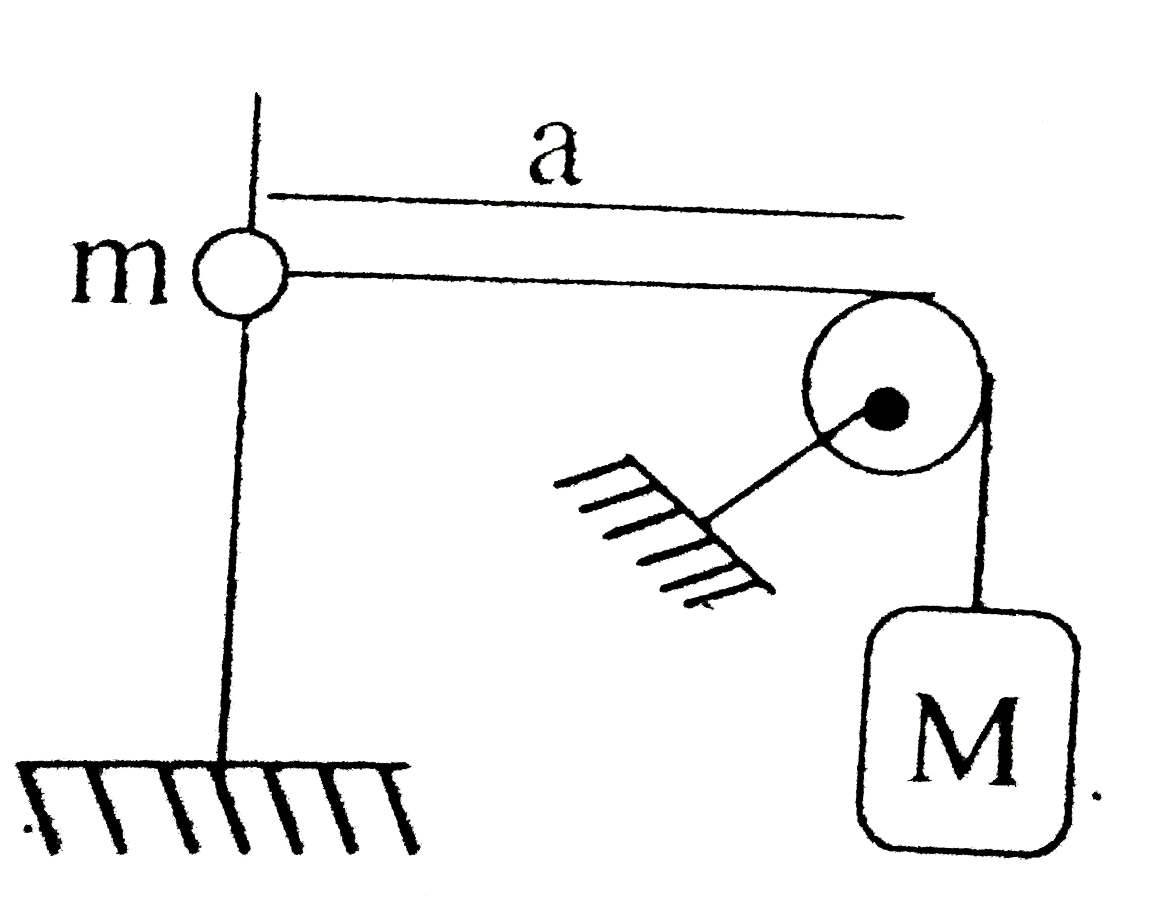
A ring of mass m can slide on the vertical smooth rod. Ring is connected by block with string as shown in figure. Pulley is of mass-less and smooth the system is released from reast. Initial tension in string isAT=MgBT>MgCT<MgDT=Mg+Mg
A point particle of mass m is released from a distance √3R along the axis of a fixed ring of mass M and radius R as shown in figure. Find the speed of particle as it passes through the centre of the ring.

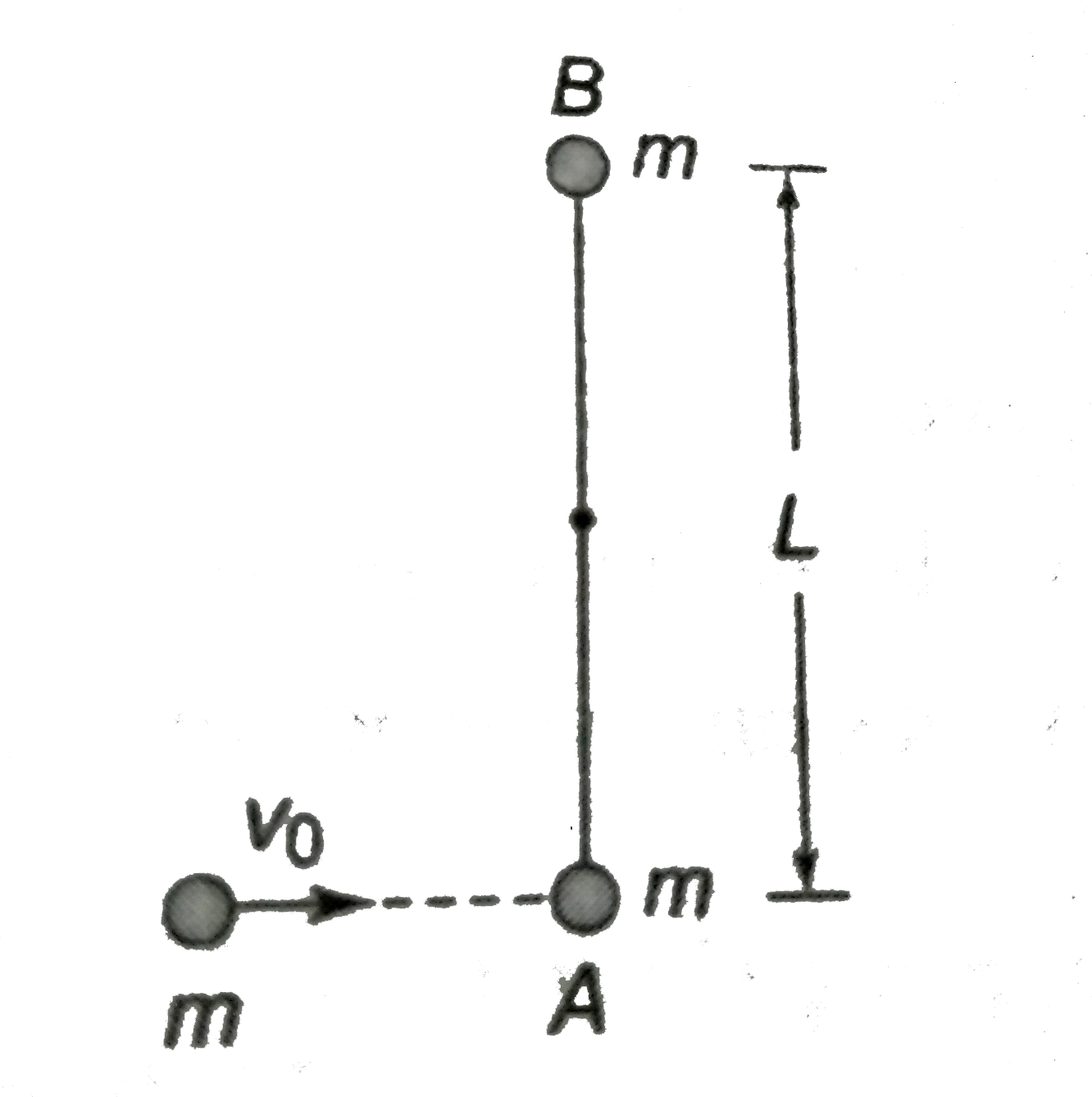
View SolutionTwo particles A and B, each of mass m, are connected by a light rod of length L lying on a smooth horizontal surface. A particle of mass m moving with speed v0 strikes to the end of rod and sticks to A as shown . Find
(a) the velocity of the center of mass,
(b) the angular velocity about the center of mass of system (A+B+ particle) and
(c) the linear speeds of A and B immediately after collision.
View SolutionA smooth sphere of radius R is moving in a straight line with an acceleration a. A particle is released from top of the sphere from rest. Find the speed of the particle when it is at an angular position θ from the initial position relative to the sphere.
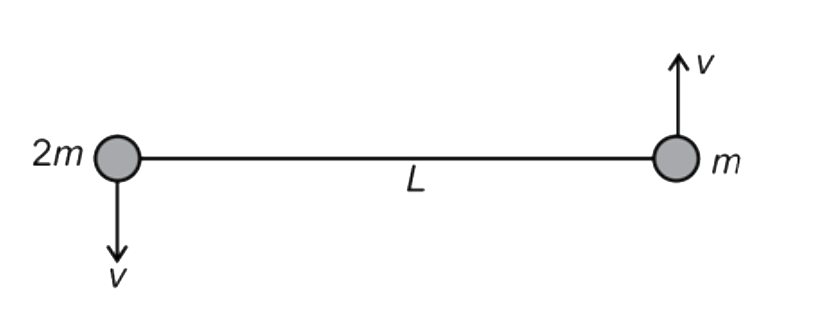
View SolutionTwo particles of mass m and 2m are connected by a string of length L and placed at rest over a smooth horizontal surface. The particles are then given velocities as indicated in the figure shown. The tension developed in the string will be
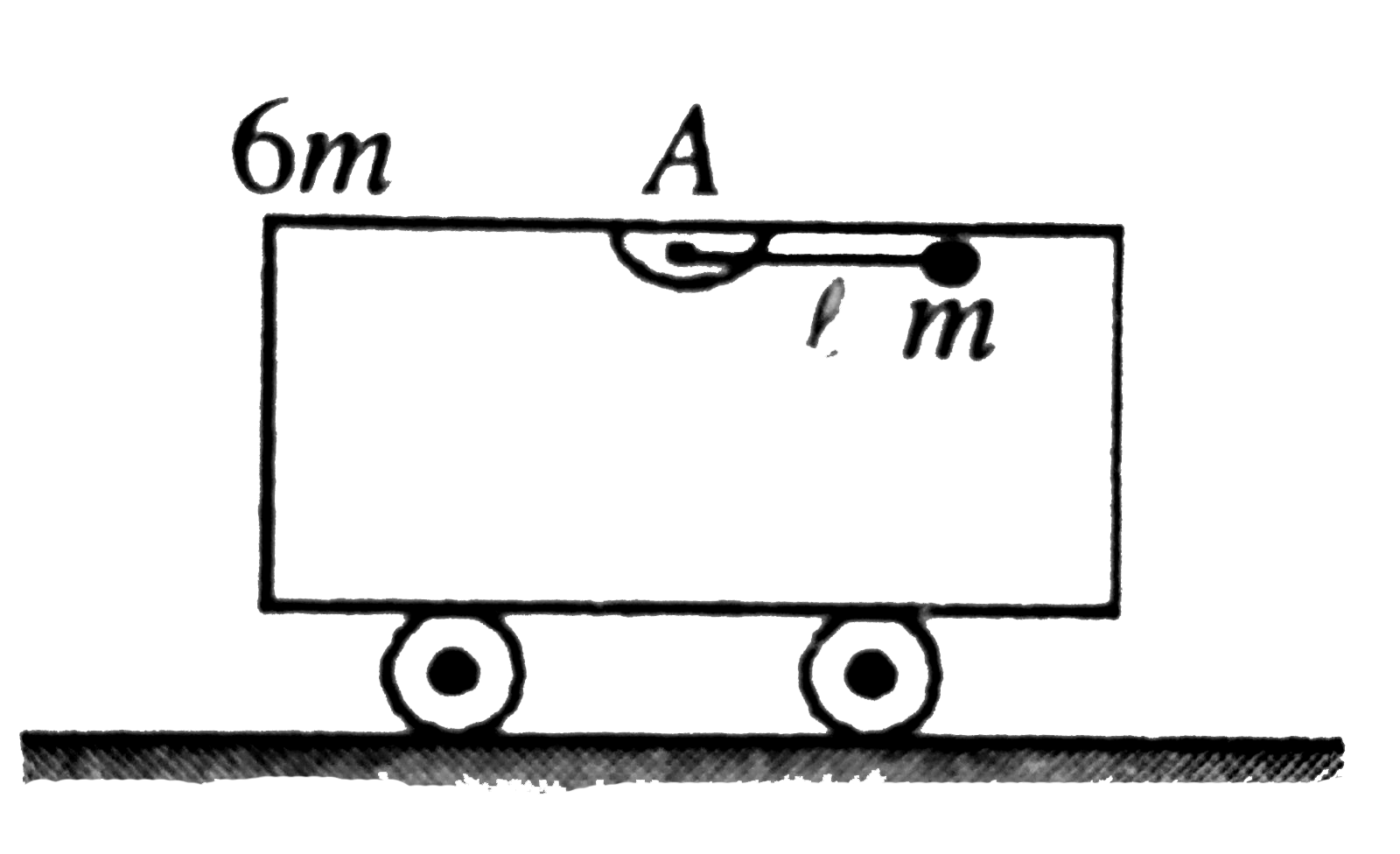
View SolutionIn the figure shown, the cart of mass 6m is initially at rest. A particle of mass in is attached to the end of the light rod which can rotate freely about A. If the rod is released from rest in a horizontal position shown, determine the velocity vrel of the particle with respect to the cart when the rod is vertical.
View Solution