A machine gun is mounted on the top of a tower of height h=100 m. At what angle should the gun be inclined to cover a maximum range of firing on the ground below ?The muzzle speed of the bullet is u=150ms−1 and g=10ms−2 .
The correct Answer is:α=12cos−1(1−u2u2+gh)=43∘47'
To solve the problem of determining the angle at which a machine gun should be inclined to cover a maximum range when fired from a height, we can follow these steps:
Step 1: Understand the Problem
We have a machine gun mounted on a tower of height h=100m. The muzzle speed of the bullet is u=150m/s, and the acceleration due to gravity is g=10m/s2. We need to find the angle α at which the gun should be inclined to maximize the horizontal range on the ground.
Step 2: Set Up the Equations
When the gun is fired at an angle α, the vertical and horizontal components of the initial velocity u are:
- ux=ucosα
- uy=usinα
The vertical displacement y of the bullet when it hits the ground is given by:
y=h−uyt−12gt2
Setting y=0 (when the bullet hits the ground), we have:
0=h−usinα⋅t−12gt2
Substituting the values:
0=100−150sinα⋅t−5t2
Step 3: Rearranging the Equation
Rearranging the equation gives us:
5t2+150sinα⋅t−100=0
This is a quadratic equation in t.
Step 4: Solve for Time t
Using the quadratic formula t=−b±√b2−4ac2a:
- Here, a=5, b=150sinα, and c=−100.
t=−150sinα±√(150sinα)2−4⋅5⋅(−100)2⋅5
t=−150sinα±√22500sin2α+200010
t=−15sinα±√22500sin2α+200010
Step 5: Calculate the Horizontal Range R
The horizontal range R is given by:
R=ux⋅t=(150cosα)⋅t
Substituting t:
R=150cosα(−15sinα+√22500sin2α+200010)
Step 6: Maximize the Range
To find the angle α that maximizes the range R, we differentiate R with respect to α and set the derivative to zero:
dRdα=0
This involves using the product rule and simplifying the resulting expression.
Step 7: Solve for α
After differentiating and simplifying, we can find the angle α that maximizes the range. The calculations yield:
α≈43.47∘
Conclusion
The machine gun should be inclined at an angle of approximately 43.47∘ to cover the maximum range on the ground below.
---
We have a machine gun mounted on a tower of height h=100m. The muzzle speed of the bullet is u=150m/s, and the acceleration due to gravity is g=10m/s2. We need to find the angle α at which the gun should be inclined to maximize the horizontal range on the ground.
When the gun is fired at an angle α, the vertical and horizontal components of the initial velocity u are:
- ux=ucosα
- uy=usinα
y=h−uyt−12gt2
Setting y=0 (when the bullet hits the ground), we have:
0=h−usinα⋅t−12gt2
Substituting the values:
0=100−150sinα⋅t−5t2
Rearranging the equation gives us:
5t2+150sinα⋅t−100=0
This is a quadratic equation in t.
Using the quadratic formula t=−b±√b2−4ac2a:
- Here, a=5, b=150sinα, and c=−100.
t=−150sinα±√(150sinα)2−4⋅5⋅(−100)2⋅5
t=−150sinα±√22500sin2α+200010
t=−15sinα±√22500sin2α+200010
The horizontal range R is given by:
R=ux⋅t=(150cosα)⋅t
Substituting t:
R=150cosα(−15sinα+√22500sin2α+200010)
To find the angle α that maximizes the range R, we differentiate R with respect to α and set the derivative to zero:
dRdα=0
This involves using the product rule and simplifying the resulting expression.
After differentiating and simplifying, we can find the angle α that maximizes the range. The calculations yield:
α≈43.47∘
The machine gun should be inclined at an angle of approximately 43.47∘ to cover the maximum range on the ground below.
Topper's Solved these Questions
Similar Questions
A machine gun is mounted on the top of a tower of height h. At what angle should the gun be inclined to cover a maximum range of firing on the ground below ? The muzzle speed of bullet is 150ms−1. Take g=10ms−2.
View SolutionA machine gun is mounted on the top of a tower 60m At what angle showld the gun be inclined to cver a vaximu range of firing on the groun below? The muzzle speed of the bullet is 90ms−1 take g=10ms1.
View Solution
Knowledge Check
- Question 1 - Select One
A machine gun fires a bullet of mass 65 g with a velocity of 1300 m/s. The main holding it can exert a maximum force of 169 N on the gun. The number of bullets he can fire per second will be
A1B2C3D4 - Question 2 - Select One
A gun of mass 20 kg has bullet of mass 0.1 kg in it. The gun is free to recoil and 804 J of recoil energy is released on firing the gun. The speed of bullet (in ms−1) is
A√804×2010B√2010804C√8042010D√804×4×103 - Question 3 - Select One
A machine gun fires a bullet of mass 40 gm with a speed 1200m/s The man holding it can exert a maximum force of 144 N on the gun. How many bullets can he fire per second at the most?
AOneBFourCTwoDThree
A stone is thrown from a cliff 4.2 m high . At what angle he must incline his rifle in order to cover a maximum range of firing on the ground below . Taken muzzle velocity of bullet =20m/s and g=10ms−2.
View SolutionA machine gun fires a bullet of mass 65 g with a velocity of 1300 m/s. The man holding it can exert a maximum force of 169 N on the gun. The number of bullets he can fire per second will be
View SolutionA machine gun fires 50-g bullets at a speed of 1000 m/s . The gunner , holding the machine in his hands , can exert an average force of 150 N against the gun . Calculate the maximum number of bullets he can fire one minute.
View SolutionA machine gun is mounted on an armored car moving with a speed of 20ms−1. The gun point against the direction of motion of car. The muzzle speed of bullet is equal to speed of sound in air i.e., 340ms−1. The time difference between bullet actually reaching and sound of firing reaching at a target 544m away from car at the instant of firing is reaching at a target 544m away from car at the instant of firing is
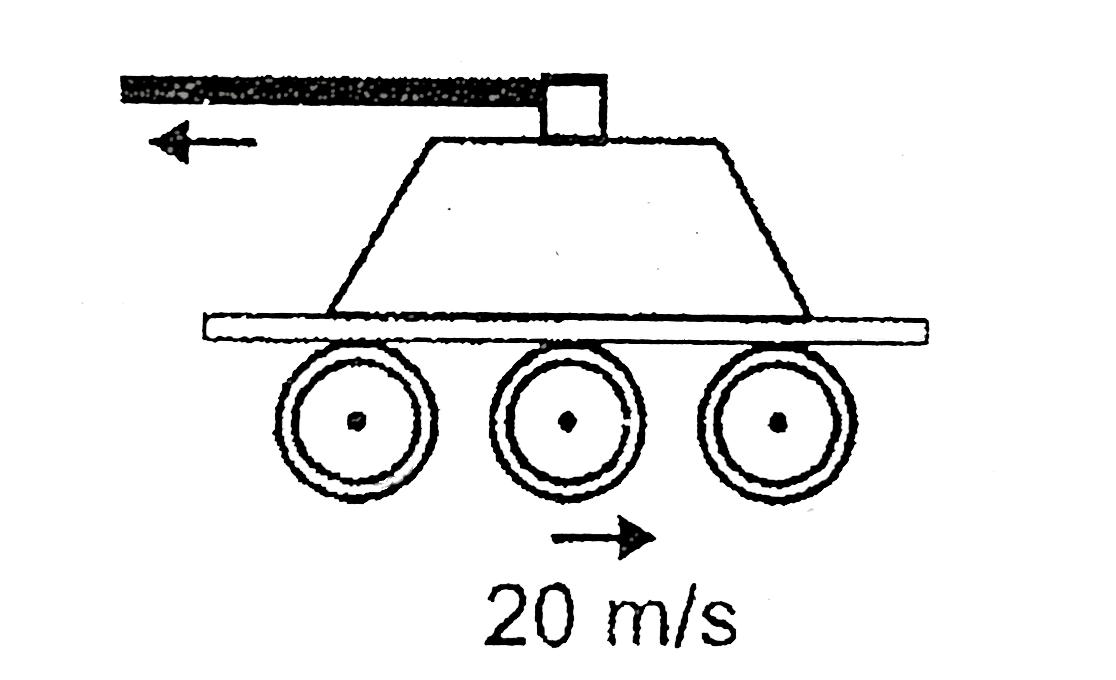 View Solution
View SolutionA bullet is fired from a gun eith a speed of 1000m/s on order to hit target 100 m away At what height above target should the gun be aimed ? (The resistance of air is negligible and g = 10 m/s2)
View Solution

