Three particles start from the origin at the same time, one with velocity u1 along the x-axis, the second with velocity u2 along the y-axis . Find the velocity of the third particles, along the x=y line so that the three particles may always lie on the same straight line.
The correct Answer is:v=√2.u1u2u1+u2
Step by step video, text & image solution for Three particles start from the origin at the same time, one with velocity u_1 along the x-axis, the second with velocity u_2 along the y-axis . Find the velocity of the third particles, along the x=y line so that the three particles may always lie on the same straight line. by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
A particle starts from the origin at time t = 0 and moves along the positive x-axis. The graph of velocity with respect to time is shown in figure. What is the position of the particle at time t = 5s ?

View SolutionA particle starts from the origin, goes along the X-axis to the pont (20m, 0) and then returns along the same line to the point (-20m,0). Find the distance and displacement of the particle during the trip.
View Solution
Knowledge Check
Three particles starts from origin at the same time with a velocity 2ms−1 along positive x-axis, the second with a velocity 6ms−1 along negative y-axis, Find the velocity of the third particle along x=y line so that the three particles may always lie in a straight line.
A−3√3B3√2C−3√2D2√2Three particles start from the origin at the same time, one with a velocity v1 along the x-axis, second along the negative y-axis with a velocity v2 and third particle moves along the line x = y. The velocity of third particle, so that three may always lie on the same line is:
Av1+v22B√v1+v2Cv1v2v1+v2Dv=√2v1v2v1−v2Three particles A,B and C start from the origin at the same time,A with a velocity a along x-axis, B with a velocity b along y-axis and C with velocity c in XY plane along the line x=y.The magnitude of c so that the three always remain collinear is:
Aa+b2B√abCaba+bD√2aba+b
A particle starts from the origin and moves along a stright line If the velocity of the particle at time t seconds 10t cm/s then find the distance traversed during fist seconds after the start.
View SolutionA particle starts from the origin,goes along the "X" - axis to the point(20m,0) and then returns along the same line to the point (-20m,0 then the displacement of the particle during the trip in negative direction is....(in m )
View SolutionThree particles start from origin at the same time: one with velocity v1 along positive x-axis, the second along the positive y-axis with a velocity v2 and the third along the line y = x with such a speed that all the three always stay in a straight line, then velocity of the third particles is:
View SolutionThe variation of velocity of a particle moving along a straight line is shown in . is
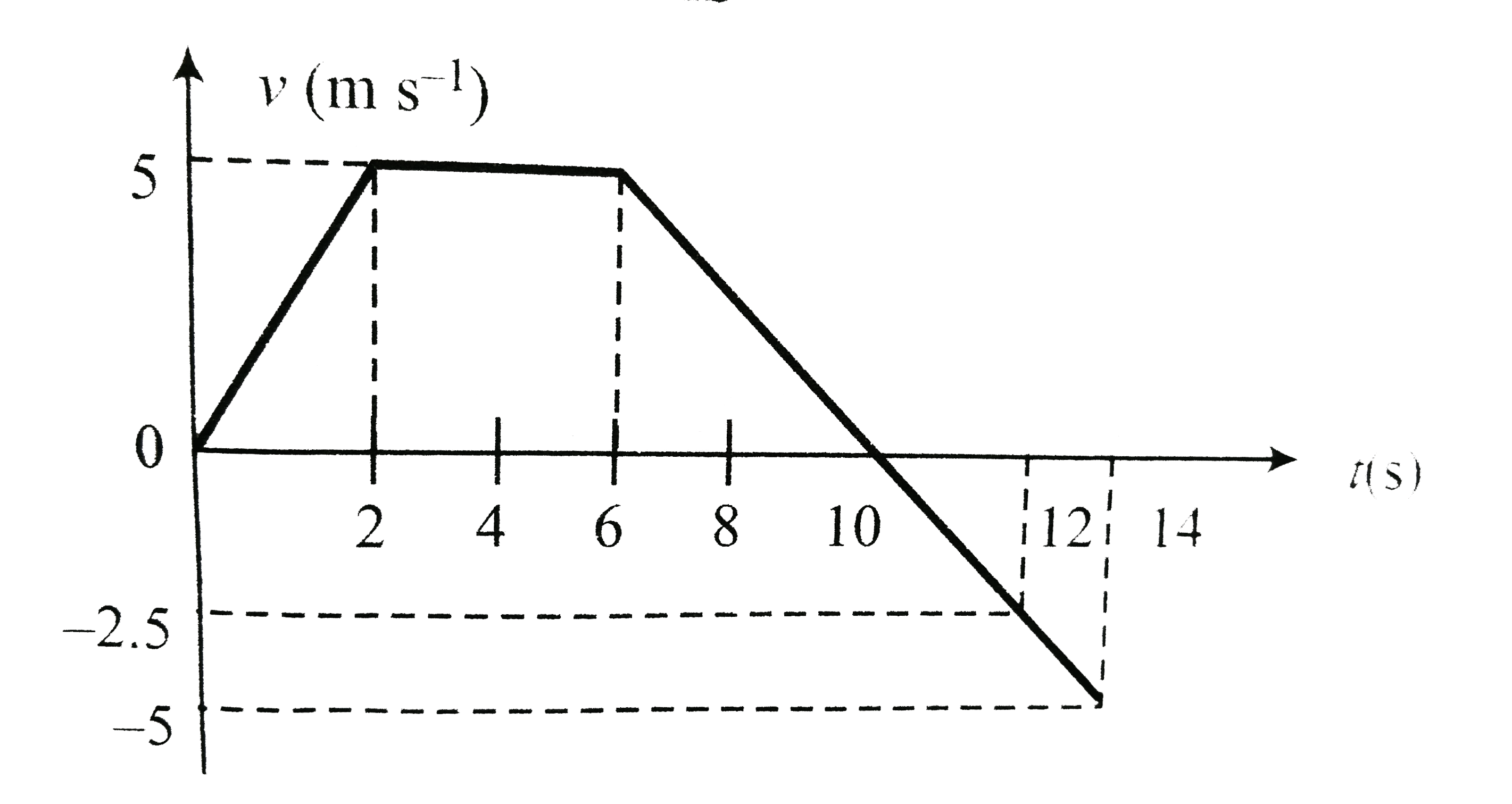 .
.
View SolutionTwo particles start moving from the same point along the same straight line. The first moves with constant velocity v and the second with constant acceleration a. During the time that elapses before the sound catches the first, the greatest distance between the particle is.
View Solution
