Suppose that the string of a simple pendulum is elastic and k is its force constant. Show that the string will stretch by an amount Δl=3mgk when Δl<<l. Also show that the speed of the bob at the bottom is v=√2g(l−3mg2k). The amplitude of the pendulum is 90∘.
Step by step video, text & image solution for Suppose that the string of a simple pendulum is elastic and k is its force constant. Show that the string will stretch by an amount Deltal=(3mg)/(k) when Delta l lt ltl. Also show that the speed of the bob at the bottom is v=sqrt(2g(l-(3mg)/(2k))). The amplitude of the pendulum is 90^(@). by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Topper's Solved these Questions
- CENTRIPITAL AND CENTRIFUGAL FORCESBOOK - NN GHOSHCHAPTER - CENTRIPITAL AND CENTRIFUGAL FORCESEXERCISE - Exercises (A)
18 Videos
- CALORIMETRYBOOK - NN GHOSHCHAPTER - CALORIMETRYEXERCISE - All Questions
28 Videos
- COMPOSITION AND RESOLUTION OF VELOCITIESBOOK - NN GHOSHCHAPTER - COMPOSITION AND RESOLUTION OF VELOCITIESEXERCISE - Exercise
25 Videos
Similar Questions
Define elastic potential energy. An elastic spring of force constant k is compressed by an amount x. Show that its pulenlial energy is 12kx2
View SolutionA simple pendulum of length L and mass m has a spring of force constant k connected to it at a distance h below its point of suspension. Find the frequency of vibrations of the system for small values of amplitude.
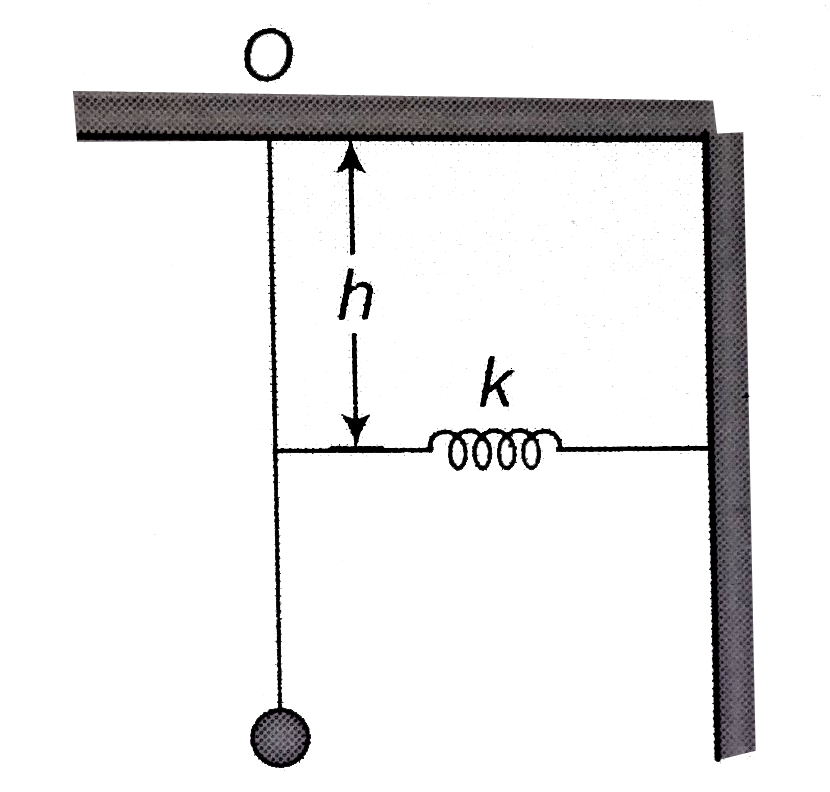
View Solution
Knowledge Check
At any instant of time, the total energy (E) of a simple pendulum is equal to the sum of its kinetic energy (12mv2) and potential energy (12kx2) where, m is the mass, v is the velocity, x is the displacement of the bob and k is a constant for the pendulum, the amplitude of oscillation of the pendulum is 10 cm and its total energy is 4 m J. Find k.
A1.8Nm−2B0.8Nm−2C0.8Nm−2DData insufficientA simple pendulum of length L swings in a vertical plane. The tension of the string when it makes an angle θ with the vertical and the bob of mass m moves with a speed v is (g is the gravitational acceleration)
Amv2/LBmgcosθ+mv2/LCmgcosθ−mv2/LDmgcosθAn elastic spring of unstretched length L and spring constant K is stretched by a small length x. It is further stretched by another small length y. the work done in second stretcing is
A1/2Ky2B1/2Ky(2x+y)C1/2K(x2+y2)D1/2k(x+y)2
The bob of a simple pendulum of length l is released from a point in the same horizontal line as the point of suspension and at a distance l from it Calculate the tension in the string at the lowest point of its swing
(b) If the string of the pendulum is catched by a nail located verticaly below the point of suspensions and the bob just swings around a complete circle around the nail, find the distance of the nail from the point of suspension
(c) If the string of the pendulum is made of rubber then show that it will be stretched by 3 mg/k on reaching the bob at the lowest point. Here k is the force constant of the string
View Solution(i) A simple pendulum consist of a small bob of mass m tied to a string of length L. Show that the total energy of oscillation of the pendulum is E≅12mgLθ20 when it is oscillating with a small angular amplitude θ0. Assume the gravitational potential energy to be zero of the lowest position of the bob. (ii) Three identical pendulums A, B and C are suspended from the ceiling of a room. They are swinging in semicircular arcs in vertical planes. The string of pendulum A snaps when it is vertical and it was found that the bob fell on the floor with speed V1. The string of B breaks when it makes an angle of 30° to the vertical and the bob hits the floor with speed V2. The string of pendulum C was cut when it was horizontal and the bob falls to the floor hitting it with a speed V3. Which is greatest and which is smallest among V1,V2 and V3?
View SolutionA simple pendulum is constructed by attaching a bob of mas m to a string of length L fixed at its upper end. The bob oscillates in a vertical circle. It is found that the speed of the bob is v when the string makes an angle θ with the vertical. Find the tension in the string at this instant.
View SolutionThe bob of a simple pendulum of length L, is displaced through 90∘, from its mean position and then released. What will be the tension in the string when the bob of mass m will be its lowest position ?
View SolutionThe bob of a simple pendulum of length L, is displaced through 90∘, from its mean position and then released. What will be the tension in the string when the bob of mass m will be at its lowest position?
View Solution
