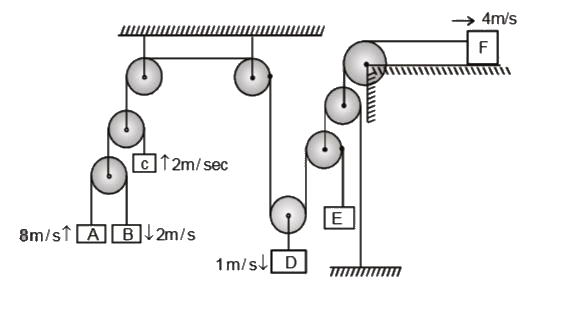
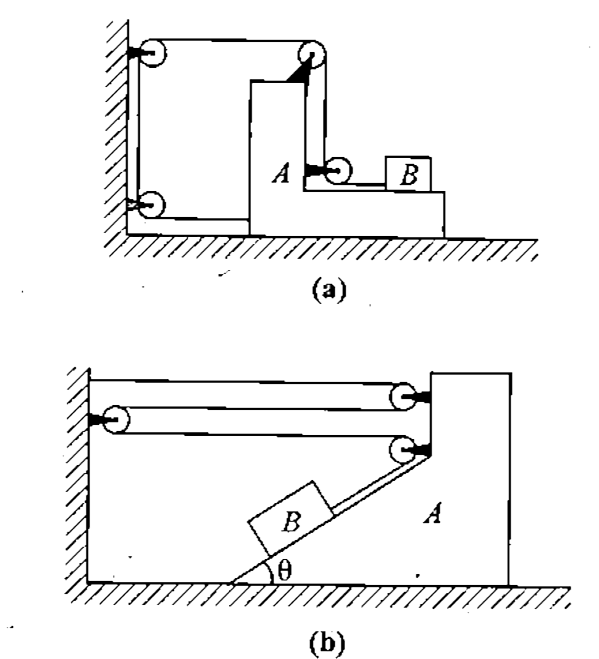
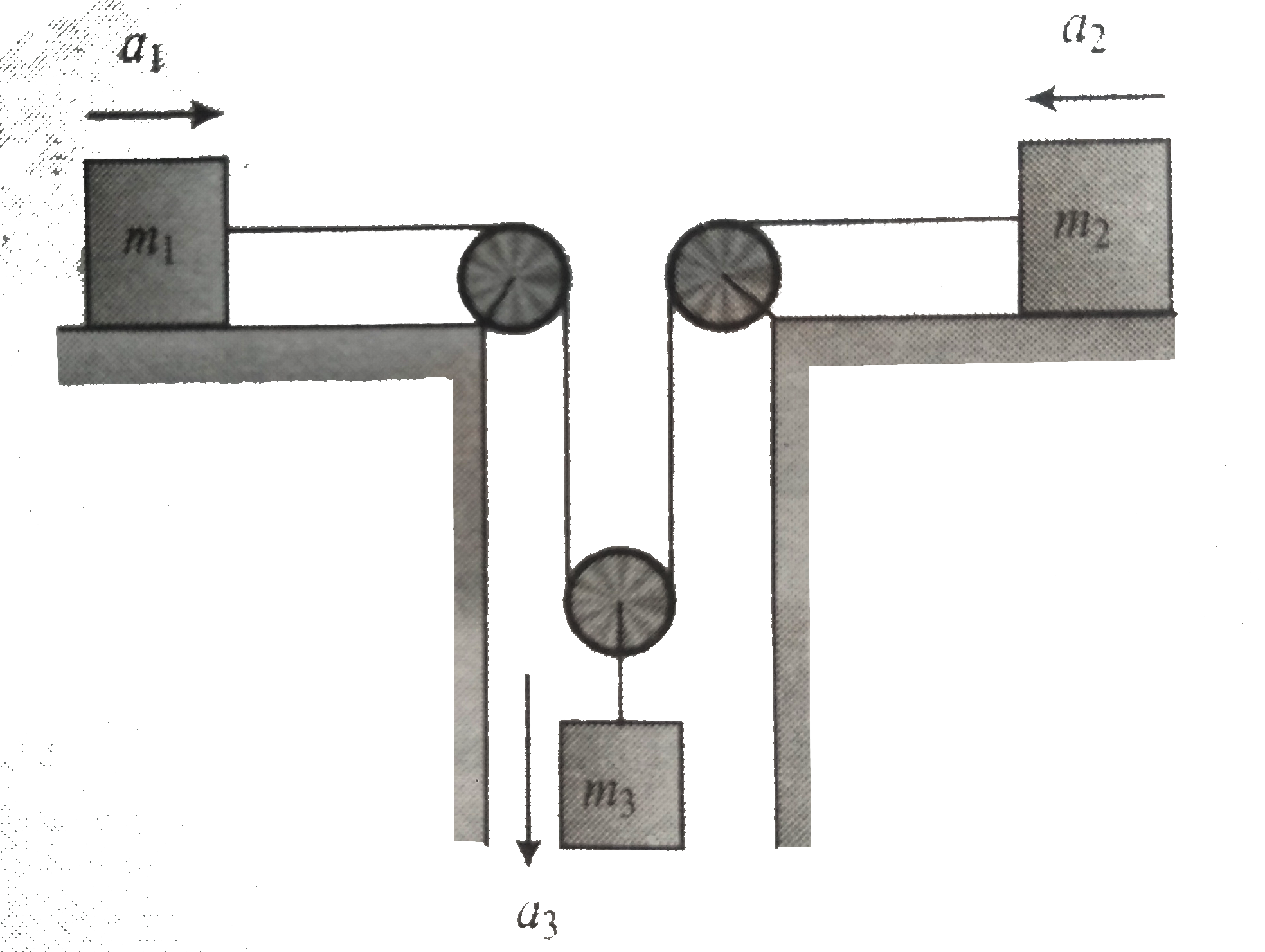
Find the Relation among velocities of the blocks shown in figure (a), (b) moving under the given constraints.
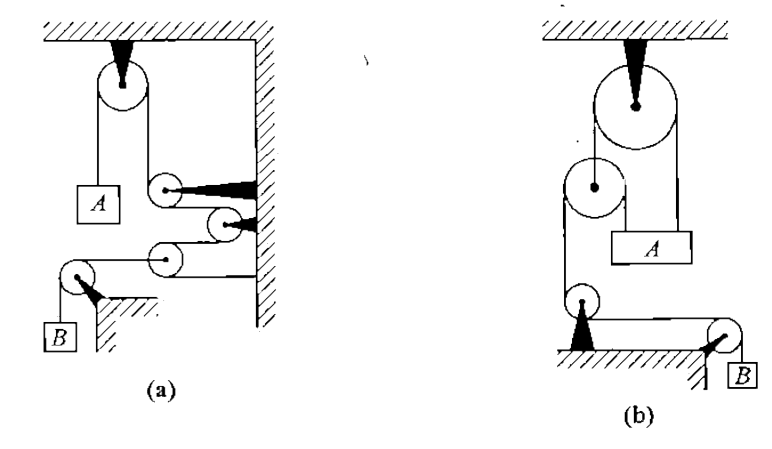
The correct Answer is:[(a) 2vB=vA(vB↑⏐;vA⏐↓) (b) 3vA=vB(vA↑⏐;vB⏐↓)]
Step by step video, text & image solution for Find the Relation among velocities of the blocks shown in figure (a), (b) moving under the given constraints. by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Topper's Solved these Questions
- BOOK - PHYSICS GALAXY - ASHISH ARORACHAPTER - KINEMATICSEXERCISE - Discussion Question18videos
KINEMATICS
- BOOK - PHYSICS GALAXY - ASHISH ARORACHAPTER - KINEMATICSEXERCISE - Conceptual MCQs Single Option Correct35videos
KINEMATICS
- BOOK - PHYSICS GALAXY - ASHISH ARORACHAPTER - KINEMATICSEXERCISE - Unsolved Numerical Pro.83videos
KINEMATICS
- BOOK - PHYSICS GALAXY - ASHISH ARORACHAPTER - GRAVITATIONEXERCISE - Unsolved Numerical94videos
GRAVITATION
- BOOK - PHYSICS GALAXY - ASHISH ARORACHAPTER - LINEAR MOMENTUM & ITS CONSERVATIONEXERCISE - exercise 1.33videos
LINEAR MOMENTUM & ITS CONSERVATION
Similar Questions
Knowledge Check
- Question 1 - Select One
Find the maximum value of force F such that the block shown in the figure does not move
 A20 NB10 NC12 ND15 N
A20 NB10 NC12 ND15 N
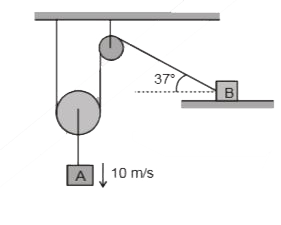
Find velocity of block 'B' at the instant shown in figure :
 View Solution
View SolutionFind the acceleration of the block B as shown in figure- 1.95 (a) and (b) relative to the block A and relative to ground if the block A is moving toward left with acceleration a.
 View Solution
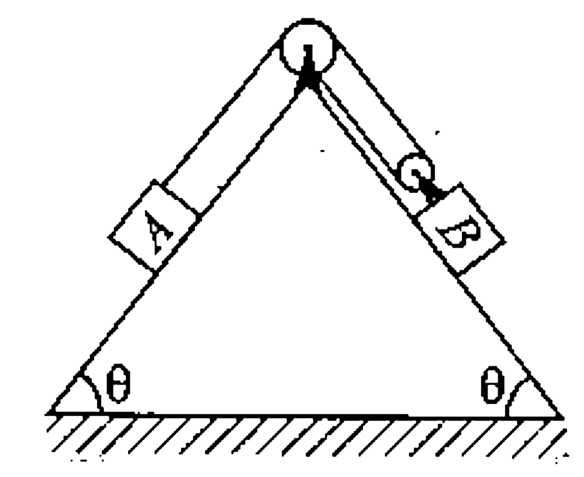
View SolutionFind the relation among the acceleration of blocks A and B constrained to move along the inclined surfaces of the fixed wedge shown in figure
 View Solution
View Solution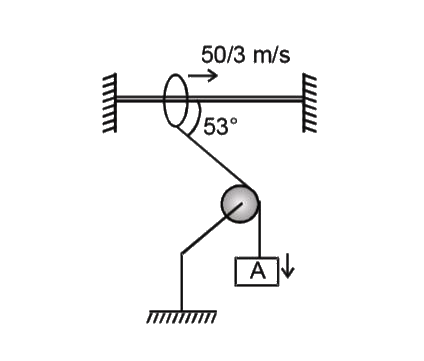
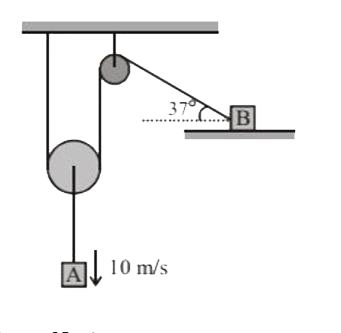
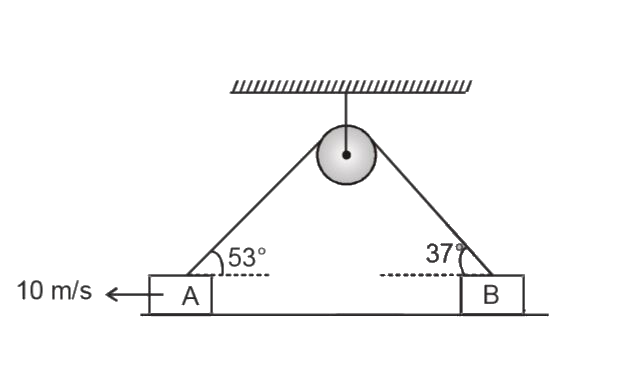
What is the velocity of block A in the figure as shown above.View SolutionFind out the velocity of block B in a pulley block system as shown in figure.
 View Solution
View SolutionFind the acceleration of the blocks A and B in the three situations shown in figure.
 View Solution
View SolutionIn the arrangement of three block as shown in fig. the string is inextensible. If the direction of accelerations are as shown in the figure, then determine the constraint relation.
 View Solution
View Solution