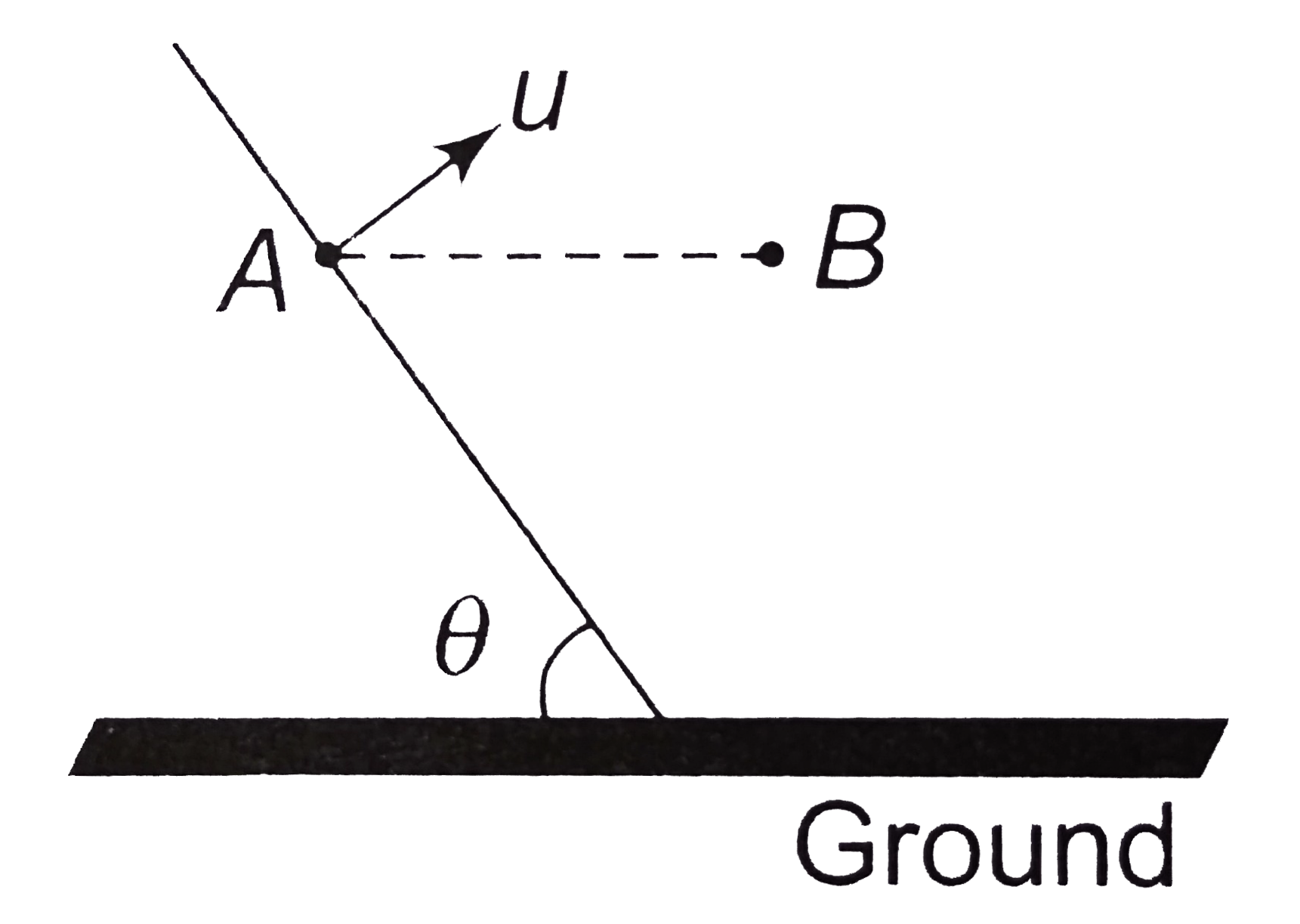
A particle is projected at point A from an inclination plane with inclination angle $θ$ as shown in figure. The magnitude of projection velocity is $\to u$ and its direction is perpendicular to the plane. After some time it passes from point B which is in the same horizontal level of A, with velocity $\to v$ . Then the angle between $\to u and \to v$ will be

Video Solution

Text Solution

Verified by Experts

The correct Answer is: $⎡ ⎢ ⎣ u = {[\frac{g R (1 + 3 {sin}^{2} β)}{2 sin β}]}^{1 / 2} ⎤ ⎥ ⎦$

Answer

Step by step video, text & image solution for A particle is projected at point A from an inclination plane with inclination angle theta as shown in figure. The magnitude of projection velocity is vecu and its direction is perpendicular to the plane. After some time it passes from point B which is in the same horizontal level of A, with velocity vecv. Then the angle between vecu and vecv will be by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.

Updated on:21/07/2023

Class 11 PHYSICS RECTILINEAR MOTION

Topper's Solved these Questions

Knowledge Check

Question 1 - Select One
A ball is projected horizontally from an inclined plane with a velocity V, as shown in the figure. It will strike the plane after a time

A $\frac{v_{0}}{\sqrt{3} g}$
B $\frac{2 v_{0}}{\sqrt{3} g}$
C $\frac{v_{0}}{g}$
D $\frac{2 \sqrt{3} v_{0}}{g}$
Question 2 - Select One
A particle is projected with a velocity u from the foot of an inclined plane whose inclination to the horizon is $β$ . It strikes. the plane at right angles if
A $tan (α - β) = cot β$
B $2 tan (α - β) = cot β$
C $2 cot (α - β) = cot β$
DNone of these
Question 3 - Select One
A particle is projected up an inclined plane with initial speed $v = 20 m / s$ at an angle $θ = 30^{\circ}$ with plane. The component of its velocity perpendicular to plane when it strikes the plane is
A $10 \sqrt{3} m / s$
B $10 m / s$
C $5 \sqrt{3} m / s$
DData is insufficient

PHYSICS GALAXY - ASHISH ARORA-KINEMATICS-Unsolved Numerical Pro.

Home
Ask Doubt
Profile

The correct Answer is:⎡⎢⎣u=[gR(1+3sin2β)2sinβ]1/2⎤⎥⎦

Topper's Solved these Questions

Similar Questions

Knowledge Check

A ball is projected horizontally from an inclined plane with a velocity V, as shown in the figure. It will strike the plane after a time

A particle is projected with a velocity u from the foot of an inclined plane whose inclination to the horizon is β. It strikes. the plane at right angles if

A particle is projected up an inclined plane with initial speed v=20m/s at an angle θ=30∘ with plane. The component of its velocity perpendicular to plane when it strikes the plane is

PHYSICS GALAXY - ASHISH ARORA-KINEMATICS-Unsolved Numerical Pro.

The correct Answer is: $⎡ ⎢ ⎣ u = {[\frac{g R (1 + 3 {sin}^{2} β)}{2 sin β}]}^{1 / 2} ⎤ ⎥ ⎦$

A particle is projected with a velocity u from the foot of an inclined plane whose inclination to the horizon is $β$ . It strikes. the plane at right angles if

A particle is projected up an inclined plane with initial speed $v = 20 m / s$ at an angle $θ = 30^{\circ}$ with plane. The component of its velocity perpendicular to plane when it strikes the plane is