A quadrilateral has vertices (4,1),(1,7),(−6,0) AND (−1,−9). Show that mid-points of the sides of this quadrilateral form a parallelogram.
Mid point of PR=⎛⎜
⎜⎝(52)+(−72)2,⎛⎜
⎜⎝4+(−92)2⎞⎟
⎟⎠
=(−12,−14)
MId point of SQ=⎛⎜
⎜⎝(32)−(52)2⎞⎟
⎟⎠,⎛⎜
⎜⎝−4+(72)2⎞⎟
⎟⎠
=(−12,−14)
PQ=√52+(12)2=√25+14=√1014
SR=√52+(12)2=√25+14+√1014
RQ=√(−72+52)2+(−92−72)2=√65
SP=√(32−52)2+(−8)2=√65
PQ=SR
RQ=SP.
Similar Questions
If the mid-points of the sides of a quadrilateral are joined in order, prove that the area of the parallelogram, so formed will be half of the area of the given quadrilateral (figure).
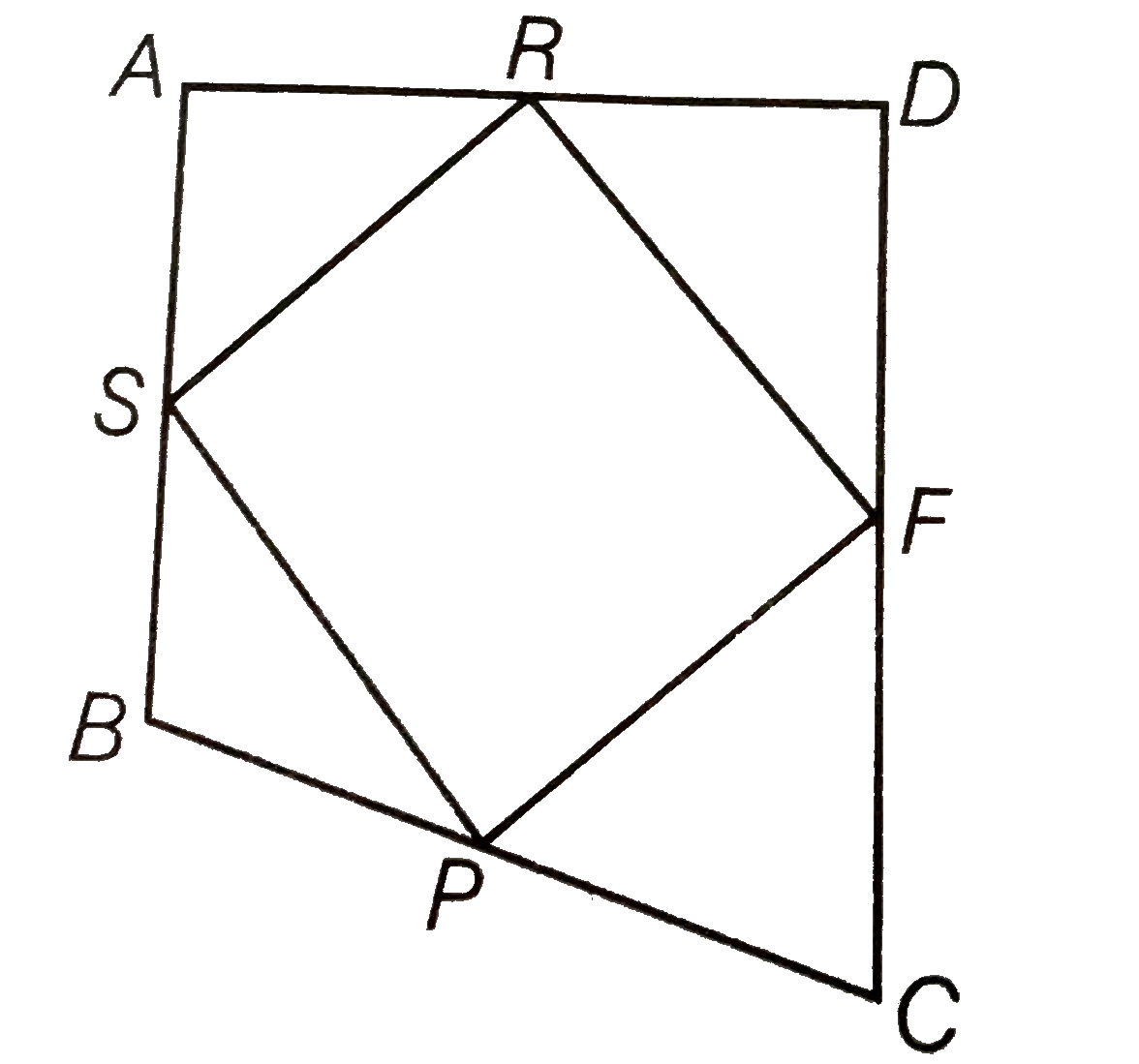
View SolutionA quadrilateral has the vertices at the points (−4,2), (2,6), (8,5) and (9,−7) . Show that the mid points of the sides of this quadrilateral are the vertices of a parallelogram.
View SolutionA quadrilateral has vertices (4,1),(1,7),(−6,0) AND (−1,−9). Show that mid-points of the sides of this quadrilateral form a parallelogram.
View SolutionIf the mid-points of the sides of a quadrilateral are joined in sequence, then prove that the area of the parallelogram so formed is half of the area of the given quadrilateral.
View Solutionसिद्ध कीजिए कि किसी चतुर्भुज कि क्रमागत भुजाओं के मध्य-बिंदुओं को मिलाने से बना चतुर्भुज समान्तर चतुर्भुज होता है।
View SolutionProve that the quadrilateral formed by joining the mid-points of the pairs of consecutive sides of a quadrilateral is a parallelogram.
View SolutionProve that the quadrilatral formed by joining the mid points of the sides of a quadrilateral is a parallelogram.
View Solution(−2,4),(4,8),(10,7) and (11,−5) are the vertices of a quadrilateral. Show that the quadrilateral, obtained on joining the mid-points of its sides, is a parallelogram.
View Solution
