The force exerted by a spring when it's displaced by x from its natural length is given by the equation F(x)=−kx, where k is a positive constant. What is the work done by a spring as it pushes out from x=−x2 to x=−x1 (where x2>x1)?
We sketch the graph of F(x)=-kx and calculate the area under the graph from x=−x2 to x=−x1.

Here, the region is a trapezoid with area A=12(base1+base2)×height, so
W=A=12(kx2+kx1)(x2−x1)
=12k(x2+x1)(x2−x1)
=12k(x22−x21).
Topper's Solved these Questions
Similar Questions
Knowledge Check
The spring force is given by F=−kx, here k is a constant and x is the deformation of spring. The F−x graph is
A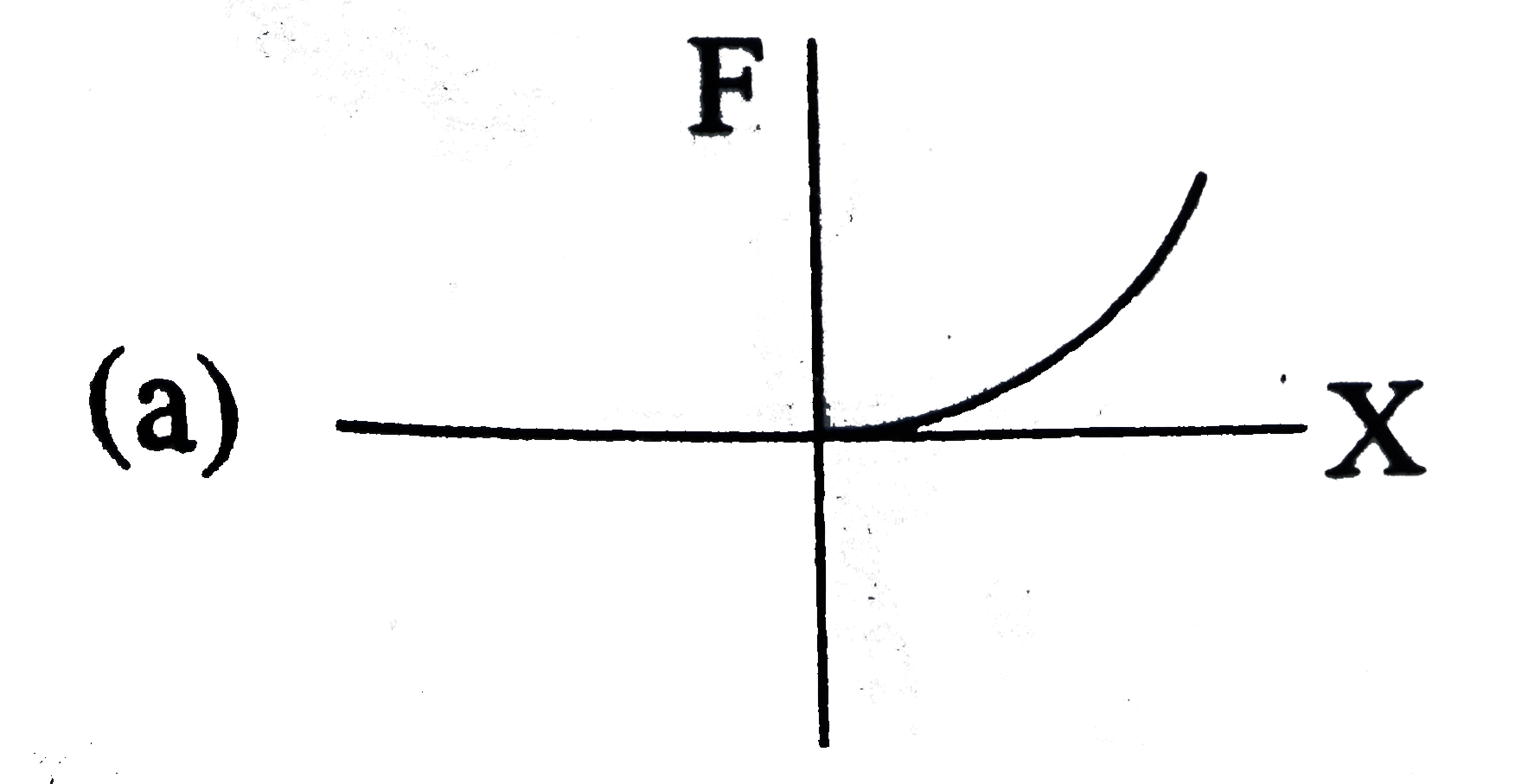 B
B C
C D
D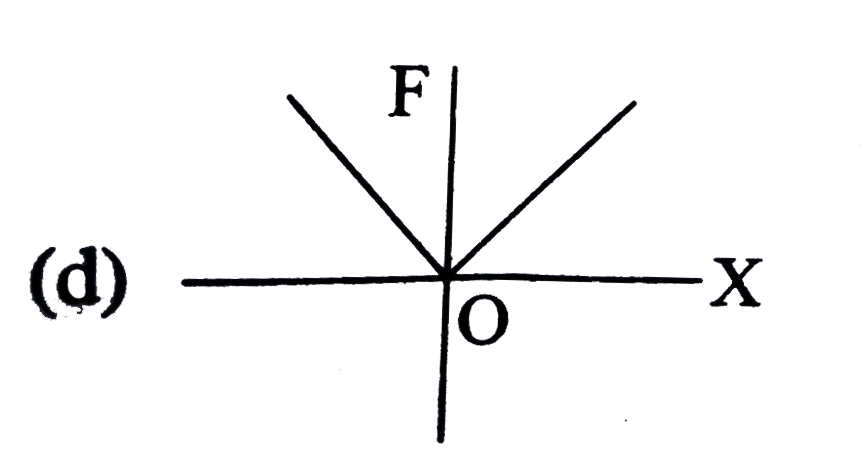
The force acting on a bod moving parallel to the X-axis is F=3x+2x2. What is the work done in displacing the body from x=1 to x=2?
A656 unitsB256 unitsC556 unitsD356 units
A force F=a+b x acts on a particle in the X-direction, where a and b are constants. Find the work done by this force during a displacement from x=0 to x=d/
View SolutionIn the above question, find the work done by the spring force as the bloc k is moved leftward from x1=15mm to xz=−10mm
View SolutionA force F=(a+bx) acts on a particle in x direction where a and b are constants . Find the work done by this force during displacement from x1 to x2 .
View SolutionA force F=a+bx acts on a particle in x-direction, where a and b are constants. Find the work done by this force during the displacement from x1 to x2.
View SolutionA force F=a+bx acts on a particle in x-direction, where a and b are constants. Find the work done by this force during the displacement from x1 to x2.
View SolutionA particle is moving along the x-axis and force acting on it is given by F=F0sinωxN, where ω is a constant. The work done by the force from x=0 to x=2 will be
View Solution
