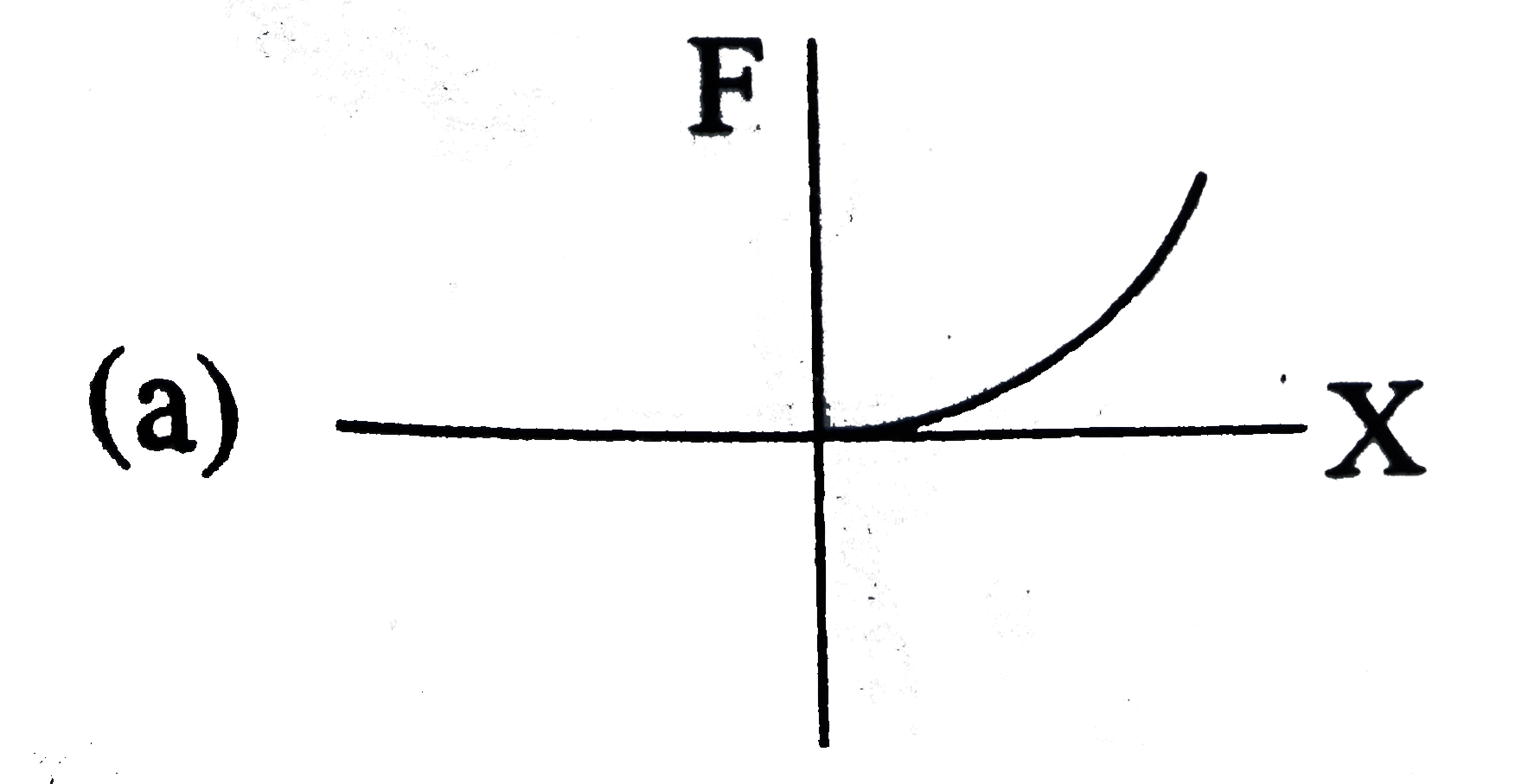
The spring force is given by F=−kx, here k is a constant and x is the deformation of spring. The F−x graph is


The correct Answer is:C
To analyze the spring force given by the equation F=−kx, we will derive the characteristics of the F versus x graph step by step.
Step 1: Understand the Equation
The equation F=−kx indicates that the force F exerted by the spring is directly proportional to the displacement x from its equilibrium position, with k being the spring constant. The negative sign indicates that the force exerted by the spring is in the opposite direction to the displacement.
Step 2: Identify the Variables
In this equation:
- F is the force exerted by the spring.
- x is the displacement or deformation of the spring from its equilibrium position.
- k is a positive constant known as the spring constant.
Step 3: Determine the Type of Graph
The equation F=−kx can be compared to the linear equation y=mx, where:
- y corresponds to F,
- x corresponds to x,
- m (the slope) corresponds to −k.
Step 4: Analyze the Slope
Since k is a positive constant, the slope of the graph F versus x is negative (−k). This means that as x increases, F decreases, indicating a linear relationship with a negative slope.
Step 5: Identify the Intercept
The equation F=−kx passes through the origin (0,0) because when x=0, F also equals 0. Therefore, the graph intercepts the origin.
Step 6: Sketch the Graph
The graph of F versus x will be a straight line that:
- Starts at the origin (0,0),
- Has a negative slope,
- Extends downward as x increases.
Conclusion
The F−x graph is a straight line passing through the origin with a negative slope.
The equation F=−kx indicates that the force F exerted by the spring is directly proportional to the displacement x from its equilibrium position, with k being the spring constant. The negative sign indicates that the force exerted by the spring is in the opposite direction to the displacement.
In this equation:
- F is the force exerted by the spring.
- x is the displacement or deformation of the spring from its equilibrium position.
- k is a positive constant known as the spring constant.
The equation F=−kx can be compared to the linear equation y=mx, where:
- y corresponds to F,
- x corresponds to x,
- m (the slope) corresponds to −k.
Since k is a positive constant, the slope of the graph F versus x is negative (−k). This means that as x increases, F decreases, indicating a linear relationship with a negative slope.
The equation F=−kx passes through the origin (0,0) because when x=0, F also equals 0. Therefore, the graph intercepts the origin.
The graph of F versus x will be a straight line that:
- Starts at the origin (0,0),
- Has a negative slope,
- Extends downward as x increases.
The F−x graph is a straight line passing through the origin with a negative slope.
Topper's Solved these Questions
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - CENGAGE PHYSICS DPPEXERCISE - subjective type51videos
CENGAGE PHYSICS DPP
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - CENGAGE PHYSICS DPPEXERCISE - Multiple correct Answer Type54videos
CENGAGE PHYSICS DPP
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - CAPACITOR AND CAPACITANCEEXERCISE - Integer5videos
CAPACITOR AND CAPACITANCE
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - COULOMB LAW AND ELECTRIC FIELDEXERCISE - Single Answer Correct Type22videos
COULOMB LAW AND ELECTRIC FIELD
Similar Questions
The force exerted by a spring when it's displaced by x from its natural length is given by the equation F(x)=−kx, where k is a positive constant. What is the work done by a spring as it pushes out from x=−x2 to x=−x1 (where x2>x1)?
View SolutionIn SHM restoring force is F=−kx, where k is force constant, x is displacement and a is amplitude of motion, then total energy depends upon
View Solution
Knowledge Check
- Question 1 - Select One
f(x)=3x3+kx2−32x+28
The function f is defined by the equation above where k is a nonzero constant. In the xy-plane the graph of f intersects the x-axis at three points: (−2,0),(32,0),and(c,0). What is the value of k?A−25B−17C7D14
A particle of mass m initially at rest. A variabl force acts on the particle f=kx2 where k is a constant and x is the displacment. Find the work done by the force f when the speed of particles is v.
View SolutionTwo identical particles each of mass m are connected by a light spring of stiffness K. The initial deformation of the spring when the system was at rest, x0. After releasing the system, if one of the particles has a speed v and the deformation of the spring is x, find the speed of the other particle neglecting friction.
View SolutionFigure a) shows a spring of force constant k clamped rigidly at once end and a mass m attached to its free end. A force F applied at the free end stretches the spring. Figure b) shows the same spring with both ends free and attached to a mass m at either end. Each end of the spring in figure is stretched by the same force F.
(a) What is the maximum extension of the spring in the two cases ?
(b) If the mass in figure and the two masses in figure are released free, what is the period of oscillation in each case?
 View Solution
View SolutionFigure a) shows a spring of force constant k clamped rigidly at once end and a mass m attached to its free end. A force F applied at the free end stretches the spring. Figure b) shows the same spring with both ends free and attached to a mass m at either end. Each end of the spring in figure is stretched by the same force F.
(a) What is the maximum extension of the spring in the two cases ?
(b) If the mass in figure and the two masses in figure are released free, what is the period of oscillation in each case?
 View Solution
View SolutionA spring of force constant k extends by a length X on loading . If T is the tension in the spring then the energy stored in the spring is
View Solution(a) What is elastic potential energy of a spring ? Obtain the relation for elastic P.E.U=12kx2. Where k is force constant of spring, x is extension or compression produced in the spring.
View SolutionA block of mass m rests on a rough horizontal plane having coefficient of kinetic friction μk and coefficient of static friction μs. The spring is in its natural, when a constant force of magnitude P=5μkmg4 starts acting on the block. The spring force F is a function of extension x as F=kx2.( Where k is spring constant )
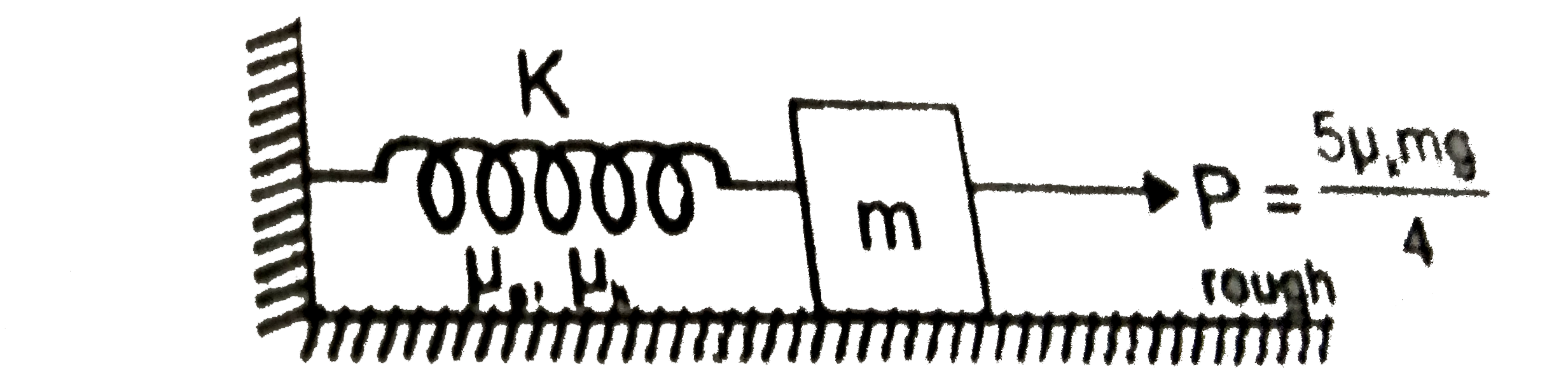
(a) Comment on the relation between μs and μk for the motion to start.
(b) Find the maximum extension in the spring ( Assume the force P is sufficient make the block move ).View Solution

