A circuit disc of mass 49 kg and of radius 50 cm is mounted axially and made to rotate. Calculate the K.E.. It possesses, when executing 120 rpm
The correct Answer is:484 J
To calculate the kinetic energy of a rotating disc, we can follow these steps:
Step 1: Convert the rotational speed from RPM to radians per second.
The formula to convert revolutions per minute (RPM) to radians per second is:
ω=2π×RPM60
Given that the disc rotates at 120 RPM:
ω=2π×12060=4πrad/s
Step 2: Calculate the moment of inertia (I) of the disc.
The moment of inertia for a solid disc rotating about its axis is given by:
I=12MR2
Where:
- M=49kg (mass of the disc)
- R=0.5m (radius of the disc, converted from cm to m)
Calculating I:
I=12×49×(0.5)2=12×49×0.25=498kg m2
Step 3: Calculate the kinetic energy (K.E.) of the rotating disc.
The kinetic energy of a rotating object is given by:
K.E.=12Iω2
Substituting the values of I and ω:
K.E.=12×498×(4π)2
Calculating ω2:
(4π)2=16π2
Now substituting back into the K.E. formula:
K.E.=12×498×16π2
K.E.=49×16π216=49π21=49π2J
Step 4: Calculate the numerical value of K.E.
Using π≈3.14:
K.E.≈49×(3.14)2≈49×9.8596≈483.1J
Final Answer:
The kinetic energy of the rotating disc is approximately 484 J.
---
The formula to convert revolutions per minute (RPM) to radians per second is:
ω=2π×RPM60
Given that the disc rotates at 120 RPM:
ω=2π×12060=4πrad/s
The moment of inertia for a solid disc rotating about its axis is given by:
I=12MR2
Where:
- M=49kg (mass of the disc)
- R=0.5m (radius of the disc, converted from cm to m)
I=12×49×(0.5)2=12×49×0.25=498kg m2
The kinetic energy of a rotating object is given by:
K.E.=12Iω2
Substituting the values of I and ω:
K.E.=12×498×(4π)2
Calculating ω2:
(4π)2=16π2
Now substituting back into the K.E. formula:
K.E.=12×498×16π2
K.E.=49×16π216=49π21=49π2J
Using π≈3.14:
K.E.≈49×(3.14)2≈49×9.8596≈483.1J
The kinetic energy of the rotating disc is approximately 484 J.
Topper's Solved these Questions
- ROTATIONAL MOTIONBOOK - SL ARORACHAPTER - ROTATIONAL MOTIONEXERCISE - Problem
24 Videos
- PROPERTIES OF SOLIDSBOOK - SL ARORACHAPTER - PROPERTIES OF SOLIDSEXERCISE - All Questions
97 Videos
- System of particles & rotational MotionBOOK - SL ARORACHAPTER - System of particles & rotational MotionEXERCISE - EXERCISE
353 Videos
Similar Questions
A disc of mass 5 kg and radius 50 cm rolls on the ground at the rate of 10ms−1. Calculate the K.E. of the disc.
View SolutionA sphere of mass 2 kg and radius 5 cm is rotating at the rate of 300 rpm. Calculate the torque required to stop it in 6.28 it in 6.28 revolution. Moment of inertia of the sphere about any diameter =25MR2
View Solution
Knowledge Check
A fly wheel of mass 60 kg and radius 40 cm is revolving at 300 rpm, then its rotational K.E. is
A48π2JB480πJC48πJD240π2JA circular disc of mass 4kg and of radius 10cm is rotating about its natural axis at the rate of 5rad/sec. Its angular momentum is
A0.25kgm2s−1B0.1kgm2s−1C2.5kgm2s−1D0.2kgm2s−1A disc of mass 2 kg and radius 0.2 m is rotating about an axis passing through its centre and perpendicular to its plane with an angular velocity 50 rad/s . Another disc of mass 4 kg and radius 0.1m rotates about an axis passing through its centre and perpendicular to its plane with angular velocity 100 rad/s. If the two disc are coaxially coupled, then the angular velocity of coupled system would be
A150 rad/sB120 rad/sC100/3 rad/sD200/3 rad/s
A sphere of mass 2kg and radius 5cm is rotating at the rate of 300 revolutions per minute. Calculate the torque required to stop it in 6.28 revolutions. [Moment of inertia of sphere about diameter =2/5mass×(radius)2].
View SolutionCalculate the KE of rotation of a circular disc of mass 1kg and radius 0.2m rotating about an axis passing through its centre and perpendicular to its plane. The disc is making 30/π rotations per minute.
View SolutionA solid cylinder, of mass 2 kg and radius 0.1 m, rolls down an inclined plane of height 3m. Calculate its rotational energy when it reaches the foot of the plane.
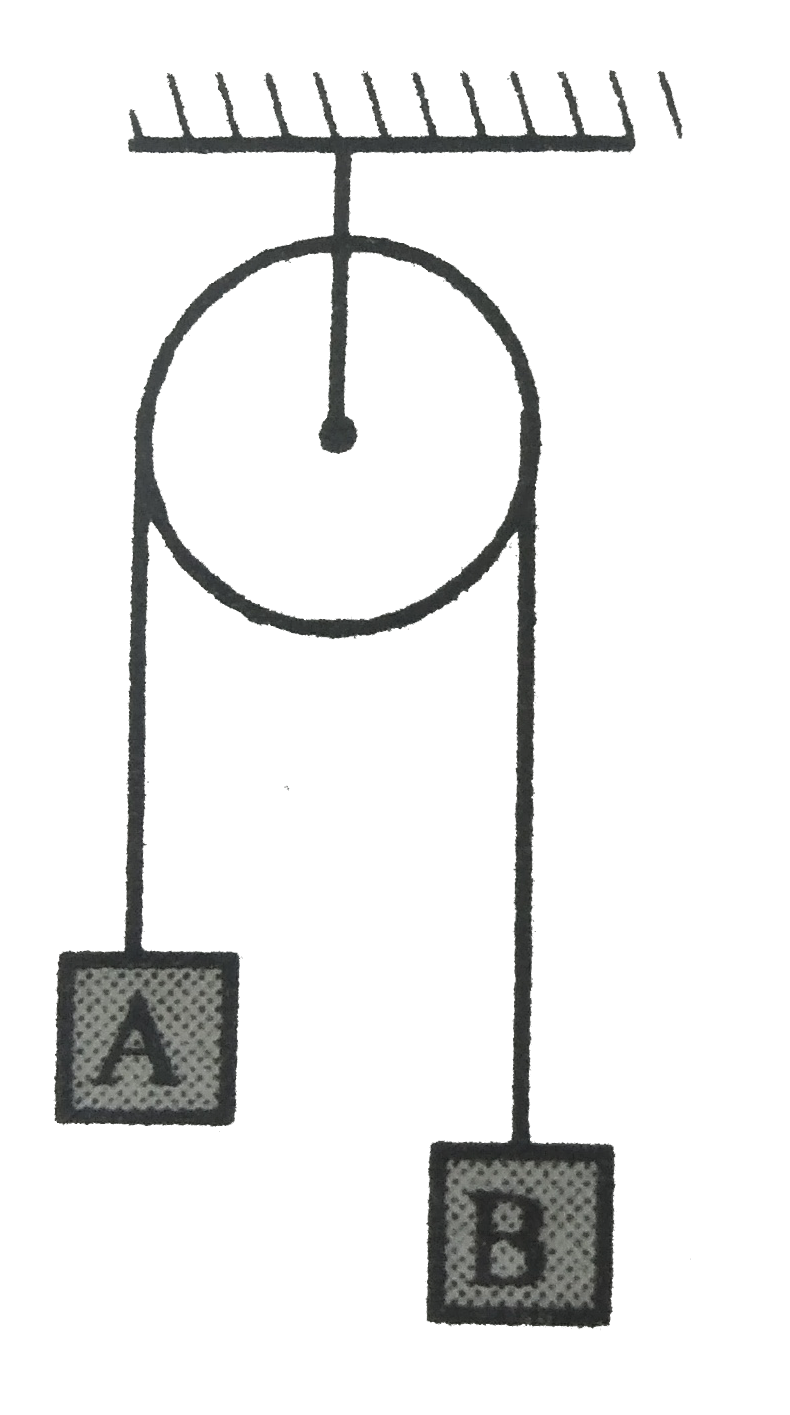
View SolutionIn the figure A and B are two blocks of mass 4kg and 2kg respectively attached to the two ends of light string passing overa disc C of mass 40kg and radius 0.1m. The disc is free to rotate about a fixed horizontal the string does not string does not slip over the disc. Find.
(i) The linear acceleration of mass B.
(ii) the number of revolutions made by the disc at the end of 10sec. from the start.
(iii) the tension in teh string segment supporting the block A.
 .
.
View SolutionA solid disc of mass 1kg and radius 10cm is rotating at the rate of 240 rpm.The torque required to stop it in 4π revolutions is?
View Solution