If an element can exist in several oxidation states, it is convenient to display the reduction potentials corresponding to the various half reactions in diagrammatic from, known as Latimer diagram. The Latimer diagram for chlorine in acid solution is
In basic solution is :
. The standard potentials for two nonadjacent species can also be calculated by using the concept that is an additive property but using potential is not an assitive property and If a given oxidation state is a the next higher oxidation state disproportionation can occur. The reverse of relative stabilities of the oxidation state can also be understood by drawing a graph of against oxidation state, known as Frost diagram, Choosing the stability of zero oxidation state arbitrery as zero. The most stable oxidation state of a apecies lies lowest in the digram Disproportionation is spontaneous if the species lies above a straight line joining its two product species.
Which of the following couple have same value of potential at pH = 0 and pH = 14?
The correct Answer is:D
Topper's Solved these Questions
- GRAPHICAL INTERPRETATIONBOOK - GRB PUBLICATIONCHAPTER - GRAPHICAL INTERPRETATIONEXERCISE - Comprehension 10
1 Videos
- GRAPHICAL INTERPRETATIONBOOK - GRB PUBLICATIONCHAPTER - GRAPHICAL INTERPRETATIONEXERCISE - Comprehension 11
1 Videos
- GRAPHICAL INTERPRETATIONBOOK - GRB PUBLICATIONCHAPTER - GRAPHICAL INTERPRETATIONEXERCISE - Comprehension 8
1 Videos
- F-BLOCK ELEMENTSBOOK - GRB PUBLICATIONCHAPTER - F-BLOCK ELEMENTSEXERCISE - Subjective type
7 Videos
- HYDROCARBON (ALIPHATIC)BOOK - GRB PUBLICATIONCHAPTER - HYDROCARBON (ALIPHATIC)EXERCISE - Previous years jee questions
28 Videos
Similar Questions
The principle on conductometric titration is based in the fact that during the titration, one of the ions is replaced by the other and invariably thses two ions differ in the ionic conductivity with the result that the conductivity of the solution varies during the course of the titration.Take,for example,the titration between strong acid, say HCl. and a strong base, say NaOH.Before NaOH is added the conuctance of HCl solution has a high value due ti the persence if highly hydrogen ions.As NaOH is added,H+ ions are the replaced by relatively slower moving Na+ ions.Consequently, the conductance of the solution decrease and this continues right upto the enuivalence point where the solution contains only NaCL.Beyond the equivalence point if more of NaOH is added, then the solution contains an excess of the fast moving OH− with the result that its as more of NaoH added, we plot the conductance value vs the amount of NaOH added we get curve of the type shown in figure.The descending portion AB represents the conductance before the equivalence point (solution cintains a mixture if acid HCl and the salt NaCL and the ascending portion CD represents the conductances after the equivalence point (solution contains the salt NaCL and the excess of NaOH). The point E which repersents only the minimum conductance is alkali and thus repersents the equivalence point.This point can, however, be obtained by the extrapoltion of the lines AB and CD and therefore one is not very particular in location this point experimentally as it is in the case of ordinary acid -base involving the acid base indicators

LET us take the specific exaanple of acetic acid being titration against NaOH.Before the addition the of alkali, the solution shows poor conductance due to feeble inization of acetic acid.Initially the addtion of alkali causes not only the replacement of H+ by Na+ but also suppresses the thus the conductance of the solution decrease in the begnning .But very soon the conductance starts increasing as addetion NaOH thus causing neutralizes the undissociated HAc to HAc with strong condutance eletroyte Na+Ac−.The increase in conducting OH− ions gtthe graph near the highly equivalence point actual equivalence point can as usual be ontained by the extrapoiation method
In all thses graphs it been assumed that the vloume change due addition of solution from burette in negligible heane vloume change of the sloution in beaker the conuctance of which is measured is almost constant throughout the measurement
the nature of curve obtained for the titration between weak acid vs strong base as described in the above will be
View SolutionTitration are one of the methods we can use to discover the precise concentration of solutions. A typical titration involves adding a solution from a burette to another solution in a flask. The end point of the titration is found by watching a colour change taking place.However, a problem arises when a suitable indicator cannot be found, or when the colour change involved are unclear. In these cases, redox potential may sometimes come to the rescue.
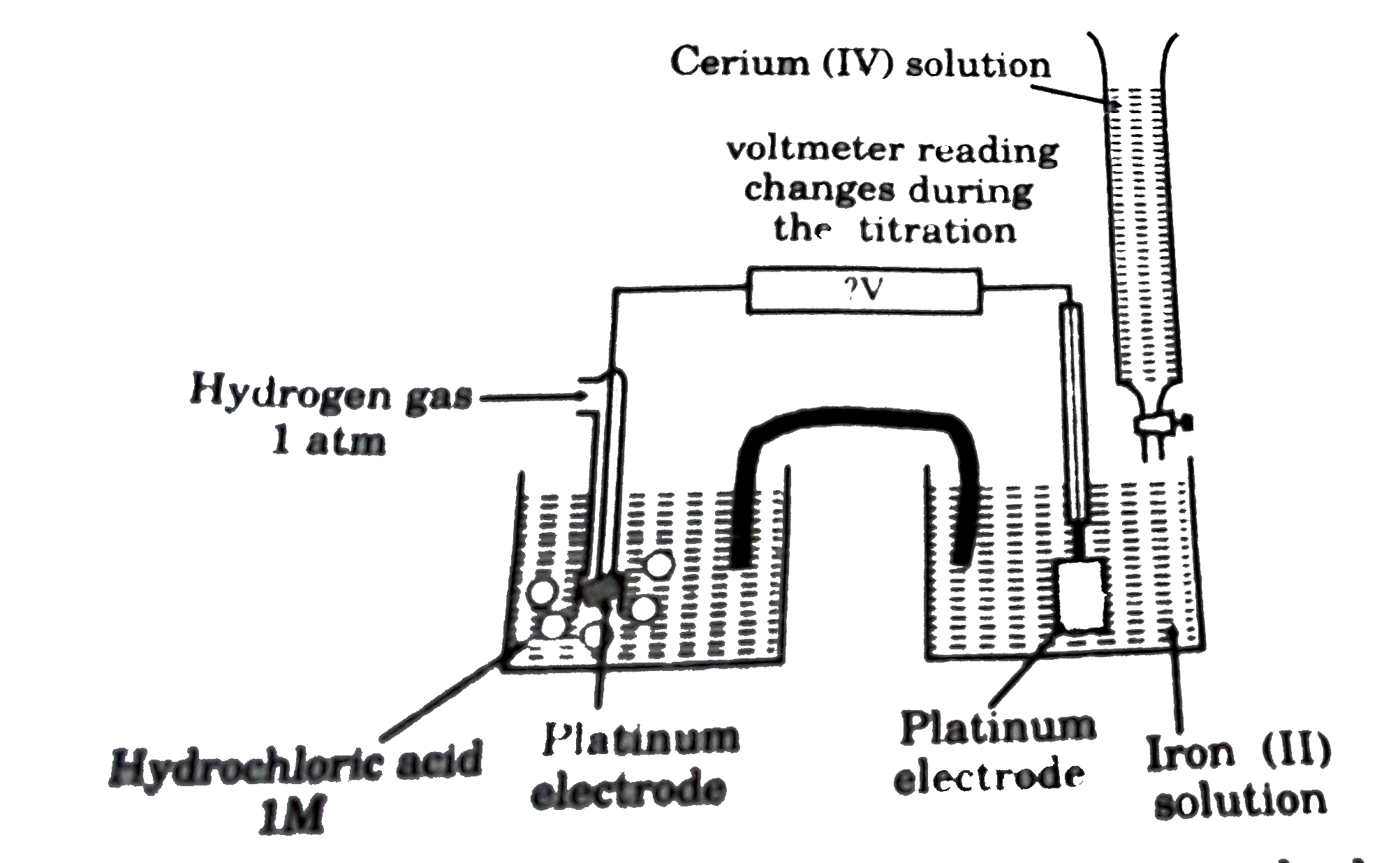
A perticularly well known example (Fig.) is a method of discovering then concentration of iron (II) ions in a solution by titrating them with a solution of cerium (IV). The redox potential that are of interest here areE∘Fe3+∣Fe2+=0.77VandE∘Ce4+∣Ce3+=1.61V. These tell us that cerium (IV) ions are the oxidising agents. They should react according to the equation
Fe2+(aq)+Ce4+(aq)→Fe3+(aq)+Ce3+(aq).
Now imagine that we know the concentration of the cerium (IV) ions solution in the burette. We want to measure the concentration of the cerium (IV) solution from the burette, some of the iron (II) ions are will be oxidised. As a consequence the beaker would now contain large number of unreacted iron (II) ions , but also some iron (III) ions as well. All of the cerium (III). The solution in the beaker now represents an iron (III)/iron(II) half cell although not at standard conditions.Thus , the emf of the cell will be near, but not equal to E∘Fe3+∣Fe2+.
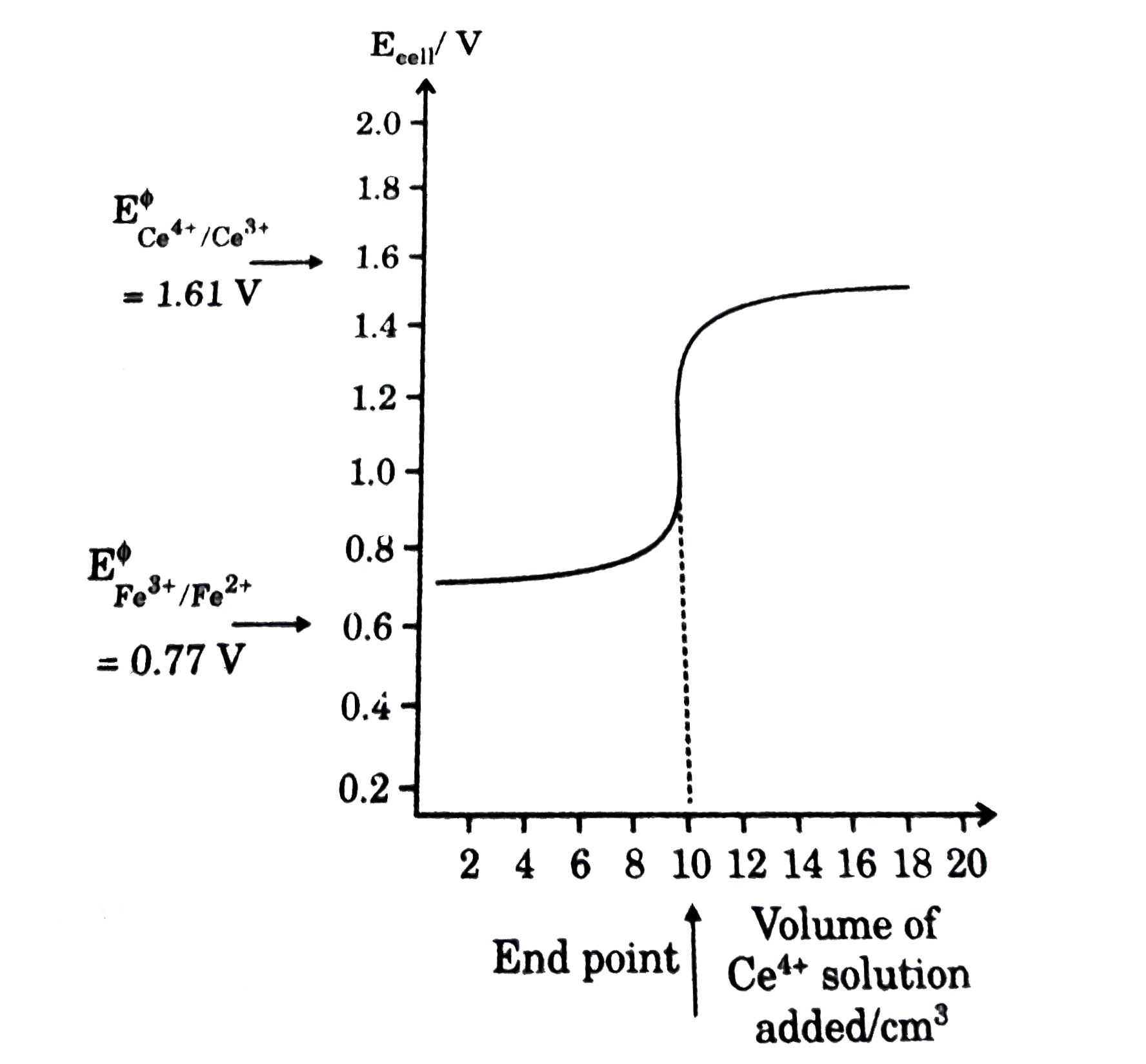
If we continue to add cerium (IV) solution , the number of iron (II) ions is gradually reduced and eventually only a very few are left (Table).At this stage the next few drops of cerium (IV) solution convert all the remaining irom (II) ions into iron (III), and some of the cerium (IV) ions are left unreacted. Once this happens we no longer have an iron (III)/iron(II) half-cell. Instead we have a solution in which there is a large number of cerium (III) ions and a smaller number of cerium (IV) ions. The solution in the beaker now behaves as a cerium (IV)/cerium(III)half-cell (although not a standard one.)
Just before all the iron (II) ions are converted into iron (III) we have a cell with an EMF of around +0.77V. After all the iron (II) ions are oxidised we have a cell with an emf of about +1.61V. This rapid rise in emf occurs with the addition of just one drop of cerium (IV) solution. You should be able to understand why a graph of cell emf against volume of cerium (IV) solution of the iron (II) solution calculated in the usual way.
When an ion is converted into a complex ion, the redox potential change. you can see this in the combination of standard emf of the iron (III)/iron(II) system (+0.36V). The cyanide ion is said to stabilize the oxidation state of the iron. If you were to make up a cell
Pt(s)∣∣ ∣∣Fe(CN)3−61mol dm−1(aq),Fe(CN)4−6∣∣ ∣∣∣∣ ∣∣Fe3+(aq)1mol dm−3,Fe2+(aq)∣∣ ∣∣Pt(s)
what would be the emf and what would be the cell reaction?
View SolutionThe variation of rate constant with temperature can be explained by Arrhenius equation. However , the can be explained by Activation energy is found to be temperature dependent/In such cases dinKdT=EaRT2 should be used to predict variation of rate constant with tempreature. On the basic of this information , answer the question that follow :
If Arrhenius constant and Ea (activation energy0 are assumed to be constant then which of the following graph will be correct
View SolutionThe first f-block organometallic compound [ThH(OR)(C5Me5)2] was prepared in late 1970s. Find the oxidation state of central metal atom/cation.
View SolutionWhich of the following will decolourise alkaline KMnO4?
View SolutionLatimer diagram for Cu in an acidic solution is:
Cu+2+0.15V−−−−→Cu++0.50−−−→Cu,
View SolutionStandard reduction potential (volts) in acid solution is given by the Latimer diagram as shown: CiO_(4)^(-)overset(1.19)rarrCIO_(3)^(-)overset(1.21)rarrCIOrarrCl_(2)rarrCl^(-) What is standard reduction potential of CIO_(3)^(-)rarr(1)/(2)Cl_(2) In acidic medium is:
View SolutionIf an element can exist in several oxidation states, it is convenient to display the reduction potentials corresponding to the various half reactions in diagrammatic from, known as Latimer diagram. The Latimer diagram for chlorine in acid solution is
Cl−4+1.20V−−−−→ClO−3+1.60V−−−−→ClO−2+1.60V−−−−→
ClO−+1.67V−−−−→Cl2+1.36V−−−−→Cl−
In basic solution is :
ClO−4+0.37V−−−−→ClO−3+0.30V−−−−→ClO−2+0.68−−−→
ClO−+0.42V−−−−→Cl2+1.36−−−→Cl−. The standard potentials for two nonadjacent species can also be calculated by using the concept that ΔG∘ is an additive property but using potential is not an assitive property and ΔG∘=−nFx0, If a given oxidation state is a the next higher oxidation state disproportionation can occur. The reverse of relative stabilities of the oxidation state can also be understood by drawing a graph of ΔG∘/F against oxidation state, known as Frost diagram, Choosing the stability of zero oxidation state arbitrery as zero. The most stable oxidation state of a apecies lies lowest in the digram Disproportionation is spontaneous if the species lies above a straight line joining its two product species.
What is the potential of couple ClO−Cl− at pH = 14 14?
View SolutionIf an element can exist in several oxidation states, it is convenient to display the reduction potentials corresponding to the various half reactions in diagrammatic from, known as Latimer diagram. The Latimer diagram for chlorine in acid solution is Cl_(4)^(-)overset(+1.20V)toClO_(3)^(-)overset(+1.60V)toClO_(2)^(-)overset(+1.60V)to ClO^(-)overset(+1.67V)toCl_(2)overset(+1.36V)toCl^(-) In basic solution is : ClO_(4)^(-)overset(+0.37V)toClO_(3)^(-)overset(+0.30V)toClO_(2)^(-)overset(+0.68)to ClO^(-)overset(+0.42V)toCl_(2)overset(+1.36)toCl^(-) . The standard potentials for two nonadjacent species can also be calculated by using the concept that DeltaG^(@) is an additive property but using potential is not an assitive property and DeltaG^(@)=-nFx^(0), If a given oxidation state is a the next higher oxidation state disproportionation can occur. The reverse of relative stabilities of the oxidation state can also be understood by drawing a graph of DeltaG^(@)//F against oxidation state, known as Frost diagram, Choosing the stability of zero oxidation state arbitrery as zero. The most stable oxidation state of a apecies lies lowest in the digram Disproportionation is spontaneous if the species lies above a straight line joining its two product species. Which of the following statement s correct ?
View SolutionIf an element can exist in several oxidation states, it is convenient to display the reduction potentials corresponding to the various half reactions in diagrammatic from, known as Latimer diagram. The Latimer diagram for chlorine in acid solution is
Cl−4+1.20V−−−−→ClO−3+1.60V−−−−→ClO−2+1.60V−−−−→
ClO−+1.67V−−−−→Cl2+1.36V−−−−→Cl−
In basic solution is :
ClO−4+0.37V−−−−→ClO−3+0.30V−−−−→ClO−2+0.68−−−→
ClO−+0.42V−−−−→Cl2+1.36−−−→Cl−. The standard potentials for two nonadjacent species can also be calculated by using the concept that ΔG∘ is an additive property but using potential is not an assitive property and ΔG∘=−nFx0, If a given oxidation state is a the next higher oxidation state disproportionation can occur. The reverse of relative stabilities of the oxidation state can also be understood by drawing a graph of ΔG∘/F against oxidation state, known as Frost diagram, Choosing the stability of zero oxidation state arbitrery as zero. The most stable oxidation state of a apecies lies lowest in the digram Disproportionation is spontaneous if the species lies above a straight line joining its two product species.
For a hypothetical element, the Frost diagram is shown in figure
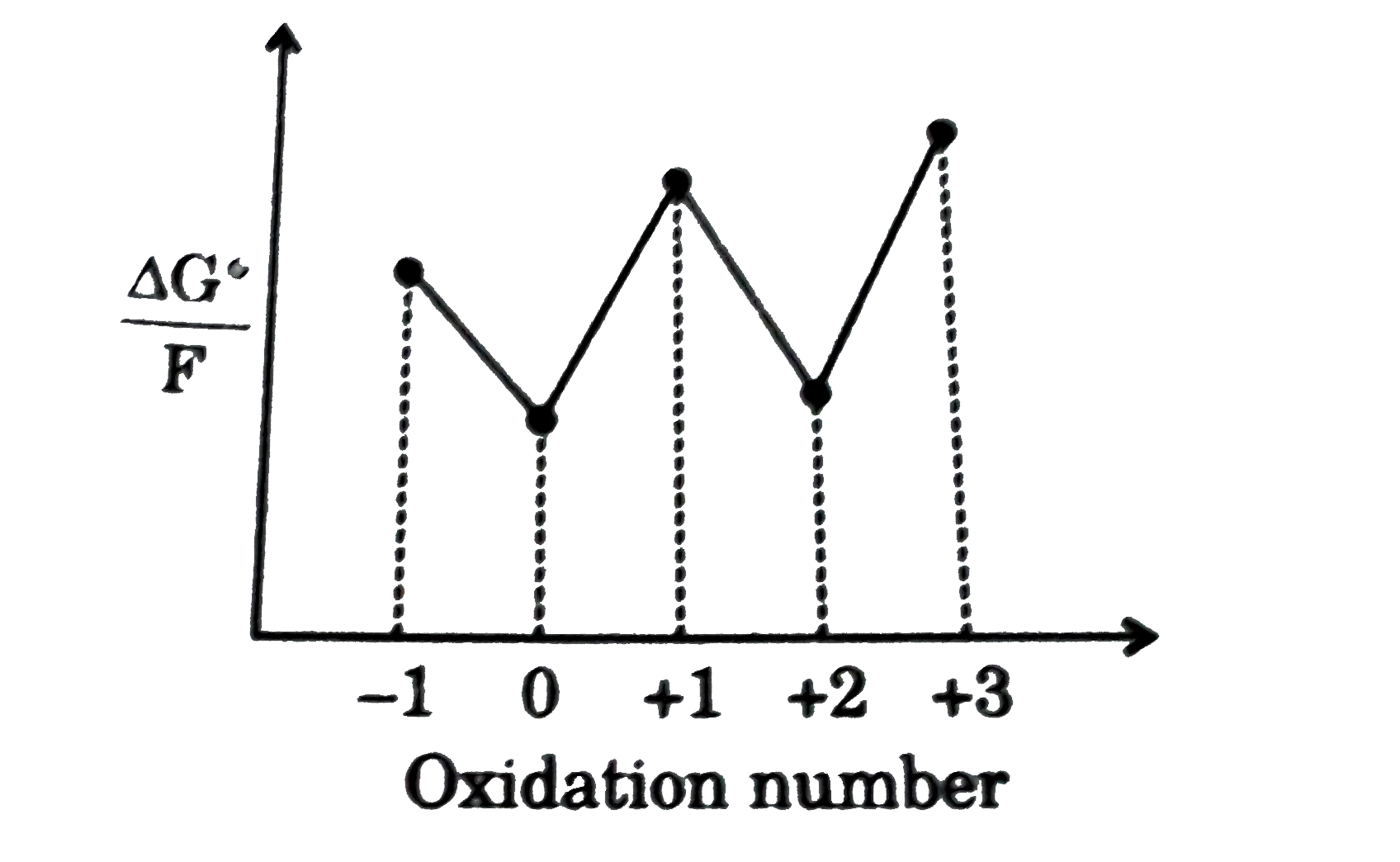
Which of the following oxidation state is least stable ?
View SolutionIf an element can exist in several oxidation states, it is convenient to display the reduction potentials corresponding to the various half reactions in diagrammatic from, known as Latimer diagram. The Latimer diagram for chlorine in acid solution is Cl_(4)^(-)overset(+1.20V)toClO_(3)^(-)overset(+1.60V)toClO_(2)^(-)overset(+1.60V)to ClO^(-)overset(+1.67V)toCl_(2)overset(+1.36V)toCl^(-) In basic solution is : ClO_(4)^(-)overset(+0.37V)toClO_(3)^(-)overset(+0.30V)toClO_(2)^(-)overset(+0.68)to ClO^(-)overset(+0.42V)toCl_(2)overset(+1.36)toCl^(-) . The standard potentials for two nonadjacent species can also be calculated by using the concept that DeltaG^(@) is an additive property but using potential is not an assitive property and DeltaG^(@)=-nFx^(0), If a given oxidation state is a the next higher oxidation state disproportionation can occur. The reverse of relative stabilities of the oxidation state can also be understood by drawing a graph of DeltaG^(@)//F against oxidation state, known as Frost diagram, Choosing the stability of zero oxidation state arbitrery as zero. The most stable oxidation state of a apecies lies lowest in the digram Disproportionation is spontaneous if the species lies above a straight line joining its two product species. Which of the following statement is correct according to above question ?
View SolutionIf an element can exist in several oxidation states, it is convernient to display the reduction potentials correspondingg to the various half reactions in diagrammatic form, know as latimer diagram the latimer diagram for chlorine in acid solutio is
CIO−4+1.20V−−−−→CiO−3+1.18V−−−−→HClO2+1.60V−−−−→HClO1.67V−−→Cl21.36V−−→Cl− in basic solution.
ClO−40.37V−−→ClO−30.30V−−→ClO−20.68V−−→ClO−0.42V−−→Cl21.36V−−→Cl− The standard potentials for two nonadjacent species can also be calculateed by using the concept that △G∘ as an additive property but potential is not an additive property and △G∘=−nFx0. if a given oxidation stateis a stronger oxidising agent than the next higher oxidation state, disproportionation can occur. The reverse of disproportionation is called comproportionation. The relative stabilities of the oxidation state can also be understood by drawing a graph of △G∘/F against oxidation state, known as frost diagram, choosing the stability of zero oxidation state arbitrarily as zero. The most stable oxidation state of a species lies lowest in the diagram, disproportionation is spontaneous if the species lies above a straight line joining its two product species.
Q. Which of the following couple have same value of potential at pH=0 and pH=14?
View SolutionIf an element can exist in several oxidation states, it is convernient to display the reduction potentials correspondingg to the various half reactions in diagrammatic form, know as latimer diagram the latimer diagram for chlorine in acid solutio is CIO_(4)^(-)overset(+1.20V)toCiO_(3)^(-)overset(+1.18V)toHClO_(2)overset(+1.60V)toHClOoverset(1.67V)toCl_(2)overset(1.36V)toCl^(-) in basic solution. ClO_(4)^(-)overset(0.37V)toClO_(3)^(-)overset(0.30V)toClO_(2)^(-)overset(0.68V)toClO^(-)overset(0.42V)toCl_(2)overset(1.36V)toCl^(-) The standard potentials for two nonadjacent species can also be calculateed by using the concept that triangleG^(@) as an additive property but potential is not an additive property and triangleG^(@)=-nFx^(0) . if a given oxidation stateis a stronger oxidising agent than the next higher oxidation state, disproportionation can occur. The reverse of disproportionation is called comproportionation. The relative stabilities of the oxidation state can also be understood by drawing a graph of triangleG^(@)//F against oxidation state, known as frost diagram, choosing the stability of zero oxidation state arbitrarily as zero. The most stable oxidation state of a species lies lowest in the diagram, disproportionation is spontaneous if the species lies above a straight line joining its two product species. Q. What is the potential couple (ClO^(-))/(Cl^(-)) at pH=14 ?
View SolutionIf an element can exist in several oxidation states, it is convernient to display the reduction potentials correspondingg to the various half reactions in diagrammatic form, know as latimer diagram the latimer diagram for chlorine in acid solutio is
CIO−4+1.20V−−−−→CiO−3+1.18V−−−−→HClO2+1.60V−−−−→HClO1.67V−−→Cl21.36V−−→Cl− in basic solution.
ClO−40.37V−−→ClO−30.30V−−→ClO−20.68V−−→ClO−0.42V−−→Cl21.36V−−→Cl− The standard potentials for two nonadjacent species can also be calculateed by using the concept that △G∘ as an additive property but potential is not an additive property and △G∘=−nFx0. if a given oxidation stateis a stronger oxidising agent than the next higher oxidation state, disproportionation can occur. The reverse of disproportionation is called comproportionation. The relative stabilities of the oxidation state can also be understood by drawing a graph of △G∘/F against oxidation state, known as frost diagram, choosing the stability of zero oxidation state arbitrarily as zero. The most stable oxidation state of a species lies lowest in the diagram, disproportionation is spontaneous if the species lies above a straight line joining its two product species.
Q. Which of the following statement is correct?
View SolutionIf an element can exist in several oxidation states, it is convernient to display the reduction potentials correspondingg to the various half reactions in diagrammatic form, know as latimer diagram the latimer diagram for chlorine in acid solutio is CIO_(4)^(-)overset(+1.20V)toCiO_(3)^(-)overset(+1.18V)toHClO_(2)overset(+1.60V)toHClOoverset(1.67V)toCl_(2)overset(1.36V)toCl^(-) in basic solution. ClO_(4)^(-)overset(0.37V)toClO_(3)^(-)overset(0.30V)toClO_(2)^(-)overset(0.68V)toClO^(-)overset(0.42V)toCl_(2)overset(1.36V)toCl^(-) The standard potentials for two nonadjacent species can also be calculateed by using the concept that triangleG^(@) as an additive property but potential is not an additive property and triangleG^(@)=-nFx^(0) . if a given oxidation stateis a stronger oxidising agent than the next higher oxidation state, disproportionation can occur. The reverse of disproportionation is called comproportionation. The relative stabilities of the oxidation state can also be understood by drawing a graph of triangleG^(@)//F against oxidation state, known as frost diagram, choosing the stability of zero oxidation state arbitrarily as zero. The most stable oxidation state of a species lies lowest in the diagram, disproportionation is spontaneous if the species lies above a straight line joining its two product species. Q. For a hypothetical element, the frost diagram is shown in figure.? which of the following oxidation state is least stable?
View Solution
