A half ring of radius r has a linear charge density λ.The potential at the centre of the half ring is
kλR
kλπR
kπλR
kπλ
The correct Answer is:D
Step by step video, text & image solution for A half ring of radius r has a linear charge density lambda.The potential at the centre of the half ring is by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
A quarter ring of radius R is having uniform charge density λ. Find the electric field and potential at the centre of the ring.
View SolutionA charge Q is distributed uniformly on a ring of radius R as shown in the following diagrams. Find the electric potential at the centre O of the ring

View Solution
Knowledge Check
A thin non-conducting ring of radius R has a linear charge density λ=λ0cosθ, where θ is measured as shown. The total electric dipole moment of the charge distribution is
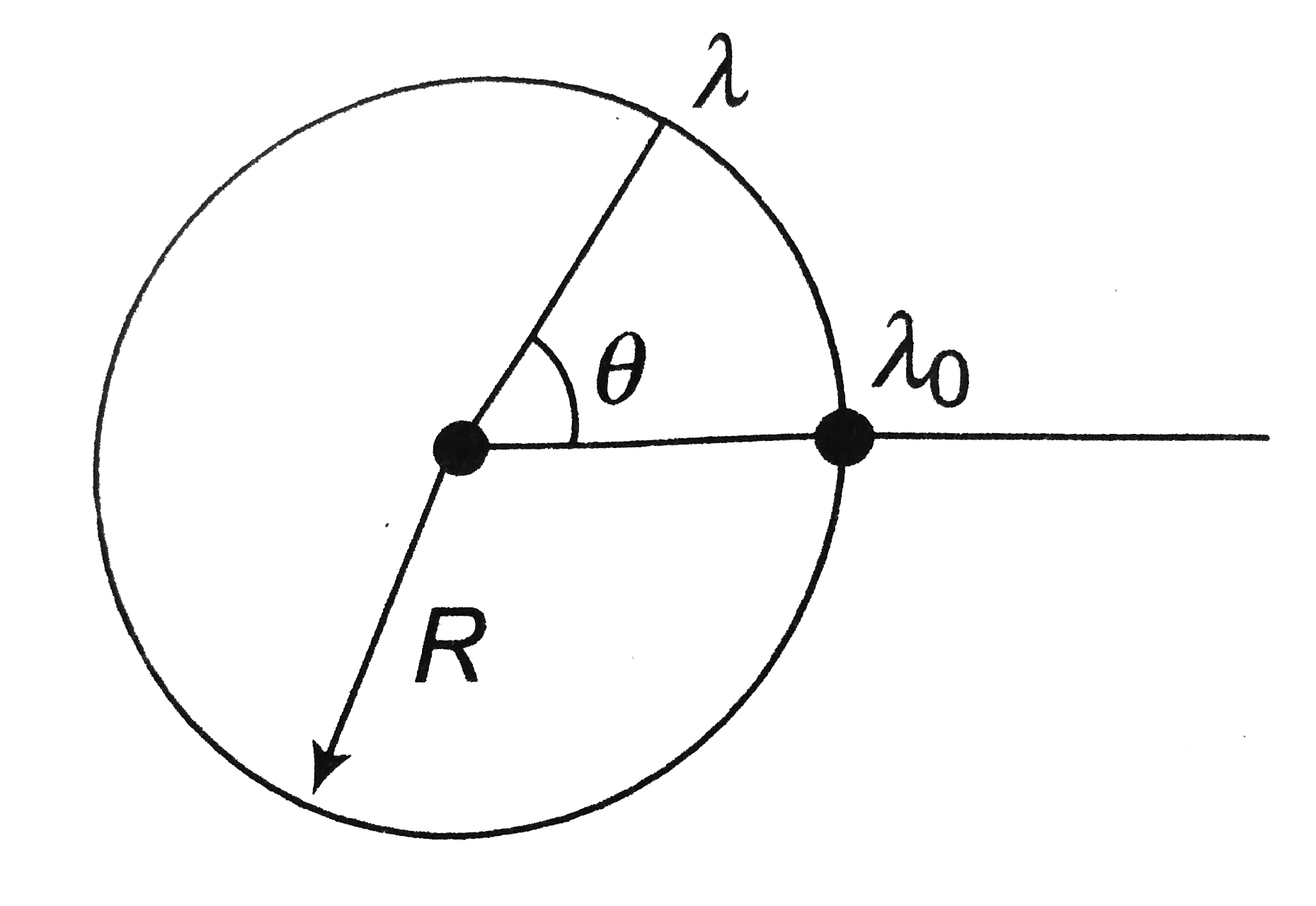 AR2λ0B2πR2λ0CπR2λ02DπR2λ0
AR2λ0B2πR2λ0CπR2λ02DπR2λ0A ring of radius R having a linear charge density λ moves towards a solid imaginary sphere of radius R2, so that the centre of ring passes through the centre of sphere. The axis of the ring is perpendicular to the line joining the centres of the ring and the sphere. The maximum flux through the sphere in this process is
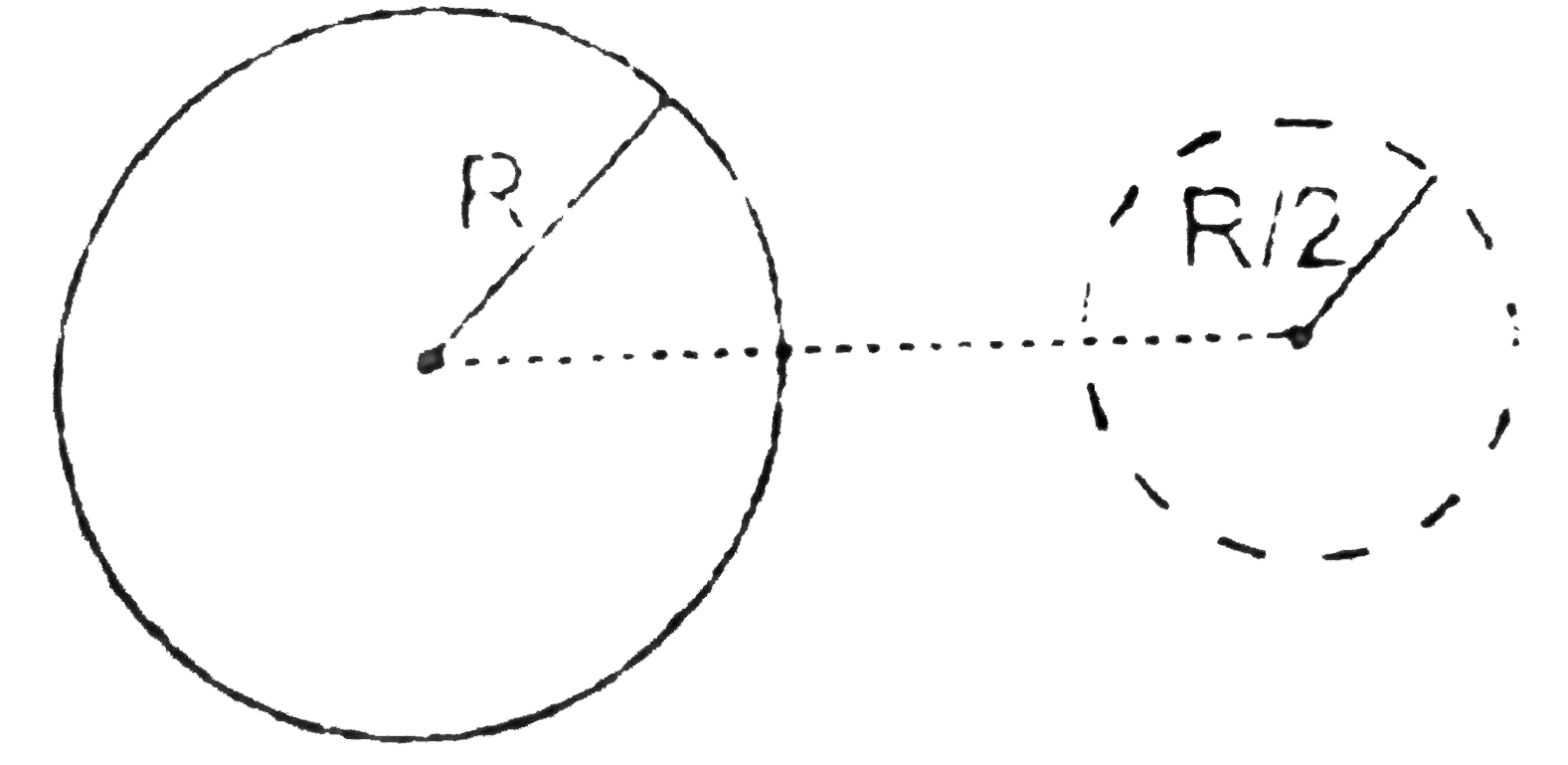 AλR∈0BλR2∈0CλπR4∈0DλπR3∈0
AλR∈0BλR2∈0CλπR4∈0DλπR3∈0
A sermicircular ring of radius R carries a uniform linear charge of λ. P is a point in the plane of the ring at a distance R from centre O. OP is perpendicular to AB. Find electric field intensity at point P.
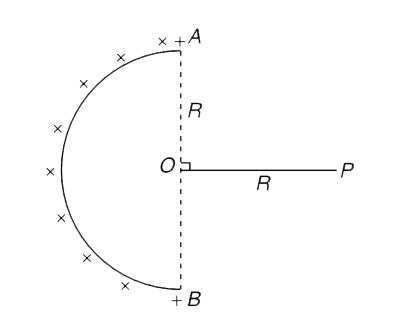
View SolutionA charge +q is distributed over a thin ring of radius r with line charge density λ=qsin2θ/(πr). Note that the ring is in the x-y plane and θ is the angle made by →r with the x axis. The work done by the electric force in displacing a point charge +Q from the center of the ring to infinity is
View SolutionA thin nonconducting ring of radius r has a linear charge density q=q0cosθ, where q0 is a constant and θ is the angle at the centre from the diameter of maximum charge density in the anticlockwise direction. Find the electric field at the centre of the ring.
View SolutionA charge Q is distributed uniformly on a ring of radius R as shown in the following diagrams. Find the electric potential at the center O of the ring.
(a)
View SolutionFind the electric field due to an infinitely long cylindrical charge distribution of radius R and having linear charge density λ at a distance half of the radius from its axis.
View SolutionConsider a circular ring of radius r, uniformly charged with linear charge density λ. Find the electric potential aty a point on the exis at a distance x from the centre of the ring. Using this expression for the potential, find the electric field at this point.
View Solution
