Three indential rings move with same speed on a horizontal magnetic field normal to plane of rings. The first (a) slips without rolling, the second(b) rolls without slipping and the third rolls with slipping:
The same emf is induced in all three rings
No emf is induced in any of the rings
In each ring all points are at the same potential.
B develops the maximum induced emf and A the least
The correct Answer is:A
Step by step video, text & image solution for Three indential rings move with same speed on a horizontal magnetic field normal to plane of rings. The first (a) slips without rolling, the second(b) rolls without slipping and the third rolls with slipping: by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
Knowledge Check
Kinetic energy of a body rolling without slipping is
AK=12mv2BK=12Iω2CK=12mv2+12Iω2DK=12mv2−12Iω2If a rigid body rolls on a surface without slipping, then:
Alinear speed is maximum at the highest point but minimum at the point of contactBlinear speed is minimum at highest point but maximum at the point of contactClinear speed is same at all points of the rigid bodyDangular speed is different at different points of a rigid bodyA disc roll on an inclined plane without slipping Rotational and total energy is
A3:1B1:3C√3:1D0:1
A thin hollow cylinder
rolls with the same speed without slipping.
Find the ratio of kinetic energies in the two cases.
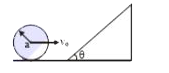
View SolutionA sphere of radius 'a' and mass 'm' rolls along a horizontal plane with constant speed v0. It encounters an inclined plane at angle θ and climbs upward. Assuming that it rolls without slipping, how far up the sphere will travel ?
View SolutionThe condition that a rigid body is rolling without slipping on an inclined plane is
View SolutionA ring starts to roll down the inclined plane of height h without slipping . The velocity when it reaches the ground is
View SolutionFrom an inclined plane a sphere, a disc, a ring and a shell are rolled without slipping. The order of their reaching at the base will be
View Solution


