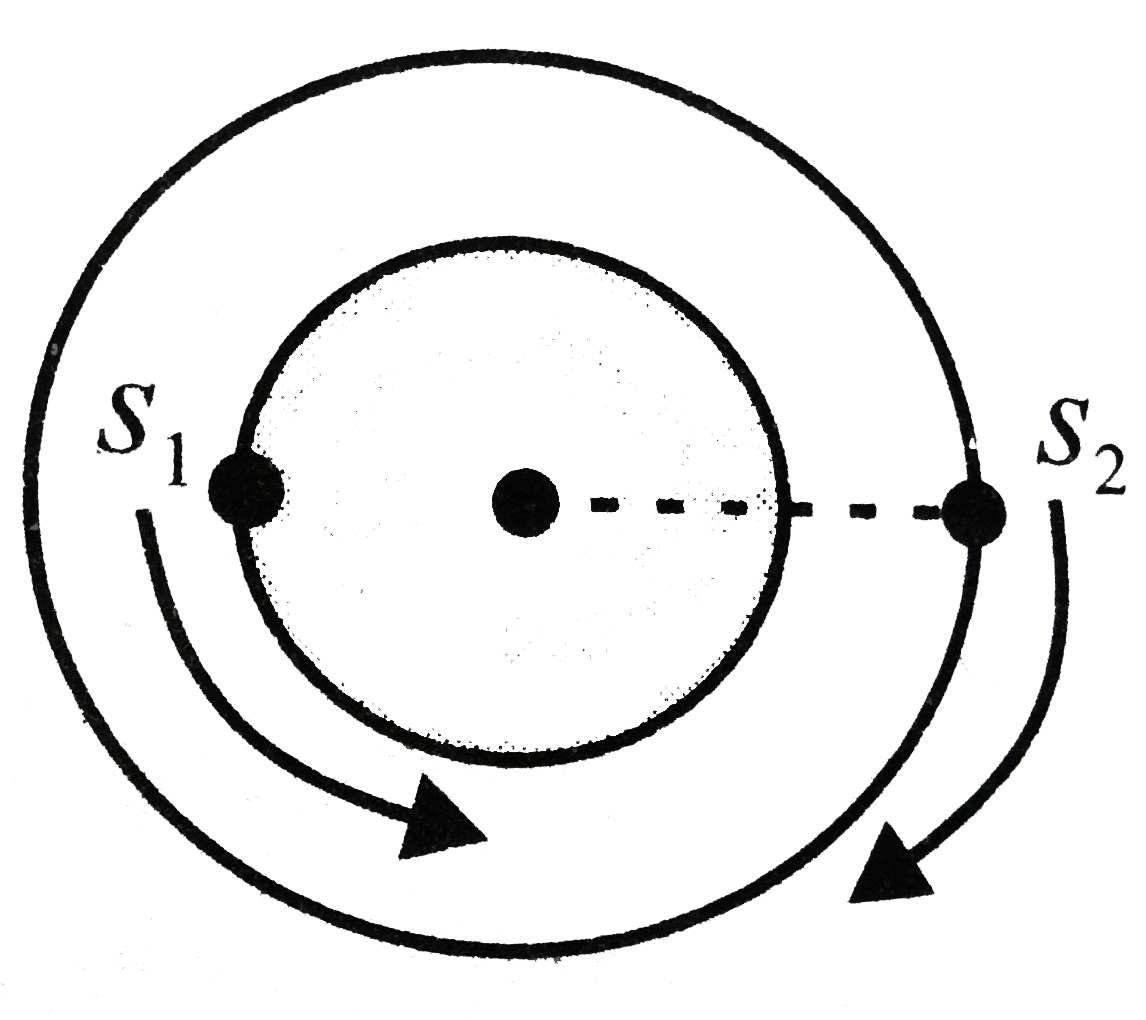
Two cars S1 and S2 are moving in coplanar concentric circular tracks in the opposite sense with the periods of revolution 3 min and 24 min, respectively. At time t = 0, the cars are farthest apart. Then, the two cars will be
closest to each other at t = 12 min and farthest at t = 18 min.
closest to each other at t = 3 min and farthest at t = 24 min
closest to each other at t = 6 min and farthest at t = 12 min
colsest to each other at t = 12 min and farthest at t = 24 min
The correct Answer is:D
Step by step video, text & image solution for Two cars S_(1) and S_(2) are moving in coplanar concentric circular tracks in the opposite sense with the periods of revolution 3 min and 24 min, respectively. At time t = 0, the cars are farthest apart. Then, the two cars will be by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
Two satellites S1 and S2 are revolving around the earth in coplanar concentric orbits in the opposite sense. At t=0, the position of satellites are shown in the diagram. The periods of S1 and S2 are 4h and 24h, respectively. The radius of orbit of S1 is 1.28×104km. For this situation, mark the correct statement (s).
View SolutionTwo cars, A and B, take the same time to go round around two concentric circular tracks of radii r1andr2, respectively. If r2=4r1, the ratio of the angular speed of car A to that of car B is
View Solution
Knowledge Check
Two satellite S1 and S2 revolve around a planet in coplanar circular orbits in the opposite sense. The periods of revolutions are T and ηT respectively. Find the angular speed of S2 as observed by an astronouts in S1, are observed by an astronaut in S1, when they are closest to each other.
Aω=2π(n−13+1)T(n13−1)Bω=2π(n−13+1)T2(n23−1)Cω=2π(n−13+1)T(n23−1)Dω=2π(n−23+1)T(n−13−1)Two satellites S1 and S2 revolve round a planet in coplanar circular orbits in the same sense. TI1eir periods of revolution are 2 hours and 16 hours respectively. If the radius of the orbit of S1 is 104, then the radius of the orbit of S2 is
A8×104 KmB2×104 KmC16×104 KmD4×104 kmTwo satellites S1 and S2 are revolving around a planet in the opposite sense in coplanar circular concentric orbits. At time t = 0, the satellites are farthest apart. The periods of revolution of S1 and S2 are 3 h and 24 h respectively. The radius of the orbit of S1 is 3×104 km. Then the orbital speed ofS2 as observed from
Athe planet is 4π×104 km h−1 when S2 is closest from S1.Bthe planet is 2π×104 km h−1 when S2 is closest from S1.CS1 is π×104 km h−1 when S2 is closest from S1DS1 is 3π×104 km h−1 when S2 is closest from S1
A racing car moving with constant acceleration cover two successive kilometers in 30 s and 20 s respectively What is the acceleration of the car?
View SolutionA cyclist moving a car circular track of raidus 40 cm completes half a revolution in 40 s. Its average velocity is
View SolutionTwo cars, A and B, take the same time to go round around two concentric circular tracks of radii r1andr2, respectively. If r2=4r1, the ratio of the angular speed of car A to that of car B is
View SolutionTwo cars A and B are going around concentric circular paths of τA and τB. If the two cars complete the circular paths in the same time then the ratio of angular speeds of A and B is
View SolutionA car is moving on a circular track of diameter 72 m with a speed of 6 m/s . It is acclereration at the rate of √3m/s2 If the mass of the car is 1000 kg , the net force acting on the car is :
View Solution