A star of mass M and radius R is made up of gases. The average gravitational pressure compressing the star due to gravitational pull of the gases making up the star depends on R as
1R4
1R
1R2
1R6
The correct Answer is:A
Step by step video, text & image solution for A star of mass M and radius R is made up of gases. The average gravitational pressure compressing the star due to gravitational pull of the gases making up the star depends on R as by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Topper's Solved these Questions
Similar Questions
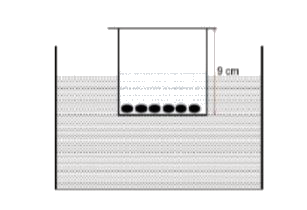
A wide bottom cylindrical massless plastic container of height 9 cm has 40 identical coins inside it and is floating on water with 3 cm inside the water. If we start putting more of such coins on its lid. It is observed that after N coins are put, its equilibrium changes from stable to unstable. Equilibrium in floating is stable if the geometric center of the submerged portion is above the center of mass of the object) The value of N is closed to
 03:53View Solution
03:53View SolutionWhy spin angular velocity of a star is greatly enhanced when it collapses under gravitational pull and becomes a neutron star ?
View SolutionA star can be considered as spherical ball of hot gas of radius R . Inside the star, the density of the gas is rho_(r) at radius r and mass of the gas within this region is M_(r) . A star in it's prime age is said to be under quilibrium due to gravitational pull and outward radiation pressure (p) . consider the shell of thikness dr as in the figure of previous question. if the pressure on this shell is dp then the correc equation is ( G is universal gravitational constant)
03:19View SolutionAn isolated triple star systerm consists of two identical stars, each of mass m and f fixed star of mass M. They revolve around the central star in the same circular orbit to radius r. The orbiting stars are always at opposite ends of a diameter of the orbit. The time period of revolution of each star around the fixed is equal to:
 View Solution
View SolutionA planet of mass m is moving around a star of mass M and radius R in a circular orbit of radius r. The star abruplly shrinks to half its radius without any loss of mass. What change will be there in the orbit of the planet ?
04:07View SolutionA system consists of two stars of equal masses that revolve in a circular orbit about their centre of mass . Orbital speed of each star is v and period is T . Find the mass M of each star . (G is gravitational constant )
View SolutionA star can be considered as a shpherical ball of hot gas of radius R.Inside the star the density of the gas is ρr at a radius r and mass of the gas within this region is Mr.The correct differential equation for variation of mass with respect to radius (dMrdt) is (refer to the adjacent figure)

A star in its prime age is said to be under equilibrium due to gravitational pull and outward radiation pressure (ρ).Consider the shell of thickness dr . If the pressure on this shell is dp then the correct equation is (G is universal gravitational constant)05:24View Solutionएक हल्का ग्रह,द्रव्यमान M के एक बहुत भारी तारे के चारों ओर R त्रिज्या की वृतीय कक्षा में परिक्रमण काल T से चक्कर लगा रहा है|यदि तारे एवं ग्रह के बीच लगने वाला गुरुत्वाकर्षण बल R−5/2 के अनुक्रमानुपाती हो तो सिद्ध कीजिए कि T2=4π2GMR7/2, जहाँ G सार्वत्रिक गुरुत्वीय स्थिरांक है|
07:05View SolutionA planet is revolving around a star in a circular orbit of radius R with a period T. If the gravitational force between the planet and the star is proportional to R^(-3//2) , then
06:16View Solutionएक बड़ा तरल तारा अपने गुरुत्वीय क्षेत्र के प्रभाव से अंतरिक्ष में दोलन करता हैं| तारे का दोलन काल T तारे की त्रिज्या R तरल के घनत्व ρ तथा सार्वत्रिक गुरुत्वीय नियतांक G पर निर्भर करता है| विमीय विधि से आवर्तकाल T के लिए सूत्र स्थापित कीजिए |
04:09View Solutionयदि कोई छोटा ग्रह किसी विशाल तारे के चारों ओर R त्रिज्या के किसी वृत्ताकार कक्ष में T परिक्रमण काल के साथ घूम रहा है। यदि ग्रह तथा तारे के मध्य गुरुवीय बल R−5/2 के समानुपाती है, तो T समानुपाती होगा-
02:46View SolutionImagine a light planet revolving around a very massive star in a circular orbit of radius r with a period of revolution T.If the gravitational force of attraction between the planet and the star is proportional to r−3 ,then the square of the time period will be proportional to
View SolutionImagine a light planet is revolving round a very massive star in a circular orbit of radius R with a time period of revolution T. If the gravitational force of attraction between the star and planetis propostional to R−n, then T2 is propostional to
02:06View SolutionAn impermeable star shrinks itself from the initial radius R in such a way that its mass remains fixed. Which of the given curves represents the gravitational acceleration on the star's surface as a function of the radius of the star?
02:54View SolutionA system consists of two stars of equal masses that revolve in a circular orbit about their centre of mass . Orbital speed of each star is v and period is T . Find the mass M of each star . (G is gravitational constant )
01:53View Solution

