Which is a solution to (8x)(24)=(12)x ?
−2
−1
−112
0
The correct Answer is:B
To solve the equation (8x)(24)=(12)x, we can follow these steps:
Step 1: Rewrite 8 as a power of 2
We know that 8=23. Therefore, we can rewrite 8x as:
8x=(23)x=23x
Now, substitute this back into the equation:
(23x)(24)=(12)x
Step 2: Simplify the left-hand side
Using the property of exponents that states am⋅an=am+n, we can combine the terms on the left:
23x+4=(12)x
Step 3: Rewrite the right-hand side
The expression 12 can be rewritten as 2−1. Thus, we have:
(12)x=(2−1)x=2−x
Now, we can rewrite the equation as:
23x+4=2−x
Step 4: Set the exponents equal to each other
Since the bases are the same, we can set the exponents equal to each other:
3x+4=−x
Step 5: Solve for x
Now, we will solve for x:
1. Add x to both sides:
3x+x+4=0
This simplifies to:
4x+4=0
2. Subtract 4 from both sides:
4x=−4
3. Divide by 4:
x=−1
Final Answer
Thus, the solution to the equation is:
−1
We know that 8=23. Therefore, we can rewrite 8x as:
8x=(23)x=23x
Now, substitute this back into the equation:
(23x)(24)=(12)x
Using the property of exponents that states am⋅an=am+n, we can combine the terms on the left:
23x+4=(12)x
The expression 12 can be rewritten as 2−1. Thus, we have:
(12)x=(2−1)x=2−x
Now, we can rewrite the equation as:
23x+4=2−x
Since the bases are the same, we can set the exponents equal to each other:
3x+4=−x
Now, we will solve for x:
1. Add x to both sides:
3x+x+4=0
This simplifies to:
4x+4=0
2. Subtract 4 from both sides:
4x=−4
3. Divide by 4:
x=−1
Thus, the solution to the equation is:
−1
Topper's Solved these Questions
Similar Questions
Knowledge Check
- Question 1 - Select One
The solution set of −812<−12−4x<712,x∈W on the number line is:
A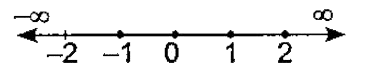 B
B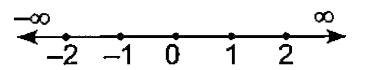 C
C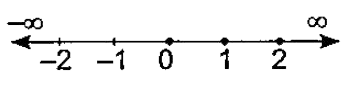 D
D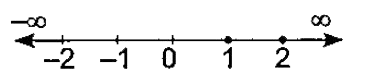
- Question 2 - Select One
In the real numbers, what is the solution of the equation 4x+2=82x−1?
A−74B−14C54D74 - Question 3 - Select One
Which of the following is a solution of −72√3x−8+3=−4x+7 for x.
A−8B−0.18C0.18D8
The number of integral solutions of x+1x2+2>14 is
View SolutionIf y(x) is solution of xdydx+2y=x2,y(1)=1 then value of y(12)= (a) −4916 (b) 4916 (c) 458 (d) −458
View SolutionIf x is not equal to 2 or -2 . Which s equivalent to 3x2−8x+4x2−4 ?
View SolutionWhich of the following are solutions to the quadratic equation (x−1)2−49?
View SolutionWhich is the equivalent form of (x−3)(x2+4x−8) ?
View Solution

