Rate of heat flow through a cylindrical rod is . Temperatures of ends of rod are and . If all the dimensions of rod become double and temperature difference remains same and rate of heat flow becomes . Then is . Find value of x.
To solve the problem, we will use the formula for the rate of heat flow through a cylindrical rod, which is given by:
H=K⋅A⋅(T1−T2)L
where:
- H is the rate of heat flow,
- K is the thermal conductivity of the material,
- A is the cross-sectional area,
- T1 and T2 are the temperatures at the ends of the rod,
- L is the length of the rod.
Step 1: Calculate H1
For the original rod, we have:
- Cross-sectional area A=πR2
- Length L
Thus, the rate of heat flow H1 is given by:
H1=K⋅A⋅(T1−T2)L=K⋅πR2⋅(T1−T2)L
Step 2: Calculate H2 for the doubled dimensions
When the dimensions of the rod are doubled:
- New radius R′=2R
- New length L′=2L
The new cross-sectional area A′ becomes:
A′=π(R′)2=π(2R)2=4πR2
Now, we can calculate H2:
H2=K⋅A′⋅(T1−T2)L′=K⋅4πR2⋅(T1−T2)2L
This simplifies to:
H2=2K⋅πR2⋅(T1−T2)L=2H1
Step 3: Find the ratio H1H2
Now, we find the ratio of H1 to H2:
H1H2=H12H1=12
Step 4: Express the ratio in terms of 0.x
From the problem, we know that:
H1H2=0.x
Since we found that H1H2=12=0.5, we can equate:
0.x=0.5
This implies that x=5.
Final Answer
Thus, the value of x is:
5
- H is the rate of heat flow,
- K is the thermal conductivity of the material,
- A is the cross-sectional area,
- T1 and T2 are the temperatures at the ends of the rod,
- L is the length of the rod.
For the original rod, we have:
- Cross-sectional area A=πR2
- Length L
When the dimensions of the rod are doubled:
- New radius R′=2R
- New length L′=2L
Now, we find the ratio of H1 to H2:
From the problem, we know that:
Thus, the value of x is:
Topper's Solved these Questions
Similar Questions
Find the rate of heat flow through a cross section of the rod shown in figure (θ2>θ1 . Thermal conductivity of the material of the rod is K.

View SolutionThe heat is flowing through two cylinderical rods of same material. The diameters of the rods are in the ratio 1:2 and their lengths are in the ratio 2:1. If the temperature difference between their ends is the same, the ratio of rates of flow of heat through them will be
View Solution
Knowledge Check
A cylinderical rod has temperature T1andT2 at its ends. The rate of flow of heat is Q1 cal/s. if all the linear dimensions are doubled keeping temperature constant then rate of flow of heat Q2 will be
A4Q1B2Q1CQ14DQ12A cylindrical rod having temperature T1 and T2 at its ends. The rate of flow of heat is Q1cal/sec. If all the linear dimensions are doubled keeping temperature constant, then rate of flow of heat Q2 will be
A4Q1B2Q1CQ14DQ12The rate of heat flow through a slab is Pcond. If the slab thickness is doubled, its cross-sectional area is halved, and the temperature difference across it is doubled, then the rate of heat flow becomes
A2PcondBPcond2CPcondDPcond8
The ratio of the diameters of two metallic rods of the same material is 2:1 and their lengths are in the ratio 1:4. If the temperature difference between their ends are equal, the rate of flow of heat in them will be in the ratio
View SolutionRate of heat flow through two conducting rods of identiclal dimensions having thermal conductivities K1 and K2 and Q1 and Q2 when their ends are maintained at the same difference of temperature individually. When the two rods are joined in series with their ends maintained at the same temperature difference (as shown in the figure), the rate of heat flow will be

View Solution1 kcal of heat flowing through a rod of iron.When the rod is cut down to 4 pieces then what will be the heat flowing through each piece having same differential temperature ?
View SolutionHeat is flowing through two cylindrical rods made of same materials whose ends are maintained at similar temperature. If diameters of the rods are in ratio 1:2 and lengths in ratio 2:1, then the ratio of thermal current through them in steady state is:
View SolutionTwo rods one is semi circular of thermal conductivity K1 and otheir is straight of thermal conductivity K2 and of same cross sectional area are joined as shown in the The points A and B are maintained at same temperature difference. If rate of flow of heat is same in two rods then K1/K2 is
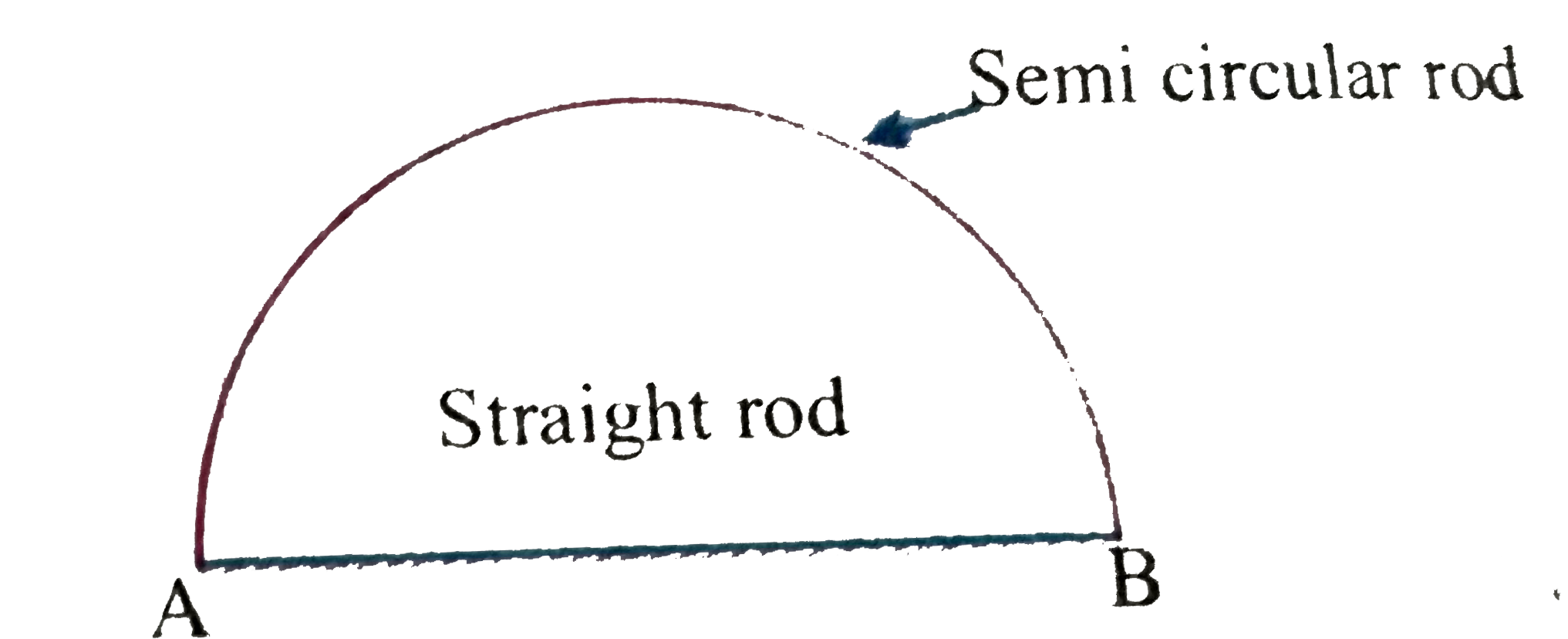 .
.
View Solution
