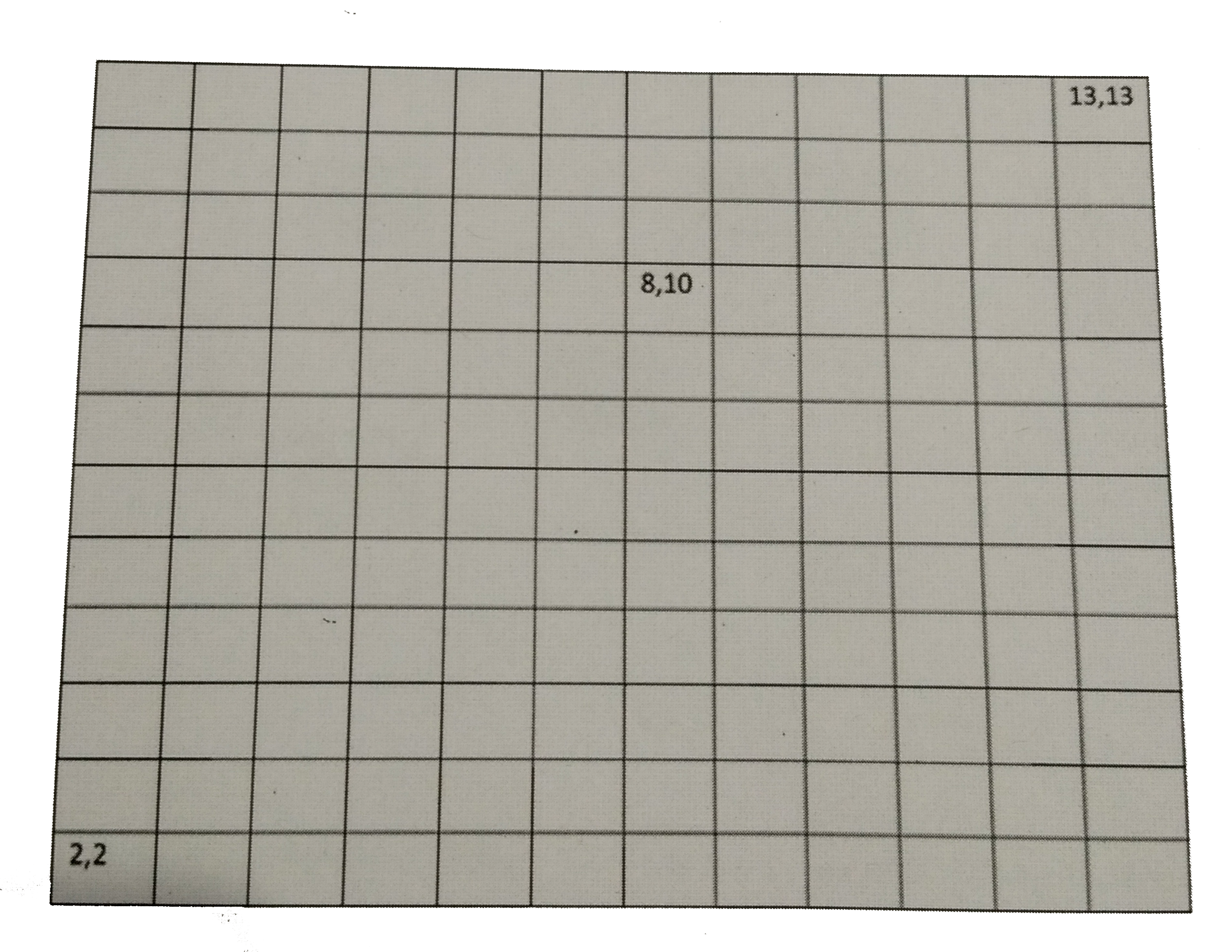
Max starts on his way to Pewter Town, for the first Gym Battle. On the way, he bumps into a mysterious man offering a chance at catching loads of Pokémon. “What do I need to do?” asks Max. The mysterious man replies “Here’s a map of Viridian Forest. You will begin at (8,10). Every grid has an unique Pokémon. But if your position is (a,b), you can only move to (a,a+b),(a,a−b),(a+b,b),(a−b,b),(b−a,b) and (a,b−a). You can continue until you have no Pokémon under your reach.” What is the sum of digits of the maximum number of Pokemons Max can catch?
Video Solution
Text Solution
This is based on the fact that HCF or GCD of (a,a-b) or (a-b,b) is the same that of (a,b). This Follows from the fact that every mutual factor of a and b are also the factor of a+b and |a-b|.
And so becomes the grid. This will happen at each stage of reach for some (a,b), Thus lion can only reach those points whose gcd or hcf is (8,10)==2, Therefore the answer is all the coordinates whose hcf is 2,
{(2,2)}∗1
{(2,4)(4,6)(6,8)(8,10)(10,12)(2,6)(6,10)(2,8)(4,10)
(2,10)(2.12)}∗2 (As these could be both a,b and b,a)
|
Updated on:21/07/2023
Similar Questions
Knowledge Check
- Question 1 - Select One
Have you been doing what has been asks of you?
Abeen doingBwhat has been asks of you?CNo errorDHave you
What do you mean by symptoms of a disease?
View SolutionDo you think the camel renders no service to man?
View SolutionWhat are the mysterious ways in which Macavity acts?(YEAR 2016)
View SolutionWhat do you understand by cathedral towns?
View SolutionDo you belong to a town? What things cause annoyance to you there?
View SolutionA mystery I propose to explain.”
What is the mystery the speaker proposes to explain?View SolutionHe asked, “What will you do if you fail in business ?”
View Solution

