A slit 4.0 cm wide is irradiated with microwaves of 2.0 cm. Find the angular spread of central maximum assuming incidence normal to the plane of the slit.
To find the angular spread of the central maximum for a single slit diffraction pattern, we can use the formula for the angular width of the central maximum, which is given by:
sinθ=λa
where:
- θ is the angle from the central maximum to the first minimum,
- λ is the wavelength of the wave,
- a is the width of the slit.
1. Identify the given values:
- Width of the slit, a=4.0cm=0.04m
- Wavelength of the microwaves, λ=2.0cm=0.02m
2. Use the formula for the angle:
We can rearrange the formula to find θ:
sinθ=λa
3. Substitute the values into the formula:
sinθ=0.02m0.04m=0.5
4. Calculate θ:
To find θ, we take the inverse sine:
θ=arcsin(0.5)
From trigonometric values, we know:
θ=30∘
5. Determine the angular spread of the central maximum:
The angular spread is typically considered as the angle from the central maximum to the first minimum on either side. Thus, the total angular spread is:
Total angular spread=2θ=2×30∘=60∘
Final Answer:
The angular spread of the central maximum is 60∘.
- θ is the angle from the central maximum to the first minimum,
- λ is the wavelength of the wave,
- a is the width of the slit.
- Width of the slit, a=4.0cm=0.04m
- Wavelength of the microwaves, λ=2.0cm=0.02m
We can rearrange the formula to find θ:
sinθ=λa
sinθ=0.02m0.04m=0.5
To find θ, we take the inverse sine:
θ=arcsin(0.5)
From trigonometric values, we know:
θ=30∘
The angular spread is typically considered as the angle from the central maximum to the first minimum on either side. Thus, the total angular spread is:
Total angular spread=2θ=2×30∘=60∘
The angular spread of the central maximum is 60∘.
Topper's Solved these Questions
Similar Questions
A slit 4.0 cm wide is irradiated with microwaves od wavelengths 2.0 cm. Find the angular spread of the central maximum, assuming incidence normal to the plane of the slit.
View SolutionMicrowaves of frequency 24,000 MHz are incident normally on a rectengular on a rectangular slit of width 5 cm. Calculate the angular spread of the central maximum of the diffraction pattern of the slit.
View Solution
Knowledge Check
- Question 1 - Select One
A slit 4.0 cm wide is irradiated with microwaves of 2.0 cm. Find the angular spread of central maximum assuming incidence normal to the plane of the slit.
A30∘B40∘C45∘D60∘ - Question 2 - Select One
A slit whose width is 5.0 cm is irradiated with microwaves of wavelength 3 cm. What is the angular spread of the central maximum? Assume normal incidence.
A±18∘,24'B±36∘52'C+43∘23'D−33∘35' - Question 3 - Select One
A slit 5 cm wide when irradiated by waves of wavelength 10 mm results in the angular spread of the central maxima on either side of incident light by about :
A1/2 radianB1/4 radianC3 radianD1/5 radian
A monochromatic light of wavelength 500nm is incident normally on a single slit of width 0.2mm to produce a diffraction pattern. Find the angular width of central maximum obtained on the screen, 1m away.
Estimate the number of fringes obtained in YDSE with fringe width 0.5mm, which can be accommodated within the region of total angular spread of the central maximum due to a single slit.View Solution(a) Explain two features to distinguish between the interference pattern in Young's double slit experiment with the difference pattern obtained due to a single slit.
(b) A monochromatic light of wavelength 500nm is incident normally on a single slit of width 0.2 nm of produce a diffraction pattern. Find the angular width of the central maximum obtained on the screen.
Estimate the number of fringes obtained in Young's double slit experimental with fringe width 0.5mm, which can be accommodated within the region of total angular spread of the central maximum due to single slit.View SolutionA monochromatic light of wavelength 500nm is incident normally on a single slit of width 0.2mm to produce a diffraction pattern. Find the angular width of central maximum obtained on the screen, 1m away.
Estimate the number of fringes obtained in YDSE with fringe width 0.5mm, which can be accommodated within the region of total angular spread of the central maximum due to a single slit.View SolutionA coherent parallel beam of microwaves of wavelength λ=0.5mm falls on a Young's double- slit apparatus. The separation between the slits is 1.0 mm. The intensity of microwaves is measured on a screen placed parallel to the plane of the slits at a distance of 1.0 m from it as shown in Fig. 2.42.
If the incident beam makes an angle or 30∘ with the x-axis (as in the dotted arrow shown in the figure), find the y-coordinates of the first minima on either side of the central maximum.
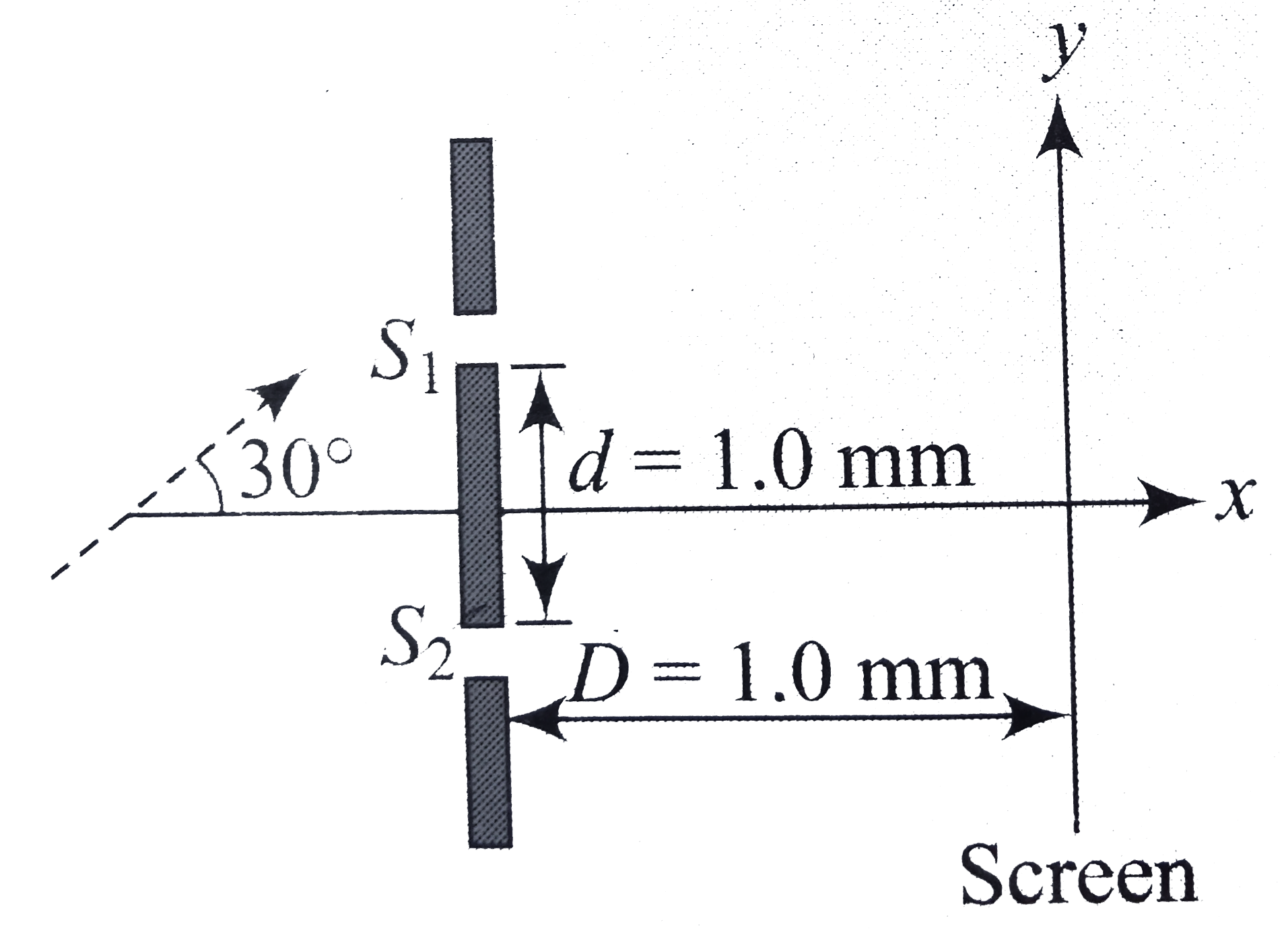 View Solution
View Solution(a) Explain two features to distinguish between the interference patternn in Young's double-slit experiment with the diffraction pattern obtained due to a single-slit.
(b) A monochromaticlight of wavelength 500 nm is incident normally on a single-slit of width 0.2mm to produce a diffraction pattern. find the angular width of the central maximum obtained on the screen.
Estimate the number of fringes obtained in Young's double-slit experiment with fringe width 0.5mm, which can be accommodated within the region of total angular apread of the central maximum due to single-slit.View Solution

