A narrow monochromatic beam of light of intensity I is incident on a glass plate. Another identical glass plate is kept close to the first one and parallel to it. Each plate reflects 25% of the incident light and transmits the remaining. Calculate the ratio of minimum and maximum intensity in the interference pattern fromed by two beams obtained after reflection from each plate.
To solve the problem, we will analyze the intensity of the light beams reflected and transmitted by the two glass plates, and then calculate the maximum and minimum intensities in the interference pattern.
1. Identify the Initial Intensity:
Let the intensity of the incident beam be I.
2. Calculate the Intensities After Reflection and Transmission:
- First Plate:
- The intensity reflected by the first plate (I2) is:
I2=0.25⋅I=14I
- The intensity transmitted through the first plate (I3) is:
I3=0.75⋅I=34I
- Second Plate:
- The intensity reflected by the second plate (I4) from the transmitted beam (I3) is:
I4=0.25⋅I3=0.25⋅34I=316I
- The intensity transmitted through the second plate (I5) is:
I5=0.75⋅I4=0.75⋅316I=964I
3. Calculate the Amplitudes:
- The amplitude of the light wave is related to intensity by the relation I∝A2.
- Let A2 be the amplitude corresponding to I2 and A5 be the amplitude corresponding to I5:
A2=√I2=√14I=12√I
A5=√I5=√964I=38√I
4. Calculate the Amplitude Ratio:
- The amplitude ratio R is given by:
R=A2A5=12√I38√I=1/23/8=86=43
5. Calculate Maximum and Minimum Intensities:
- The maximum intensity Imax and minimum intensity Imin in the interference pattern are given by:
Imax=(R+1)2andImin=(R−1)2
- Substituting R=43:
Imax=(43+1)2=(43+33)2=(73)2=499
Imin=(43−1)2=(43−33)2=(13)2=19
6. Calculate the Ratio of Minimum and Maximum Intensities:
- The ratio IminImax is:
IminImax=19499=149
Final Answer:
The ratio of minimum and maximum intensity in the interference pattern is 1:49.
Let the intensity of the incident beam be I.
- First Plate:
- The intensity reflected by the first plate (I2) is:
I2=0.25⋅I=14I
- The intensity transmitted through the first plate (I3) is:
I3=0.75⋅I=34I
- The intensity reflected by the second plate (I4) from the transmitted beam (I3) is:
I4=0.25⋅I3=0.25⋅34I=316I
- The intensity transmitted through the second plate (I5) is:
I5=0.75⋅I4=0.75⋅316I=964I
- The amplitude of the light wave is related to intensity by the relation I∝A2.
- Let A2 be the amplitude corresponding to I2 and A5 be the amplitude corresponding to I5:
A2=√I2=√14I=12√I
A5=√I5=√964I=38√I
- The amplitude ratio R is given by:
R=A2A5=12√I38√I=1/23/8=86=43
- The maximum intensity Imax and minimum intensity Imin in the interference pattern are given by:
Imax=(R+1)2andImin=(R−1)2
- Substituting R=43:
Imax=(43+1)2=(43+33)2=(73)2=499
Imin=(43−1)2=(43−33)2=(13)2=19
- The ratio IminImax is:
IminImax=19499=149
The ratio of minimum and maximum intensity in the interference pattern is 1:49.
Topper's Solved these Questions
- BOOK - SL ARORACHAPTER - WAVE OPTICSEXERCISE - Based on Wave nature of light12videos
WAVE OPTICS
- BOOK - SL ARORACHAPTER - WAVE OPTICSEXERCISE - Based on Young s Double Slit experiment22videos
WAVE OPTICS
- BOOK - SL ARORACHAPTER - WAVE OPTICSEXERCISE - EXERCISE2videos
WAVE OPTICS
- BOOK - SL ARORACHAPTER - UNIVERSEEXERCISE - PROBLEM_TYPE6videos
UNIVERSE
Similar Questions
_E01_107_Q01.png)
A narrow monochromatic beam of light of intensity I is incident on a galss plate as shown in figure. Another identical glass plate is kept close to the first one & parallel to it. Each glass plate reflects 25% of the light incident on it % transmits the remaining find the ratio of the minimum & the maximum intensities in the interference pattern formed by the two beams obtained after one reflection at each plate.View SolutionA narrow monochromatic beam of light of intensity 1 is incident on a glass plate as shown in figure Another identical glass plate is kept close to the first one and parallel to it. Each glass plate reflects 25% of the light incident on it and transmits intensities in the interference pattern formed by two beams obtained after one reflection at each plate.
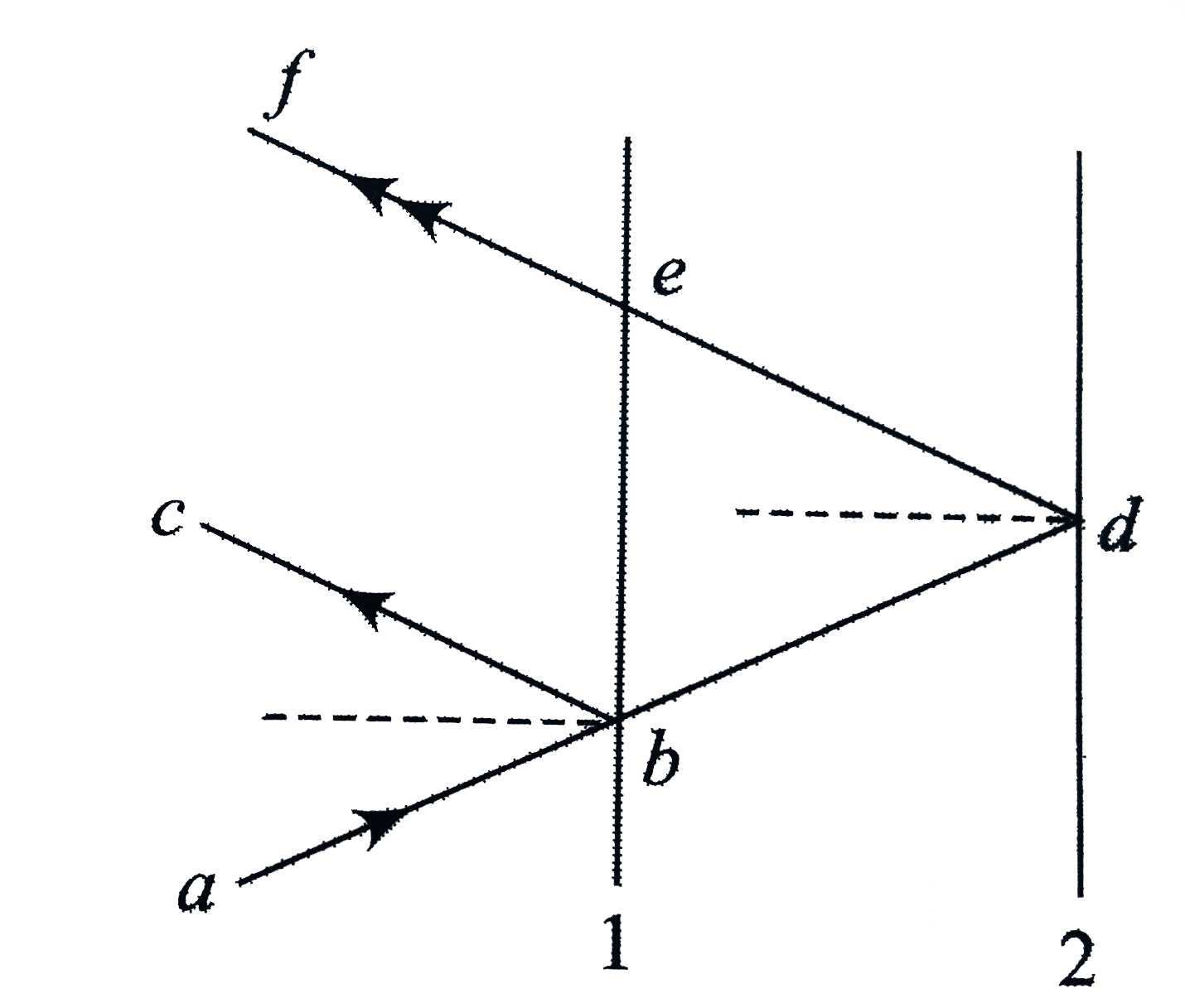 View Solution
View Solution
Knowledge Check
- Question 1 - Select One
A parallel beam of light of intensity I is incident on a glass plate. 25% of light is reflected in any reflection by upper surface and 50% of light is reflected by any reflection from lower surface. Rest is refracted The ratio of maximum to minimum intensity in interference region of reflected rays is
 A⎛⎜ ⎜⎝12+√3812−√38⎞⎟ ⎟⎠2B⎛⎜ ⎜⎝14+√3812−√38⎞⎟ ⎟⎠2C58DNone of these
A⎛⎜ ⎜⎝12+√3812−√38⎞⎟ ⎟⎠2B⎛⎜ ⎜⎝14+√3812−√38⎞⎟ ⎟⎠2C58DNone of these - Question 2 - Select One
A ray of light intensity I is incident on a parallel glass-slab at a point A as shown in figure. It undergoes partial reflection and refraction. At each reflection 25% of incident energy is reflected. The rays AB and A′B′ undergo interference. The ratio Imax/Imin is
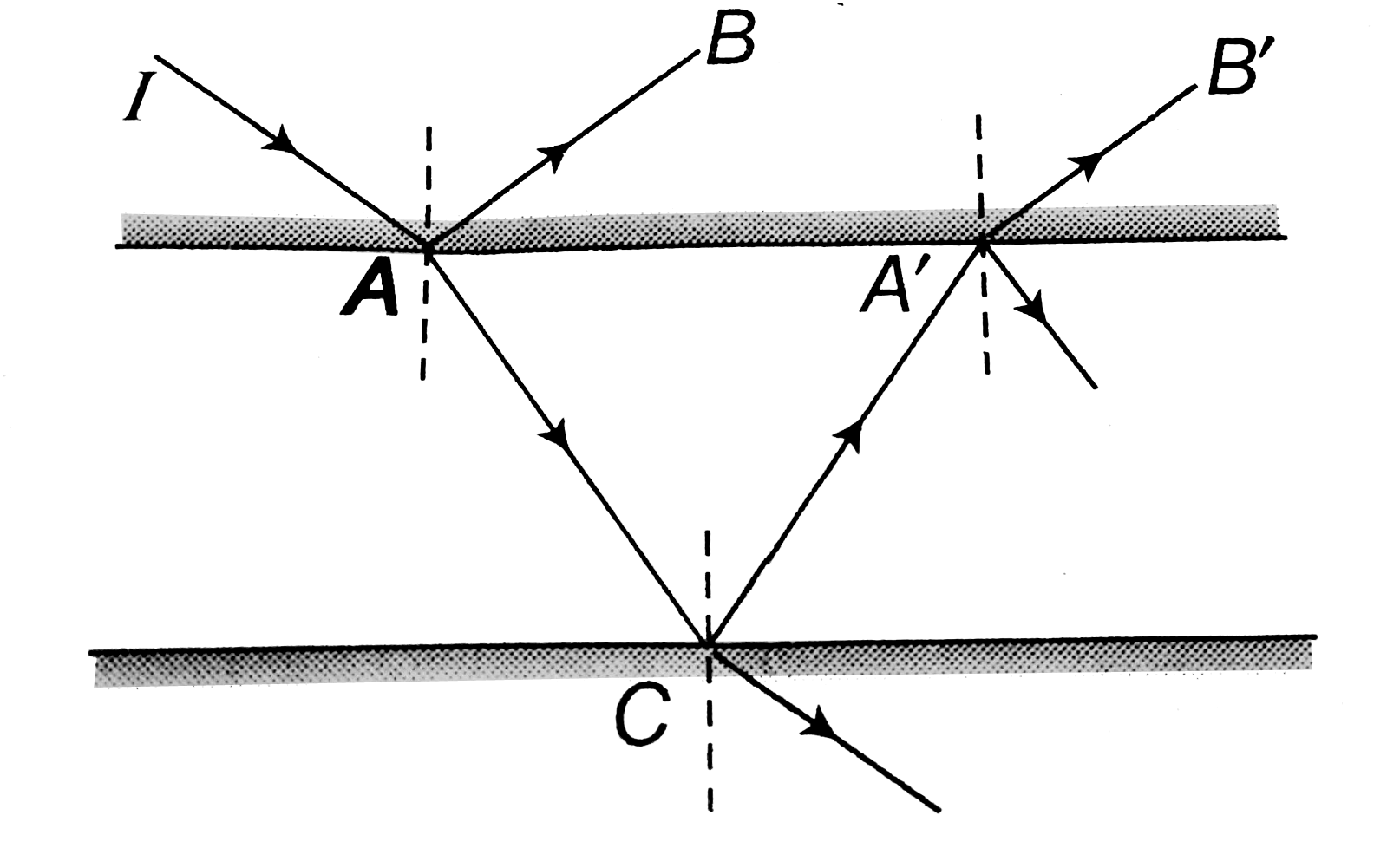 A49:1B7:1C4:1D8:1
A49:1B7:1C4:1D8:1 - Question 3 - Select One
A ray of light of intensity I is incident on a parallel glass slab at a point A as show. It undergoes partial reflection and refraction. At each reflaction 25% of incident energy is reflected. The rays AB and A'B' undergo interference. The ratio Imax/Imin is
 A4:1B8:1C7:1D49:1
A4:1B8:1C7:1D49:1
Two thin glass plates are placed parallel to each other such that distance between them is very small. Each glass plate reflects 25% of incident energy and transmits remaining 75% Light beam is incident on this system. Consider the interference of two light beams obtained after one reflection from each glass plate. Calculate ratio of the maximum to minimum intensity.
View SolutionA plate is kept in front of a beam of photons. The plate reflects 40% of the incident photons and absorbs the remainder. If the plate absorbs energy at a rate 1200J−1s, find the net force acting on it.
View Solution(a) If one of two identical slits producing interference in Young's experiment is covered with glass so that the light intensity passing through it is reduced to 50%, find the ratio of the maximu and minimum intensity of the fringe in the interference pattern.
(b) What kind of fringes do you expect to observe if white light is used instead of monochromatic light ?View Solution_E01_093_Q01.png)
A ray light of intensity I is incident on a parallel glass-slab at a point A as shown in figure it undergoes partial reflection and refraction. At each reflection 20% of incident energy is refracted rays AB and A'B' undergo interference find the ratio Imax/IminView SolutionA beam of light strikes a glass plate at an angle of incident 60∘ and reflected light is completely polarised than the refractive index of the plate is-
View Solution

