A survey was taken of the value of homes in a county, and it was found that the mean home value was $165,000 and the median home value was $125,000. Which of the following situations could explain the difference between the mean and median home values in the county?
The homes have values that are close to each other.
There are a few homes that are valued much less than the rest.
There are a few homes that are valued much more than the rest.
Many of the homes have values between $125,000 and $165,000.
Choice C is correct. The mean and median values of a data set are equal when there is a symmetrical distribution. For example, a normal distribution is symmetrical. If the mean and the median values are not equal, then the distribution is not symmetrical. Outliers are a small group of values that are significantly smaller or larger than the other values in the data. When there are outliers in the data, the mean will be pulled in their direction (either smaller or larger) while the median remains the same. The example in the question has a mean that is larger than the median, and so an appropriate conjecture is that large outliers are present in the data, that is, that there are a few homes that are valued much more than the rest.
Choice A is incorrect because a set of home values that are close to each other will have median and mean values that are also close to each other. Choice B is incorrect because outliers with small values will tend to make the mean lower than the median. Choice D is incorrect because a set of data where many homes are valued between $125,000 and $165,000 will likely have both a mean and a median between $125,000 and $165,000.
Similar Questions
Knowledge Check
A study of gasoline prices across a particular state showed that the state's mean gas price was $2.87 per gallon, and the median gas price was $3.25 per gallon. Which of the following most likely accounts for the difference between the mean and median gas prices in this state?
AA few areas of the state had substantially lower gas prices than the rest.BA few areas of the state had substantially highest gas prices than the rest.CThe majority of areas of areas in the study has gas prices between $2.87 and $3.25 per gallon.DAn error was made during data collection or data analysis.A company purchased a machine valued at $120,000. The value of the machine depreciates by the same amount each year so that after 10 years the value will be $30,000. Which of the following equations gives the value, v, of the machine, in dollars, t years after it was purchased for 0≤t≤10 ?
Av = 30,000 − 9,000tBv = 120, 000 − 9, 000tCv = 120,000 + 9,000tDv = 120,000 − 30,000tThe mean of the numbers a, b, 8, 5, 10 is 6 and the variance is 6.80. Then which one of the following gives possible values of a and b?
Aa = 0, b = 7Ba = 5, b = 2Ca = 1, b = 6Da = 3, b = 4
The mean and median of 100 items are 50 and 52 respectively. The value of the largest item is 100. It was later found that it is actually 110. Then the true mean and median are
View SolutionFor which of the following function 9s) Lagrange's mean value theorem is not applicable in [1,2] ?
View SolutionThe table below gives the results of three titrations carried out with 0.200 M HCl to determine the molarity of a given NaOH solution using phenolphthalein as indicator. NaOH was taken in the burette and HCl was taken in a conical flask for the titrations
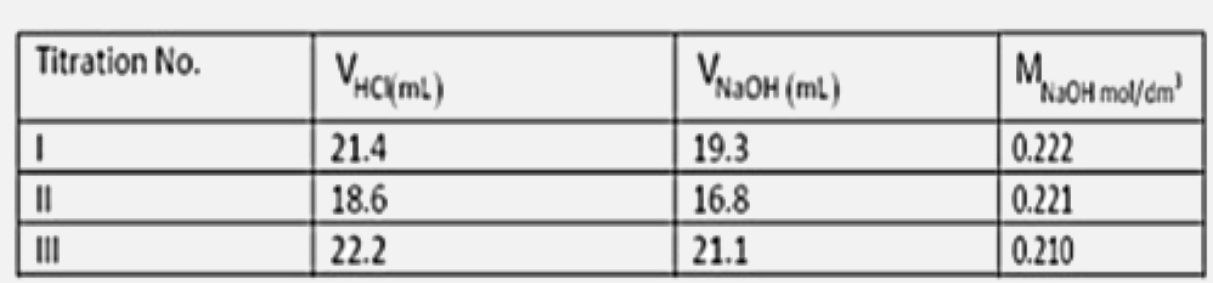
The actual molarity of the prepared NaOH solution was 0.220 mol dm−3. Which among the following could be the reason for the wrong value obtained in titration III?
View SolutionChris plans to purchase a car that loses it value at rate of 14% per year. If the initial cost of the car is $27,000, which of the following equations should Chris use to determine the value, v, of the car after 4 years?
View SolutionIf the range of a set of integers is 2 and the mean is 50, which of the following statements must be true?
I. The mode is 50.
II. The median is 50.
III. There are exactly three data value.
View Solution
