A hollow sphere of radius r is put inside another hollow sphere of radius R. The charges on the two are +Q and -q as shown in the figure. A point P is located at a distance x from the common centre such that r<x<R. The potential at the point P is
14πε0(Q−qx)
14πε0(QR−qr)
14πε0(QR−qx)
14πε0(qr−Qx)
The correct Answer is:C
To find the electric potential at point P located at a distance x from the common center of two concentric hollow spheres with charges +Q and −q, we will follow these steps:
Step 1: Understand the Configuration
We have two hollow spheres:
- The inner sphere has a radius r and charge +Q.
- The outer sphere has a radius R and charge −q.
- The point P is located at a distance x from the center, where r<x<R.
Step 2: Determine the Potential Due to Each Sphere
1. Potential due to the Outer Sphere (V1):
- For a hollow sphere, the electric field inside the sphere is zero. Therefore, the potential inside the outer sphere (at point P) is constant and equal to the potential at its surface.
- The potential V1 due to the outer sphere at the surface is given by:
V1=kQR
where k is the Coulomb's constant.
2. Potential due to the Inner Sphere (V2):
- The potential inside a hollow charged sphere is also constant and equal to the potential at its surface.
- The potential V2 due to the inner sphere at the surface is given by:
V2=k(−q)r
where −q is the charge on the inner sphere.
Step 3: Calculate the Total Potential at Point P
- The total potential VP at point P is the sum of the potentials due to both spheres:
VP=V1+V2
Substituting the expressions for V1 and V2:
VP=kQR+k(−q)r
Simplifying this gives:
VP=kQR−kqr
Final Expression for the Potential at Point P
Thus, the potential at point P is given by:
VP=kQR−kqr
We have two hollow spheres:
- The inner sphere has a radius r and charge +Q.
- The outer sphere has a radius R and charge −q.
- The point P is located at a distance x from the center, where r<x<R.
1. Potential due to the Outer Sphere (V1):
- For a hollow sphere, the electric field inside the sphere is zero. Therefore, the potential inside the outer sphere (at point P) is constant and equal to the potential at its surface.
- The potential V1 due to the outer sphere at the surface is given by:
V1=kQR
where k is the Coulomb's constant.
- The potential inside a hollow charged sphere is also constant and equal to the potential at its surface.
- The potential V2 due to the inner sphere at the surface is given by:
V2=k(−q)r
where −q is the charge on the inner sphere.
- The total potential VP at point P is the sum of the potentials due to both spheres:
VP=V1+V2
Substituting the expressions for V1 and V2:
VP=kQR+k(−q)r
Simplifying this gives:
VP=kQR−kqr
Thus, the potential at point P is given by:
VP=kQR−kqr
Topper's Solved these Questions
- BOOK - DC PANDEYCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITORSEXERCISE - (B) Chapter exercises20videos
ELECTROSTATIC POTENTIAL AND CAPACITORS
- BOOK - DC PANDEYCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITORSEXERCISE - (C) Chapter exercises50videos
ELECTROSTATIC POTENTIAL AND CAPACITORS
- BOOK - DC PANDEYCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITORSEXERCISE - Check point 2.520videos
ELECTROSTATIC POTENTIAL AND CAPACITORS
- BOOK - DC PANDEYCHAPTER - ELECTROMAGNETIC WAVESEXERCISE - Sec C22videos
ELECTROMAGNETIC WAVES
- BOOK - DC PANDEYCHAPTER - ELECTROSTATICSEXERCISE - Medical entrances gallery37videos
ELECTROSTATICS
Similar Questions
Inside a charged thin conducting shell of radius R, a point charge +Q is placed as shown in figure potential of shell may be
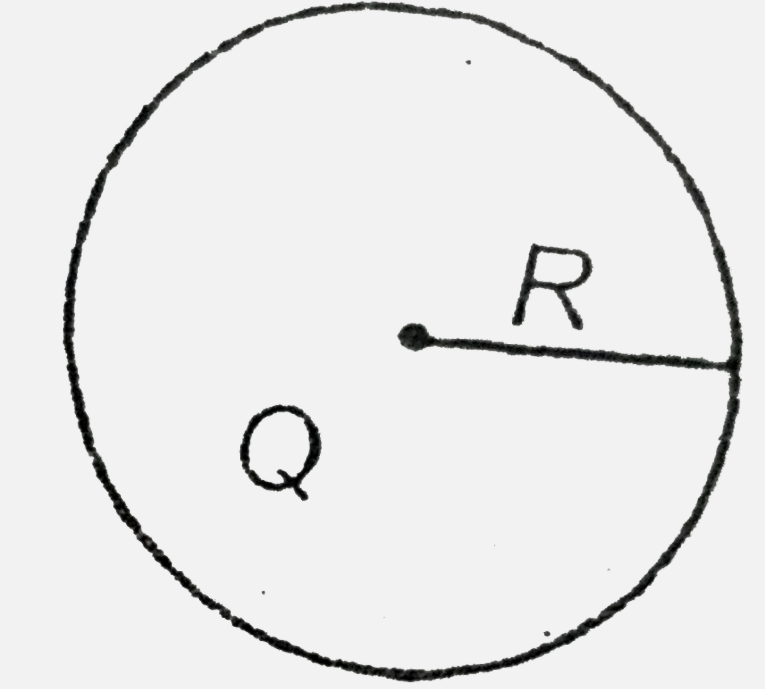 View Solution
View SolutionA solid sphere of mass m and radius r is placed inside a hollow thin spherical shell of mass M and radius R as shown in figure−6.30. A particle of mass m' is placed on the line joining the two centres at a distance x from the point of contact of the sphere and the shell. Find the magnitude of the resultant gravitational force on this - particle due to the sphere and the shell if (a) r<x<2r,(b)2r<x<2Rand(c)x>2R.
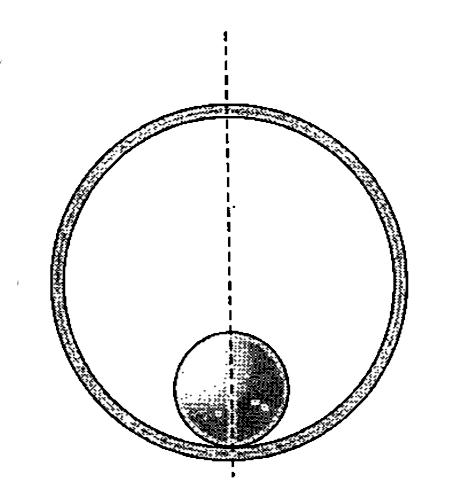 View Solution
View Solution
Knowledge Check
- Question 1 - Select One
A point charge q is placed at a distance of r from cebtre of an uncharged conducting sphere of rad R(<r). The potential at any point on the sphere is
AzeroB14πε0qrC14πε0qRr2D14πε0qr2R - Question 2 - Select One or More
A hollow conducting sphere of inner radius r and outer radius 2R is given charge Q as shown in figure, then the
 Apotential at A and B is same.Bpotential at O and B is same.Cpotential at O and C is same.Dpotential at A,B,C and O is same.
Apotential at A and B is same.Bpotential at O and B is same.Cpotential at O and C is same.Dpotential at A,B,C and O is same. - Question 3 - Select One
If charge q is induced on outer surface of sphere of radius R, then intensity at a point P at a distance S from its centre is
Ainversely proportional to (S+R)2Binversely proportional to R2Cinversely proportional to S2Ddirectly proportional to S2
Inside a neutral hollow conducting sphere of radius X and centre C, a point charge q is placed as shown in the figure. Another point charge q1, is placed outside the sphere at distance d from centre. The net- electrostatic force on charge q placed at the centre is K=14πε0
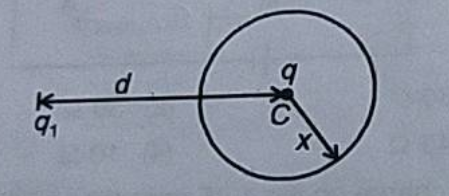 View Solution
View SolutionA sphere of radius 2R and mas M has a spherical cavity of radius R as shown in the figure. Find the value of gravitational field at a point P at a distance of 6R from centre of the sphere.
 View Solution
View SolutionThere is a conductor with cavity of radius R as shown in figure. A point charge Q is placed at R/2 distance from centre of cavity.
A point P is at r distance from centre of conductor. Then,
 View Solution
View SolutionA small conducting sphere of radius r is lying concentrically inside a bigger hollow conducting sphere of radius R. The bigger and smaller spheres are charged with Q and q(Q>q) and are insulated from each other. The potential difference between the spheres will be
View SolutionA hollow sphere of radius 2R is charged to V volts and another smaller sphere of radius R is charged to V/2 volts. Now the smaller sphere is placed inside the bigger sphere without changing the net charge on each sphere. The potential difference between the two spheres would be
View Solution

