The apparent depth of an object at the bottom of tank filled with a liquid of refractive index 1.3 is 7.7 cm. what is the actual depth of the liquid in the tank ?
Step by step video & image solution for The apparent depth of an object at the bottom of tank filled with a liquid of refractive index 1.3 is 7.7 cm. what is the actual depth of the liquid in the tank ? by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- RAY OPTICSBOOK - SL ARORACHAPTER - RAY OPTICSEXERCISE - TYPE E
10 Videos
- RAY OPTICSBOOK - SL ARORACHAPTER - RAY OPTICSEXERCISE - TYPE G
19 Videos
- RAY OPTICSBOOK - SL ARORACHAPTER - RAY OPTICSEXERCISE - TYPE C
17 Videos
- OPTICAL INSTRUMENTSBOOK - SL ARORACHAPTER - OPTICAL INSTRUMENTSEXERCISE - Problems for self practice
35 Videos
- SOLIDS AND SEMICONDUCTOR DEVICESBOOK - SL ARORACHAPTER - SOLIDS AND SEMICONDUCTOR DEVICESEXERCISE - PROBLEM_TYPE
9 Videos
Similar Questions
An object is placed at the bottom of a tank filled with liquid of refractive index 1.6. If the actual depth of the tank is 10 cm, what will be the apparent depth of the object?
View SolutionA spherical shaped fish bowl filled with water of refractive index 1.3 is placed in another large tank filled with another liquid of refractive index 1.4 such that rim of the fish bowl is slightly above the liquid filled in the tank. The diameter of the fish bowl is 20 cm. A parallel light rays from a distant object is incident on the spherical fish bowl. Find the position at which incident light rays will get focused when seen by a small fish at the centre of the bowl.
View Solution
Knowledge Check
A vessle of depth d is first filled to (23)rd of its depth with a liquid of refractive index 32 and the rest of the vessel is filled with a liquid of refractive index 43. What is the apparent depth of the inner surface of the bottom of the vessle.
A5d16B29d36C25d36D15d16The apparent depth of a needle laying at the bottom of the tank, which is filled with water of refractive index 1.33 to a height of 12.5 cm is measured by a microscope to be 9.4cm. If water is replaced by a liquid of refractive index 1.63 up to the same height. What distance would the microscope have to be moved to focus on the needle again ?
A1.73cmB2.13cmC1.5cmD2.9cmA metal coin at the bottom of a beaker filled with a liquid of refractive index 4/3 to height of 6cm. To an observer looking from above the surface of the liquid, coin will appear at a depth of (consider paraxial rays).
A7.5cmB6.75cmC4.5cmD1.5cm
A tank is filled with water to a height of 12.5cm The apparent depth of a needle lying at the bottom of the tank is measured by a microscope to be 9.4cm. What is the refractive index of water ? If water is replaced by a liquid of refractive index 1.63 upto the same height, by what distance would the microscope have to be moved to focus on the needle again ?
View SolutionA tank is filled with water to a height of 12.5cm. The apparent depth of a needle lying at the bottom of the tank is measured by a microscope to be 9.4cm. What is the refractive index of water ? If water is replaced by a liquid of refractive index 1.63 upto the same height, by what distance would the microscope have to be moved to focus on the needle again ?
View SolutionA tank is filled with water to a height of 12.5 cm. The apparent depth of a needle lying at the bottom of the tank is measured by a microscope to be 9.4 cm. What is the refractive index of water? If water is replaced by a liquid of refractive index 1.63 up to the same height, by what distance would the microscope have to be moved to focus on the needle again?
View Solution
A real image of an object is formed by a conex lens at the bottom of an empty beaker. The beaker is now filled with a liquid of refractive index 1.4 to a depth of 7 cm. In order to get the image again at the bottom the beaker shoud be moved
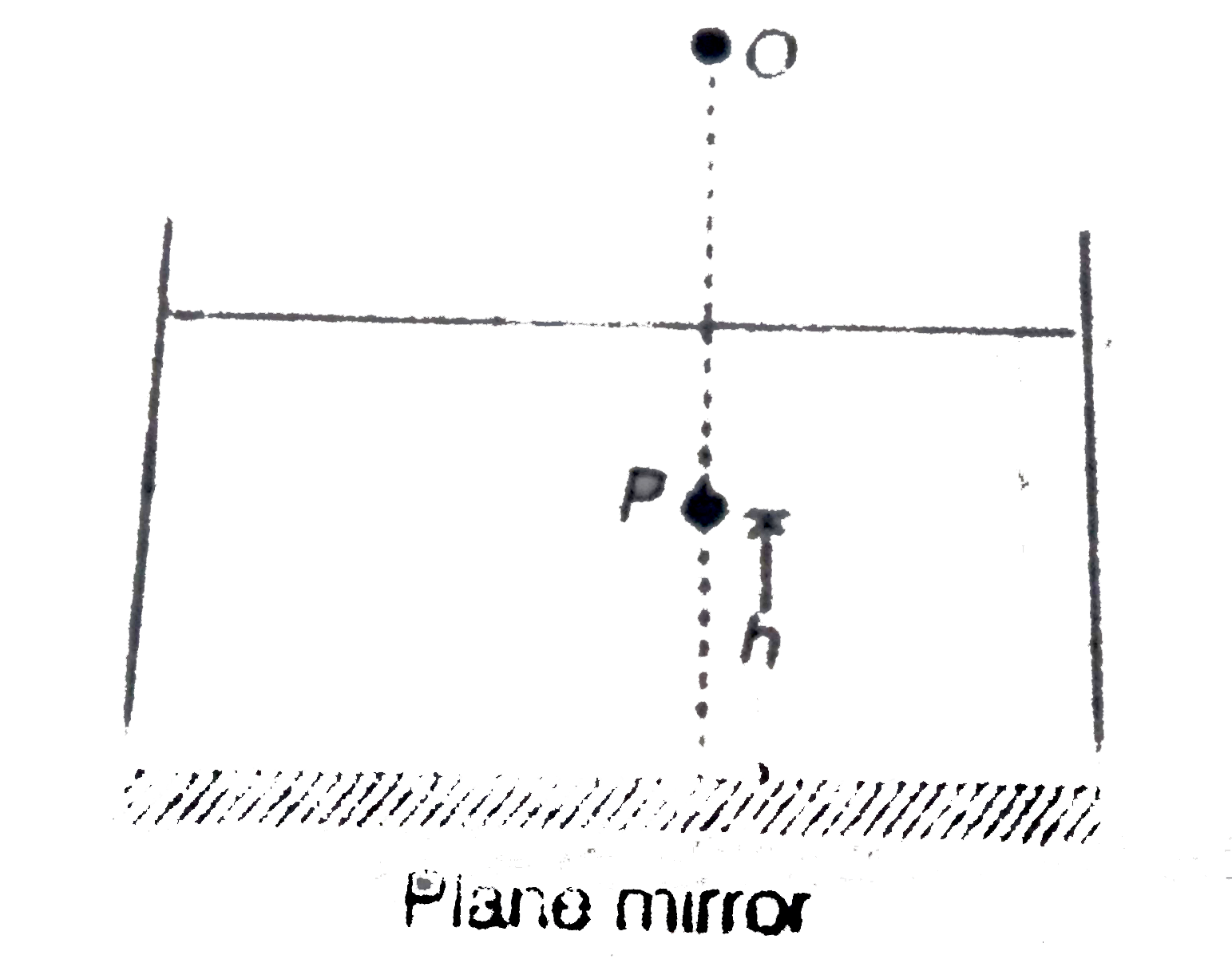
View SolutionA plane mirror is placed at the bottom of a tank containing a liquid of refractive index μ. P is a small object at a height h above the mirror. An observes P and its image in the mirror. The apparent distance between two will be
View Solution
