The bottom of a container is a 4.0 cm thick glass (μ=1.5) slab. The container contains two immiscible liquids A and B of depth 6.0 cm and 8.0 cm respectively. What is the apparent position of a scratch on the outer surface of the bottom of the glass slab when viewed through the container? Refractive indices of A and B are 1.4 and 1.3 respectively.
To find the apparent position of a scratch on the outer surface of the bottom of the glass slab when viewed through the container, we will follow these steps:
Step 1: Understand the Problem
We have a glass slab of thickness 4.0 cm with a refractive index (μ) of 1.5. Above the glass slab, there are two immiscible liquids: Liquid A with a depth of 6.0 cm and a refractive index of 1.4, and Liquid B with a depth of 8.0 cm and a refractive index of 1.3. We need to find the apparent position of a scratch on the outer surface of the glass slab.
Step 2: Use the Formula for Apparent Depth
The apparent depth (D) can be calculated using the formula:
D=T(1−1μ1)+T1(1−1μ2)+T2(1−1μ3)
where:
- T is the thickness of the glass slab (4.0 cm),
- T1 is the depth of liquid A (6.0 cm),
- T2 is the depth of liquid B (8.0 cm),
- μ1 is the refractive index of glass (1.5),
- μ2 is the refractive index of liquid A (1.4),
- μ3 is the refractive index of liquid B (1.3).
Step 3: Substitute Values into the Formula
Substituting the values into the formula:
D=4(1−11.5)+6(1−11.4)+8(1−11.3)
Step 4: Calculate Each Term
1. For the glass slab:
4(1−11.5)=4(1−0.6667)=4×0.3333=1.3332cm
2. For liquid A:
6(1−11.4)=6(1−0.7143)=6×0.2857=1.7142cm
3. For liquid B:
8(1−11.3)=8(1−0.7692)=8×0.2308=1.8464cm
Step 5: Sum the Results
Now, sum all the calculated apparent depths:
D=1.3332+1.7142+1.8464=4.8938cm
Step 6: Round the Result
Rounding to two decimal places, the apparent depth is approximately:
D≈4.89cm
Final Answer
The apparent position of the scratch on the outer surface of the bottom of the glass slab is approximately 4.89 cm.
---
We have a glass slab of thickness 4.0 cm with a refractive index (μ) of 1.5. Above the glass slab, there are two immiscible liquids: Liquid A with a depth of 6.0 cm and a refractive index of 1.4, and Liquid B with a depth of 8.0 cm and a refractive index of 1.3. We need to find the apparent position of a scratch on the outer surface of the glass slab.
The apparent depth (D) can be calculated using the formula:
D=T(1−1μ1)+T1(1−1μ2)+T2(1−1μ3)
where:
- T is the thickness of the glass slab (4.0 cm),
- T1 is the depth of liquid A (6.0 cm),
- T2 is the depth of liquid B (8.0 cm),
- μ1 is the refractive index of glass (1.5),
- μ2 is the refractive index of liquid A (1.4),
- μ3 is the refractive index of liquid B (1.3).
Substituting the values into the formula:
D=4(1−11.5)+6(1−11.4)+8(1−11.3)
1. For the glass slab:
4(1−11.5)=4(1−0.6667)=4×0.3333=1.3332cm
6(1−11.4)=6(1−0.7143)=6×0.2857=1.7142cm
8(1−11.3)=8(1−0.7692)=8×0.2308=1.8464cm
Now, sum all the calculated apparent depths:
D=1.3332+1.7142+1.8464=4.8938cm
Rounding to two decimal places, the apparent depth is approximately:
D≈4.89cm
The apparent position of the scratch on the outer surface of the bottom of the glass slab is approximately 4.89 cm.
Topper's Solved these Questions
- BOOK - SL ARORACHAPTER - RAY OPTICSEXERCISE - TYPE E10videos
RAY OPTICS
- BOOK - SL ARORACHAPTER - RAY OPTICSEXERCISE - TYPE G19videos
RAY OPTICS
- BOOK - SL ARORACHAPTER - RAY OPTICSEXERCISE - TYPE C17videos
RAY OPTICS
- BOOK - SL ARORACHAPTER - OPTICAL INSTRUMENTSEXERCISE - Problems for self practice35videos
OPTICAL INSTRUMENTS
- BOOK - SL ARORACHAPTER - SOLIDS AND SEMICONDUCTOR DEVICESEXERCISE - PROBLEM_TYPE9videos
SOLIDS AND SEMICONDUCTOR DEVICES
Similar Questions
A jar contains two immiscible liquids A and B with a coin at its bottom. The depths of liquid A and liquid B are 6 cm and 8 cm, respectively. If the apparent depth of the coin is 9 cm and refractive index of liquid A is 1.5, what is the refractive index of liquid B?
View SolutionA man observes a coin placed at the bottom of a beaker which contains two immiscible liquids of refractive indices 1.2 and 1.4 as shown in the figure. Find the depth of the coin below the surface, as observed from above.
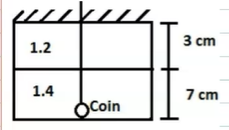 View Solution
View Solution
Knowledge Check
- Question 1 - Select One
The time taken by a ray of light to travel through a glass slab of thickness 2 cm and refractive index 1.5 will be
A10^(-8) sB10−10sC2×10−8sD10−12s - Question 2 - Select One
A glass slab of thickness 12 cm is placed on a table. The level surface of the slab has a black spot. At what depth from the upper surface will the spot appear when viewed from above?
(Refractive index of glass = 1.5)A2 mmB4 mmC6 mmD8 mm - Question 3 - Select One
A beaker is filled with two immiscible transparent liquids of refractive indices μ1 and μ2 having thickness of layers d1 and d2 respectively. The apparent depth of the bottom of the beaker is
A(d1×d2d1+d2)((1μ1)+(1μ2))B(μ1×μ2)(d1+d2)C((d1μ2)+(d2μ1))D⎛⎜⎝d1μ1d2μ2⎞⎟⎠
A small filament is at the centre of a hollow glass sphere of inner and outer radii 8 cm and 9 cm respectively. The refractive index of glass is 1.50. Calculate the position of the image of the filament when viewed from outside the sphere.
View SolutionA thick rectangular glass slab is kept inside a water trough. A ray of light passing through water is incident on the glass slab at an angle of 45∘. Calculate the angle of refraction, if refractive indices of water and glass are 1.33 and 1.45 respectively.
View SolutionA vessel has a 3-cm-thick bottom made of glass (μ=1.5). The vessel contains water (μ=1.33) up to a depth of 6 cm and oil (μ=1.30) up to a depth of another 7 cm. Find the apparent depth of vessel when viewed from above.
View SolutionTwo immiscible liquids of refractive index √2 and 2√2 are filled with equal height h in a vessel. Then apparent depth of bottom surface of the container given that outside medium is air
View SolutionA glass beaker is filled with water up to 5cm. It is kept on top of a 2cm thick glass slab. When a coin at the bottom of the glass slab is viewed at the normal incidence from above the beaker, its apparent depth from the water surface is dcm. Value of d is close (the refractive index of water and glass are 1.33 and 1.50,respectively)
View Solution

