A particle moving with a uniform acceleration along a straight line covers distances a and b in successive intervals of p and q second. The acceleration of the particle is
pq(p+q)2(bp−aq)
2(aq−bp)pq(p−q)
bp−aqpq(p−q)
2(bp−aq)pq(p+q)
To find the acceleration of a particle moving with uniform acceleration that covers distances a and b in successive intervals of p and q seconds, we can use the equations of motion. Here’s a step-by-step solution:
Step 1: Write the equations of motion for the distances covered.
1. For the first distance a covered in time p:
\(
a = u p + \frac{1}{2} A p^2 \quad \text{(1)}
\)
where \( u \) is the initial velocity and \( A \) is the acceleration.
2. For the second distance b covered in time q:
The velocity after time p is:
\(
v = u + A p \quad \text{(2)}
\)
Now, using this velocity as the initial velocity for the next interval:
\(
b = v q + \frac{1}{2} A q^2
\)
Substituting \( v \) from equation (2):
\(
b = (u + A p) q + \frac{1}{2} A q^2 \quad \text{(3)}
\)
Step 2: Substitute and rearrange the equations.
From equation (1):
\(
u p = a - \frac{1}{2} A p^2
\)
Substituting this into equation (3):
\(
b = \left(a - \frac{1}{2} A p^2 + A p\right) q + \frac{1}{2} A q^2
\)
Expanding this gives:
\(
b = aq - \frac{1}{2} A p^2 q + A p q + \frac{1}{2} A q^2
\)
Step 3: Rearranging to isolate terms involving \( A \).
Rearranging gives:
\(
b - aq = A p q - \frac{1}{2} A p^2 q + \frac{1}{2} A q^2
\)
Factoring out \( A \):
\(
b - aq = A \left( p q - \frac{1}{2} p^2 q + \frac{1}{2} q^2 \right)
\)
Step 4: Solve for acceleration \( A \).
Now, rearranging to find \( A \):
\(
A = \frac{b - aq}{pq - \frac{1}{2} p^2 q + \frac{1}{2} q^2}
\)
This can be simplified further to:
\(
A = \frac{2(b - aq)}{pq(p + q)}
\)
Final Result:
The acceleration \( A \) of the particle is given by:
\(
A = \frac{2(b p - a q)}{pq(p + q)}
\)
\(
a = u p + \frac{1}{2} A p^2 \quad \text{(1)}
\)
where \( u \) is the initial velocity and \( A \) is the acceleration.
The velocity after time p is:
\(
v = u + A p \quad \text{(2)}
\)
Now, using this velocity as the initial velocity for the next interval:
\(
b = v q + \frac{1}{2} A q^2
\)
Substituting \( v \) from equation (2):
\(
b = (u + A p) q + \frac{1}{2} A q^2 \quad \text{(3)}
\)
\(
u p = a - \frac{1}{2} A p^2
\)
Substituting this into equation (3):
\(
b = \left(a - \frac{1}{2} A p^2 + A p\right) q + \frac{1}{2} A q^2
\)
Expanding this gives:
\(
b = aq - \frac{1}{2} A p^2 q + A p q + \frac{1}{2} A q^2
\)
\(
b - aq = A p q - \frac{1}{2} A p^2 q + \frac{1}{2} A q^2
\)
Factoring out \( A \):
\(
b - aq = A \left( p q - \frac{1}{2} p^2 q + \frac{1}{2} q^2 \right)
\)
\(
A = \frac{b - aq}{pq - \frac{1}{2} p^2 q + \frac{1}{2} q^2}
\)
This can be simplified further to:
\(
A = \frac{2(b - aq)}{pq(p + q)}
\)
The acceleration \( A \) of the particle is given by:
\(
A = \frac{2(b p - a q)}{pq(p + q)}
\)
Topper's Solved these Questions
Similar Questions
A car starting from rest and moving along a straight path with uniform acceleration covers distances p and q in the first two successive equal intervals of time. Find the ratio of p to q.
View SolutionA car starting from rest and moving along a straight path with uniform acceleration covers distances p and q in the first two successive equal intervals of time. Find the ratio of p to q.
View Solution
Knowledge Check
A particle is moving along a straight line.
AIf the acceleration of the particle is more than that of another object, the particle is moving faster.BIf the acceleration is positive, the speed must be increasing.CIf the acceleration and the velocity have the same sign, the speed must be increasing.DIf the acceleration is zero, the velocity is constant but the speed may be changing.A body moving in a straight line with constant acceleration covers distances a and b in successive equal time interval of t. The acceleration of the body is
Aa+bt2Bb−at2C2b−a2t2Db−2at2The displacement of a particle moving in a straight line, is given by s=2t2+2t+4 where s is in metres and t in seconds. The acceleration of the particle is.
A2m/s2B4m/s2C6m/s2D8m/s2
A particle moves with uniform acceleration along a straight line from rest. The percentage increase in displacement during sixth second compared to that in fifth second is about
View SolutionA particle moving with uniform acceleration from A to B along a straight line has velcities v1 and v2 at A and B respectively. If C is the mid-point between A and B then determine the velocity of the particle at C .
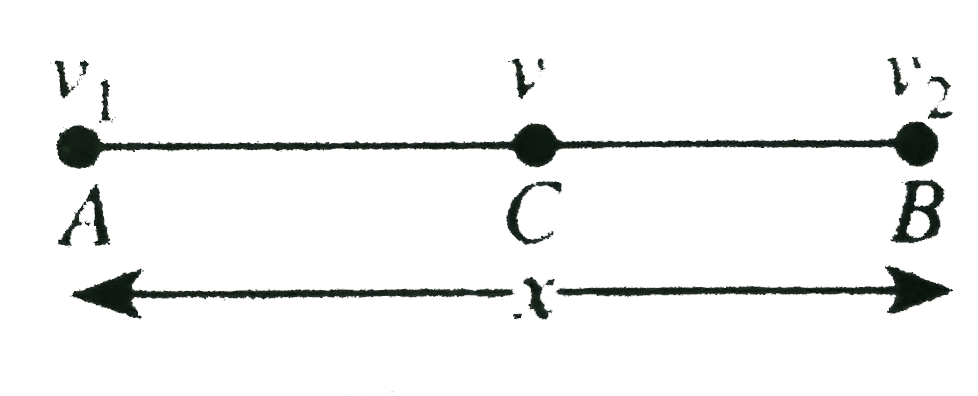 .
.
View SolutionA particle moving with uniform retardation covers distances 18 m, 14 m and 10 m in successive seconds. It comes to rest after travelling a further distance of
View SolutionA particle moves in a straight line with a constant acceleration. It passing through a distance 135 m in t second. The value of t (in second) is
View SolutionThe speed v of a particle moving along a straight line is given by a+bv2=x2 where x is its distance from the origin. The acceleration of the particle is
View Solution
