
A certain cookie company sells several varieties of chocolate cookies, each with an add ingredient. The company sells the different varieties in differently sized boxes. The number of cookies per box and the profit per box for the different varieties is shown in the table above. The relationship between the number of cookies per box and the profit, in dollars, that the company makes per box can be represented by a linear function. Which of the following functions correctly represents the relationship ?
p(n)=0.11n−0.25
p(n)=0.1n−0.35
p(n)=0.09n−0.95
p(n)=0.8n−0.5
The correct Answer is:D
Another approach would be to use two of the pairs of data points from the table to calculate a slope, for example, using the "pecans" and "macadamias" rows would yield 1.10−0.4620−12=0.648=0.08. Because only one answer has a slope of 0.08, you can pick (D).
Finally, you could backslove. Plug any one of the rows of data from the table into all four answer choices. The second row has the easiest numbers to work with, so use those. You are checking which equation will produce a profit of $1.10 per box given 20 cookies per box:
A) 0.11(20)−0.25=1.95≠1.10
B) 0.1(20))−0.35=1.65≠1.10
C) 0.09(20)−0.45=1.35≠1.10
(D) 0.08(20)−0.5=1.10
Again, (D) is correct.
Topper's Solved these Questions
- FUNCTIONS BOOK - KAPLANCHAPTER - FUNCTIONS EXERCISE - GRAPHS OF FUNCTIONS
1 Videos
- EXPONENTS, RADICALS, POLYNOMIALS, AND RATIONAL EXPRESSIONSBOOK - KAPLANCHAPTER - EXPONENTS, RADICALS, POLYNOMIALS, AND RATIONAL EXPRESSIONSEXERCISE - RATIONAL EXPRESSIONS AND EQUATIONS
1 Videos
- GEOMETRY BOOK - KAPLANCHAPTER - GEOMETRY EXERCISE - THREE-DIMENSIONAL FIGURES
1 Videos
Similar Questions
There are 45 cookies in a box. 27 of them have raisins while the rest are plain. What is the ratio of the number of plain cookies to that of raisin cookies in the box?
View SolutionSix different balls are put in three different boxes, no box being empty. The probability of putting balls in the boxes in equal numbers is
View Solution
Knowledge Check
In how many different ways can 6 identical balls be distributed to 4 different boxes , when each box can have any number of balls ?
A2048B1296C(24)2D4096The number of ways in which 6 different balls can be put in two boxes of different sizes so that no box is empty is
A64B62C60D30In how many different ways can 6 different balls be distributed to 4 different boxes, when each box can hold av number of ball?
A2048B1296C(24)2D4096
Six different balls are put in three different boxes, no box being empty. The probability of putting balls in the boxes in equal numbers is
View SolutionThe number of ways we can put 5 different balls in 5 different boxes such that at most three boxes is empty, is equal to
View SolutionJohn decided to treat his friends with some chocolates. On visiting a Hershey's outlet, he found that chocolates were sold in boxes. The boxes had different number of chocolates and the prices of the boxes were also different. The prices are shown in the table below.
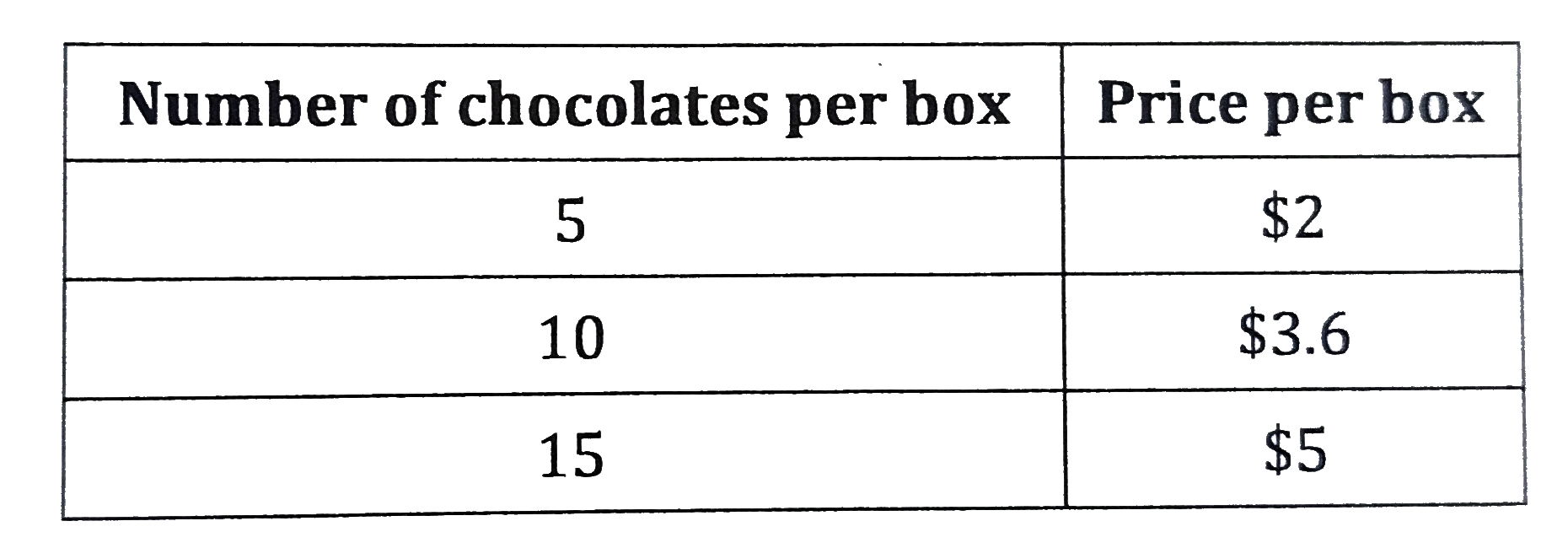
How much less does John spend if he busy 90 chocolates in boxes of 15 chocolates than if he buys the same in boxes of 10 chocolates ?
View SolutionA cookie store's weekly profit is a function of the number of cookies , c, that it sells . The equation approximating the weekly profit , in dollars , is
f( c)=0.60c-900
Which of the following is a false statement about the weekly profits ?
View SolutionIn how many different ways can 6 identical balls be distributed to 4 different boxes , when each box can have any number of balls ?
View Solution
