Find the surface density of electric charge at a place on the earth's surface, where the rate of fall of potential is 2.5 V.
2.0×10−9C/m2
2.21×10−9C/m2
3.36×10−9C/m2
3.5×10−9C/m2
To find the surface density of electric charge at a place on the Earth's surface where the rate of fall of potential is 2.5 V, we can follow these steps:
1. Understand the relationship between electric field (E) and potential (V):
The electric field (E) is related to the potential (V) by the equation:
E=−dVdr
where dV is the change in potential and dr is the change in distance.
2. Substitute the given rate of fall of potential:
Given that the rate of fall of potential is 2.5 V, we can express this as:
E=−dVdr=−2.5V/m
(assuming the distance dr is 1 meter for simplicity).
3. Use Gauss's law to relate electric field and surface charge density:
According to Gauss's law, the surface charge density (σ) is related to the electric field by the equation:
σ=ϵ0E
where ϵ0 is the permittivity of free space, approximately 8.85×10−12C/m2.
4. Calculate the surface charge density:
Substitute the value of E into the equation:
σ=ϵ0⋅E=(8.85×10−12C/m2)⋅(2.5V/m)
5. Perform the multiplication:
σ=8.85×10−12⋅2.5=2.2125×10−11C/m2
6. Round the answer:
Rounding to two decimal places, we get:
σ≈2.21×10−11C/m2
Final Answer:
The surface density of electric charge at that place is approximately:
σ≈2.21×10−11C/m2
The electric field (E) is related to the potential (V) by the equation:
E=−dVdr
where dV is the change in potential and dr is the change in distance.
Given that the rate of fall of potential is 2.5 V, we can express this as:
E=−dVdr=−2.5V/m
(assuming the distance dr is 1 meter for simplicity).
According to Gauss's law, the surface charge density (σ) is related to the electric field by the equation:
σ=ϵ0E
where ϵ0 is the permittivity of free space, approximately 8.85×10−12C/m2.
Substitute the value of E into the equation:
σ=ϵ0⋅E=(8.85×10−12C/m2)⋅(2.5V/m)
σ=8.85×10−12⋅2.5=2.2125×10−11C/m2
Rounding to two decimal places, we get:
σ≈2.21×10−11C/m2
The surface density of electric charge at that place is approximately:
σ≈2.21×10−11C/m2
Topper's Solved these Questions
- GAUSS LAW AND ELECTRIC POTENTIAL THEORYBOOK - BITSAT GUIDECHAPTER - GAUSS LAW AND ELECTRIC POTENTIAL THEORYEXERCISE - BITSTAT ARCHIVES
4 Videos
- FLUID MECHANICSBOOK - BITSAT GUIDECHAPTER - FLUID MECHANICSEXERCISE - BITSAT Achives
15 Videos
- MAGNETIC FIELDBOOK - BITSAT GUIDECHAPTER - MAGNETIC FIELDEXERCISE - All Questions
52 Videos
Similar Questions
Calculate the surface density of change at a place on the earth's surface where the rate of fall of potential is 250 volts per metre.
[Hint : rate of fall of potential =electric =E=σε0 by Gauss's theorm.]
View SolutionOn the basis of your understanding of the following paragraph and the related studied concepts.
An equipotential surface is a surface with a constant value of electric potential at all points on the surface. The component of electric field parallel to an equipotential surface is zero, as the potential does not change in this direction. Thus, the electric field is perpendicular to the equipotential surface at each point of the surface. Further, say the direction of electric field electrical potential gradually fall with distance.
If electric potential at origin point O be 100 V, then for electric field specified in part (b), draw equipotential surfaces corresponding to potentials 80 V, 60 V and 40 V respectively.
View Solution
Knowledge Check
A meteoroid that falls on the Earth's surface is called a _______ .
AMeteoriteBAsteroidsCMeteorsDOrionsThe surface density of charge on the earth's surface is 2.65×10−9C/m2. If the radius of the earth is 6400 km then the charge carried by the earth is
A1.363×106CB1.364×109CC3.364×106CD2.396×106CA body of mass m rises to a height h=R/5 from the earth's surface where R is earth's radius. If g is acceleration due to gravity at the earth's surface, the increase in potential energy is
AmghB45mghC56mghD67mgh
On the basis of your understanding of the following paragraph and the related studied concepts.
An equipotential surface is a surface with a constant value of electric potential at all points on the surface. The component of electric field parallel to an equipotential surface is zero, as the potential does not change in this direction. Thus, the electric field is perpendicular to the equipotential surface at each point of the surface. Further, say the direction of electric field electrical potential gradually fall with distance.
If a charge of 2 nC is taken from equipotential surface number 2 to 3, what is the amount of work done ?
View SolutionOn the basis of your understanding of the following paragraph and the related studied concepts.
An equipotential surface is a surface with a constant value of electric potential at all points on the surface. The component of electric field parallel to an equipotential surface is zero, as the potential does not change in this direction. Thus, the electric field is perpendicular to the equipotential surface at each point of the surface. Further, say the direction of electric field electrical potential gradually fall with distance.
Draw equipotential surfaces around an isolated positive point charge + q. Mark direction of electric field →E also.
View SolutionOn the basis of your understanding of the following paragraph and the related studied concepts.
An equipotential surface is a surface with a constant value of electric potential at all points on the surface. The component of electric field parallel to an equipotential surface is zero, as the potential does not change in this direction. Thus, the electric field is perpendicular to the equipotential surface at each point of the surface. Further, say the direction of electric field electrical potential gradually fall with distance.
Four equipotential surfaces are shown in the adjoining figure. Find the magnitude and direaction of the electric field.
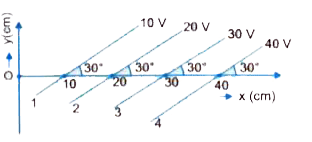
View SolutionOn the basis of your understanding of the following paragraph and the related studied concepts.
An equipotential surface is a surface with a constant value of electric potential at all points on the surface. The component of electric field parallel to an equipotential surface is zero, as the potential does not change in this direction. Thus, the electric field is perpendicular to the equipotential surface at each point of the surface. Further, say the direction of electric field electrical potential gradually fall with distance.
A uniform electric field →E=1000ˆiVm−1 exists in space at a given place. What is the shape of equipotential surfaces there ?
View SolutionA spherical charged conductor has σ as the surface density of charge. The electric field on its surface is E. If the radius of the sphere is doubled keeping the surface density of charge unchanged, what will be the electric field on the surface of the new sphere -
View Solution
