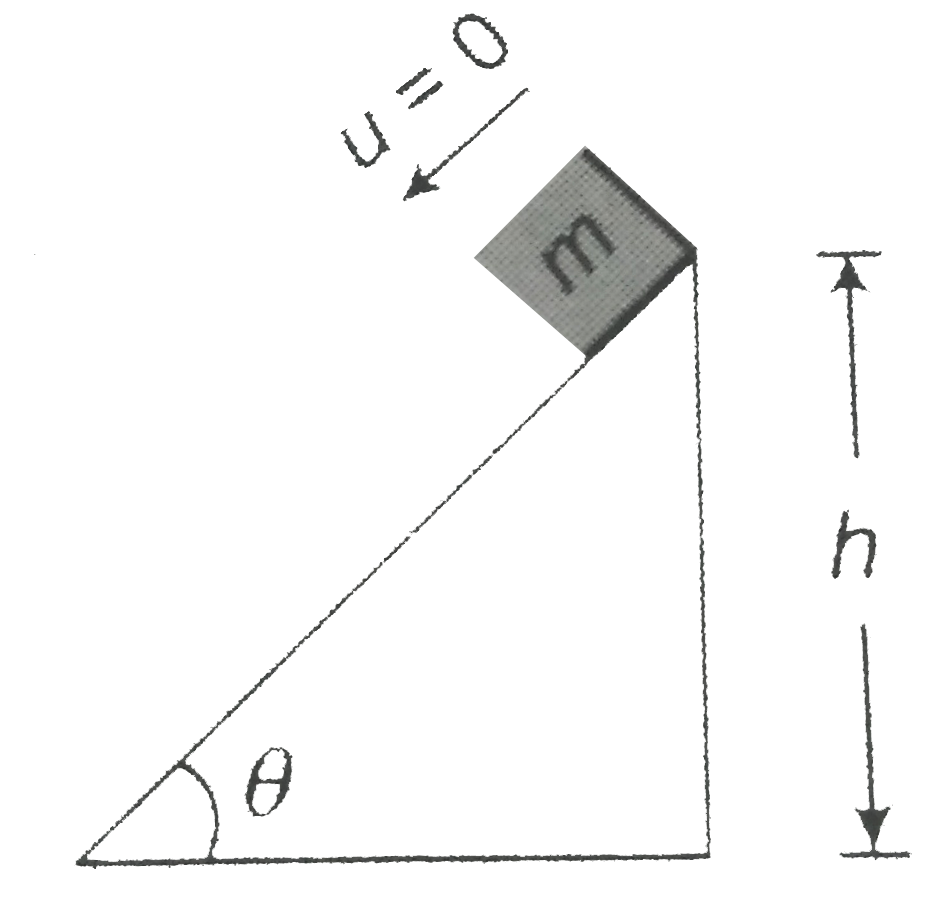
An object is placed on the surface of a smooth inclined plane of inclination $θ$ . It takes time t to reach the bottom. If the same object is allowed to slide down a rough inclined plane of inclination $θ$ , it takes time nt to reach the bottom where n is a number greater than 1. The coefficient of friction $μ$ is given by:-

$μ = tan θ (1 - \frac{1}{n^{2}})$

$μ = cot θ (1 - \frac{1}{n^{2}})$

$μ = tan θ \sqrt{1 - \frac{1}{n^{2}}}$

$μ = cot θ \sqrt{1 - \frac{1}{n^{2}}}$

Text Solution

Verified by Experts

The correct Answer is:A

On smooth inclined plane : Acceleration of the body =g sin $θ$
If s be the distance travelled , then
$s = \frac{1}{2} g sin θ \times t_{1}^{2}$ ….(i)
On rough inclined plane :
Acceleration , $a = \frac{m g sin θ - μ R}{m}$
or $a = \frac{m g sin θ - μ m g cos θ}{m}$
$= g sin θ - μ g cos θ$
$∴ s = \frac{1}{2} (g sin θ - μ g cos θ) t_{2}^{2}$ ...(ii)
From equations (i) and (ii) ,
$\frac{t_{2}^{2}}{t_{1}^{2}} = \frac{sin θ}{sin θ - μ cos θ}$
But $t_{2} = n t_{1}, ∴ n^{2} = \frac{sin θ}{sin θ - μ cos θ}$
or $μ = \frac{n^{2} - 1}{n^{2}} \times \frac{sin θ}{cos θ}$ or $μ = (1 - \frac{1}{n^{2}}) tan θ$

Updated on:21/07/2023

Class 12 PHYSICS DRILL TEST 6

Topper's Solved these Questions

Knowledge Check

Question 1 - Select One
An object is placed on the surface of a smooth inclined plane of inclination . It takes time t to reach the bottom of the inclined plane. If the same object is allowed to slide down rough inclined plane of same inclination , it takes time nt to reach the bottom where n is a number greater than 1. The coefficient of friction  is given by -
A $μ = tan θ [1 - \frac{1}{n^{2}}]$
B $μ = cot θ [1 - \frac{1}{n^{2}}]$
C $μ = tan θ {[1 - \frac{1}{n^{2}}]}^{1 / 2}$
D $μ = cot θ {[1 - \frac{1}{n^{2}}]}^{1 / 2}$
Question 2 - Select One
An object is placed on the surface of a smooth inclined plane of inclination . It takes time t to reach the bottom of the inclined plane. If the same object is allowed to slide down rough inclined plane of same inclination , it takes time nt to reach the bottom where n is a number greater than 1. The coefficient of friction  is given by -
A $μ = tan θ (1 - \frac{1}{n^{2}})$
B $μ = cot θ (1 - \frac{1}{n^{2}})$
C $μ = tan θ {(1 - \frac{1}{n^{2}})}^{1 / 2}$
D $μ = cot θ {(1 - \frac{1}{n^{2}})}^{1 / 2}$
Question 3 - Select One
A block moves down a smooth inclined plane of inclination $θ$ . Its velocity on reaching the bottom is v. If it slides down a rough inclined plane of some inclination, its velocity on reaching the bottom is v/n, where n is a number greater than 0. The coefficient of friction is given by -
A $μ = tan θ (1 - \frac{1}{n^{2}})$
B $μ = cot θ (1 - \frac{1}{n^{2}})$
C $μ = tan θ (1 + \frac{1}{n^{2}})$
D $μ = cot θ (1 + \frac{1}{n^{2}})$

NEET MAJOR TEST (COACHING)-DRILL TEST 6-PHYSICS

Home
Ask Doubt
Profile

The correct Answer is:A

Topper's Solved these Questions

Similar Questions

Knowledge Check

A block moves down a smooth inclined plane of inclination θ. Its velocity on reaching the bottom is v. If it slides down a rough inclined plane of some inclination, its velocity on reaching the bottom is v/n, where n is a number greater than 0. The coefficient of friction is given by -

NEET MAJOR TEST (COACHING)-DRILL TEST 6-PHYSICS

A block moves down a smooth inclined plane of inclination $θ$ . Its velocity on reaching the bottom is v. If it slides down a rough inclined plane of some inclination, its velocity on reaching the bottom is v/n, where n is a number greater than 0. The coefficient of friction is given by -