A balloon leaves the earth and rises at a uniform velocity. At the end of 2 min, an observer situated at 200m from the point the balloon was released, finds the regular elevation of the balloon to be 60∘. What is the speed in meters per second, to the nearest integer, of the balloon?
The correct Answer is:3
To find the speed of the balloon, we will follow these steps:
Step 1: Understand the Problem
We know that a balloon is rising at a uniform velocity, and after 2 minutes, the observer sees the balloon at an angle of elevation of 60∘ from a point that is 200 meters away horizontally from the release point.
Step 2: Set Up the Right Triangle
The situation can be visualized as a right triangle where:
- The height of the balloon (h) is the vertical side.
- The horizontal distance from the observer to the point of release is 200 meters (the base).
- The angle of elevation is 60∘.
Step 3: Use the Tangent Function
Using the tangent function, we can relate the height of the balloon to the horizontal distance:
tan(60∘)=heighthorizontal distance=h200
Since tan(60∘)=√3, we can write:
√3=h200
Step 4: Solve for Height (h)
Rearranging the equation to solve for h:
h=200⋅√3
Step 5: Calculate Height
Now, we can calculate the height:
h=200⋅√3≈200⋅1.732≈346.4 meters
Step 6: Convert Time to Seconds
The time taken for the balloon to rise is given as 2 minutes. We need to convert this into seconds:
2 minutes=2×60=120 seconds
Step 7: Calculate Speed
Speed is defined as distance traveled over time taken. Therefore, the speed of the balloon can be calculated as:
Speed=HeightTime=346.4120≈2.8867 m/s
Step 8: Round to the Nearest Integer
Finally, rounding 2.8867 to the nearest integer gives us:
Speed≈3 m/s
Final Answer
The speed of the balloon is approximately 3 m/s.
---
We know that a balloon is rising at a uniform velocity, and after 2 minutes, the observer sees the balloon at an angle of elevation of 60∘ from a point that is 200 meters away horizontally from the release point.
The situation can be visualized as a right triangle where:
- The height of the balloon (h) is the vertical side.
- The horizontal distance from the observer to the point of release is 200 meters (the base).
- The angle of elevation is 60∘.
Using the tangent function, we can relate the height of the balloon to the horizontal distance:
tan(60∘)=heighthorizontal distance=h200
Since tan(60∘)=√3, we can write:
√3=h200
Rearranging the equation to solve for h:
h=200⋅√3
Now, we can calculate the height:
h=200⋅√3≈200⋅1.732≈346.4 meters
The time taken for the balloon to rise is given as 2 minutes. We need to convert this into seconds:
2 minutes=2×60=120 seconds
Speed is defined as distance traveled over time taken. Therefore, the speed of the balloon can be calculated as:
Speed=HeightTime=346.4120≈2.8867 m/s
Finally, rounding 2.8867 to the nearest integer gives us:
Speed≈3 m/s
The speed of the balloon is approximately 3 m/s.
Topper's Solved these Questions
- BOOK - ENGLISH SATCHAPTER - ADDITIONAL TOPICS IN MATHEXERCISE - Grib-In32videos
ADDITIONAL TOPICS IN MATH
- BOOK - ENGLISH SATCHAPTER - ADDITIONAL TOPICS IN MATH EXERCISE - PRACTICE TEST24videos
ADDITIONAL TOPICS IN MATH
- BOOK - ENGLISH SATCHAPTER - COMPLEX NUMBERSEXERCISE - EXERCISE6videos
COMPLEX NUMBERS
Similar Questions
A car is being driven, in a straight line and at a uniform speed, towards the base of a vertical tower of height 30 feet. The top of the tower is observed from the car and, in the process, the angle of elevation changes from 45∘ at B to 60∘ at A. What is the distance, in feet, to the nearest integer, between the points A and B?
View SolutionA stationary balloon is observed from three points A, B and C on the plane groundand is found that its angle of elevation from each point is 60∘. If ∠ABC=30∘ and AC = 5 meters, then the height of the balloon from the ground is
View SolutionA balloon rises with uniform velocity of 10ms−1. When the balloon is at a height of 75 m if a stone is dropped from it the time taken by it to reach the ground is
View SolutionAn observer having a gun observes a remotely controlled balloon. When he first noticed the balloon, it was at an altitude of 800m and moving vertically upward at a constant velocity of 5m/s. The horizontal displacement of balloon from the observer is 1600m. Shells fired from the gun have an initial velocity of 400m/s at a fixed angle θ(sinθ=3/5and cosθ=4/5). The observer having gun waits ( for some time after observing the balloon ) and fires so as to destroy the balloon. Assume g=10m/s2. Neglect air resistance.
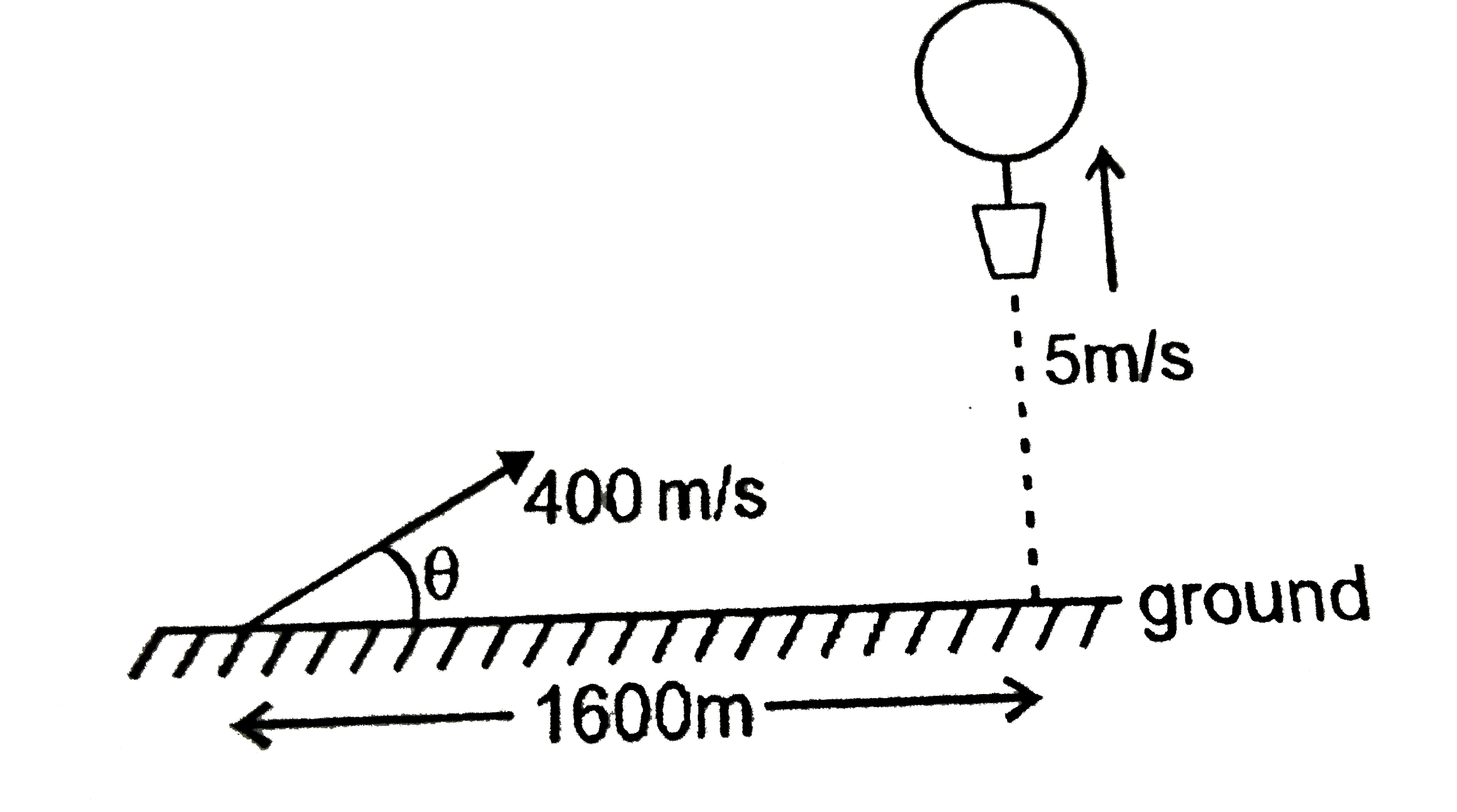
The flight time of the shell before it strikes the balloon isView SolutionA 1.2 m tall girl spots a balloon moving with the wind in a horizontal line at a height of 88.2 m from the ground. The angle of elevation of the balloon from the eyes of the girl at any instant is 60° . After some time, the angle of elevation reduces to 30°.Find the distance travelled by the ballon during the interval
View SolutionA balloon is rising vertically upwards. An an instant, an observation on the ground, whose distance from the balloon is 100 meters, sees the balloon at an angle of elevation of 30∘. If the balloon rises further vertically to a point where the angle of elevation as seen by the observer is 45∘, then its height (in meters) from the ground is
(Take √3=1.73)View SolutionA balloon starts rising from the earth's surface. The ascension rate is constant and equal to v0. Due to the wind. The balloon gathers the horizontal velocity component vx=ky, where k is a constnat and y is the height of ascent. Find how the following quantities depednd on the height of ascent.
(a) the horizontal drift of the balloon x (y)
(b) the total tangential and normal accelrations of the balloon.View SolutionA stone is dropped from a balloon going up with a uniform velocity of 5.0 m/s. If the balloon was 50 m high when the stone was dropped, find its height when the stone hits the ground. Take g=10ms2.
View SolutionAn arrow is launched upward with an initial speed of 70m/s ( meters per second). The equation v2=v20−2gh describes the motion of the arrow, where v0 is the initial speed of the arrow, v is the speed of the arrow as it is moving up in the air, h is the height of the arrow above the ground, t is the time elapsed since the arrow was projected upward, and g is the acceleration due to gravity ( approximately 9.8m/s2). What is the maximum height from the ground the arrow will rise to the nearest meter?
View SolutionA hydrogen balloon is flying eastward with speed 12ms−1 , when wind starts blowing from north to south with speed 5ms−1 what is the resultant velocity of balloon ?
View Solution

