In the standard (x,y) coordinate plane, what is the slope of the line 3x+8y=5?
−3
−38
35
3
The correct Answer is:B
To find the slope of the line given by the equation 3x+8y=5, we can follow these steps:
Step 1: Rewrite the equation in slope-intercept form
The slope-intercept form of a line is given by the equation y=mx+c, where m is the slope and c is the y-intercept. We need to isolate y in the equation 3x+8y=5.
Step 2: Isolate y
Start by moving 3x to the right side of the equation:
8y=5−3x
Step 3: Divide by the coefficient of y
Next, divide every term by 8 to solve for y:
y=−38x+58
Step 4: Identify the slope
Now that we have the equation in the form y=mx+c, we can identify the slope m. From the equation, we see that:
m=−38
Conclusion
Thus, the slope of the line 3x+8y=5 is −38.
---
The slope-intercept form of a line is given by the equation y=mx+c, where m is the slope and c is the y-intercept. We need to isolate y in the equation 3x+8y=5.
Start by moving 3x to the right side of the equation:
8y=5−3x
Next, divide every term by 8 to solve for y:
y=−38x+58
Now that we have the equation in the form y=mx+c, we can identify the slope m. From the equation, we see that:
m=−38
Thus, the slope of the line 3x+8y=5 is −38.
Topper's Solved these Questions
Similar Questions
Knowledge Check
In the standard (x, y) coordinate plane, what is the slope of the line segement joining the points (3, -5) and (7, 2) ?
A−74B−34C34D74Points (2,-2) and ((3,10) lie on the same line in the standed (x,y) coordinate plane . What is the slope of this line ?
A12B8C112D−8The slope of a line in the standard (x,y) coordinate plane is 4. What is the slope of a line perpendicular to that line ?
A4B14C−14D−1
Which of the following values is the x-coordinate of the point in the standard (x,y) coordinate plane where the graph of the line y = 7 intersects the graph of the function y=1n(x−2)+3?
View SolutionThe curve y=0.005x2−2x+200 for 0≤x≤200 and the line segment from F(0,200) to G(200,0) are shown in the standard (x,y) coordinate plane below.
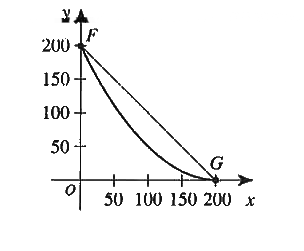
What is the y-coordinate for the point on the curve with x-coordinate 20?
View SolutionWhich of the following ordered pairs in the standard (x,y) coordinate plane satisfies the system of inequalities below?
x>2
y>0
x+y<5
View SolutionWhat is the y-intercept of the line in the standard (x,y) coordinate plane that goes through the points (-3,6) and (3,2) ?
View SolutionFor the rectangle shown in the standard ( x, y) Coordinate plane below , what are the coordinate of the unlabeled vertex ?
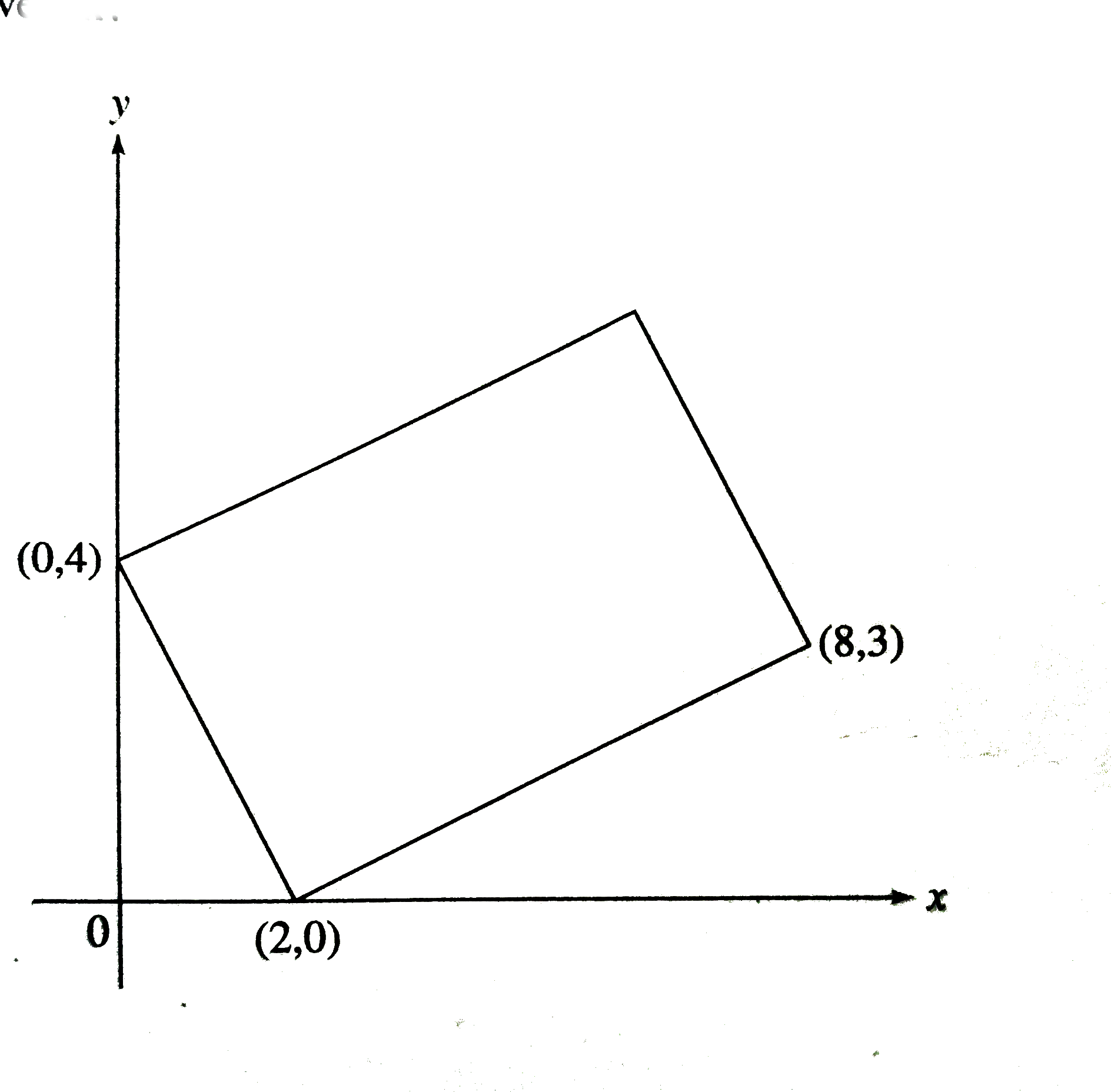
View Solution