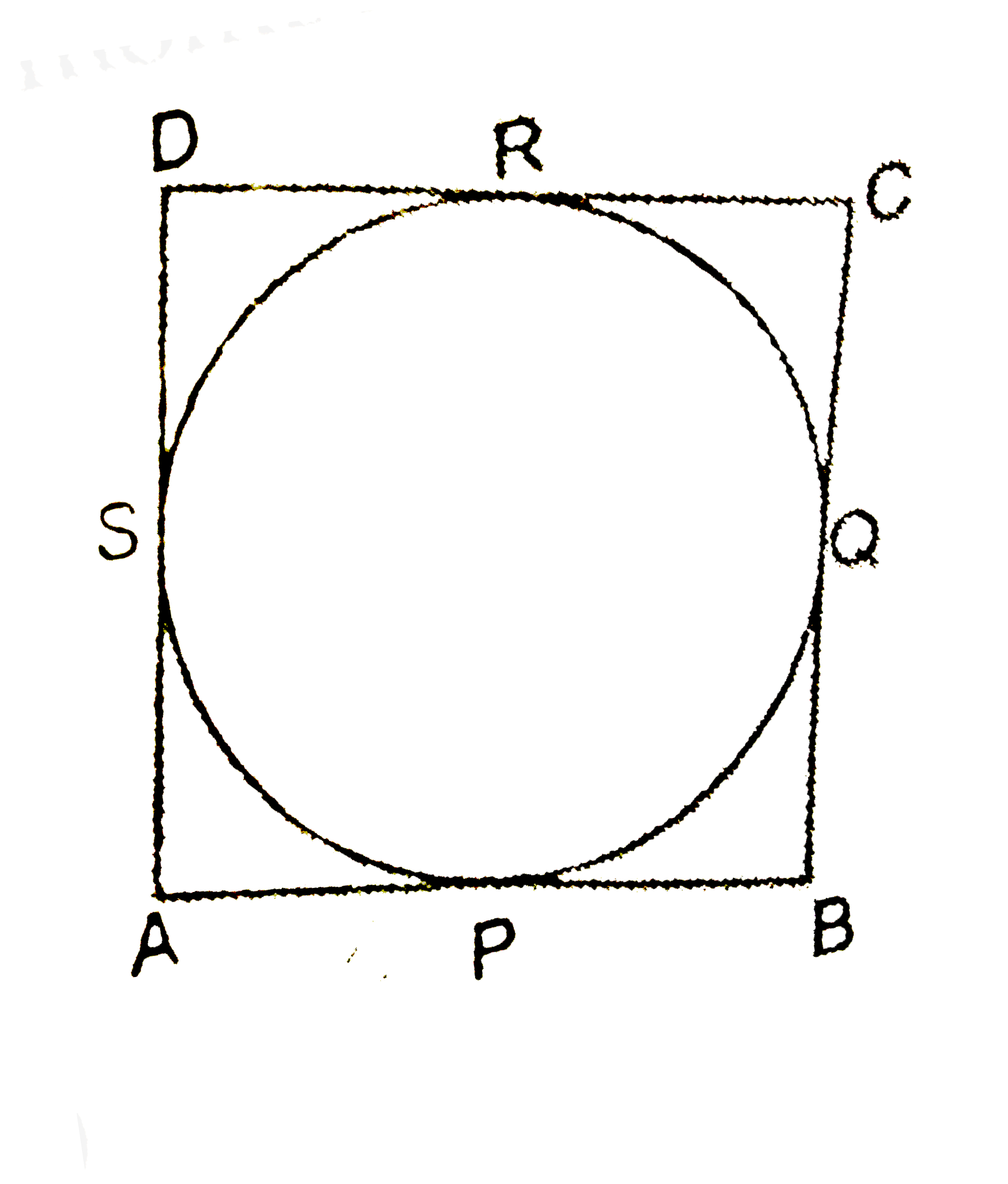
Prove that the parallelogram circumscribing a circle is a rhombus.
Step by step video solution for Prove that the parallelogram circumscribing a circle is a rhombus. by Maths experts to help you in doubts & scoring excellent marks in Class 10 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
Knowledge Check
Assertion(A) If two tangents are drawn to a circle from an external point then they subtend equal angles at the centre.
Reason (R ) A parallelogram circumscribing a circle is a rhombusABoth Assertion (A) and Reason (R ) are true and Reason (R ) is a correct explanation of Asseration (A).BBoth Assertion (A) and Reason (R ) are true but Reason (R ) is not a correct explanation of Asseration (A).CAssertion(A) is true and Reason (R ) is false.DAssertion(A) is false and Reason (R ) is true.
If a parallelogram circumscribes a circle then prove that it must be a rhombus.
View SolutionProve that opposite sides of a quadrilateral circumscribing a circle subtend supplementary angles at the centre of the circle.
View SolutionProve that opposite sides of a quadrilateral circumscribing a circle subtend supplementary angles at the centre of the circle.
View SolutionProve that opposite sides of a quadrilateral circumscribing a circle, subtend supplementary angles at the centre of a circle.
View SolutionRhombus a parallelogram having all sides equal is called a rhombus.
View SolutionRhombus a parallelogram having all sides equal is called a rhombus.
View SolutionProve that the centre of the circle circumscribing the cyclic rectangle ABCD is the point of intersection of its diagonals.
View Solution
