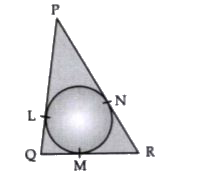
A circle is inscribed in a triangle PQR with PQ = 10 cm, QR = 8 cm and PR = 12 cm. Find the lengths QM, RN and PL.
To find the lengths QM, RN, and PL in triangle PQR with the given side lengths, we can use the property of the incircle of a triangle. The segments created by the points where the incircle touches the sides of the triangle can be expressed in terms of the triangle's sides.
1. Identify the sides of the triangle:
- Let PQ=a=10cm
- Let QR=b=8cm
- Let PR=c=12cm
2. Calculate the semi-perimeter (s):
The semi-perimeter s of the triangle is given by:
s=a+b+c2=10+8+122=302=15cm
3. Use the semi-perimeter to find the lengths of the segments:
The lengths of the segments from the vertices to the points where the incircle touches the sides are given by:
- QM=s−a
- RN=s−b
- PL=s−c
4. Calculate each segment:
- For QM:
QM=s−a=15−10=5cm
- For RN:
RN=s−b=15−8=7cm
- For PL:
PL=s−c=15−12=3cm
5. Final lengths:
- QM=5cm
- RN=7cm
- PL=3cm
Summary of Results:
- QM=5cm
- RN=7cm
- PL=3cm
- Let PQ=a=10cm
- Let QR=b=8cm
- Let PR=c=12cm
The semi-perimeter s of the triangle is given by:
s=a+b+c2=10+8+122=302=15cm
The lengths of the segments from the vertices to the points where the incircle touches the sides are given by:
- QM=s−a
- RN=s−b
- PL=s−c
- For QM:
QM=s−a=15−10=5cm
- For RN:
RN=s−b=15−8=7cm
- For PL:
PL=s−c=15−12=3cm
- QM=5cm
- RN=7cm
- PL=3cm
- QM=5cm
- RN=7cm
- PL=3cm
Topper's Solved these Questions
Similar Questions
Knowledge Check
In a triangle PQR, PQ = 20 cm and PR = 6 cm, the side QR is :
Aequal to 14 cmBless than 14 cmCgreater than 14 cmDnone of theseIn a triangle PQR, PQ=20 cm and PR =6 cm, the side QR is :
Aequal to 14 cmBless than 14 cmCgreater than 14 cmDnone of the above
Construct a triangle PQR such that PQ=6 cm, QR=7cm and PR=4.5cm.
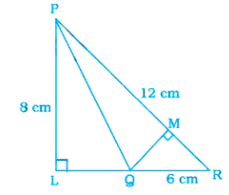
View SolutionIn Fig. 9.21, if PR = 12 cm, QR = 6 cm and PL = 8 cm, then QM is
View SolutionConstruct a triangle PQR in which QR = 6cm, ∠Q=60∘ and PR – PQ = 2cm.
View SolutionIn a triangle PQR, right angled at Q. PQ=5 cm, QR=12 cm. Find the length of QM , if M is the mid point of PR .
View SolutionConstruct a parallelogram PQRS, when PQ =3.7cm, QR =2.3 cm and PR=4.8 cm.
View SolutionIn △PQR,PQ=10 cm , QR =12 , PR =8 cm . Find out the greatest and the smallest angle of the triangle .
View Solution