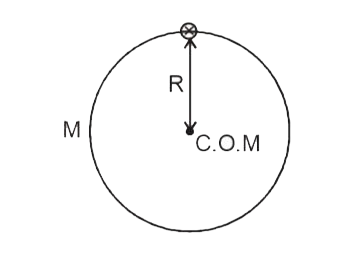
A ring is suspended at a point on its rim and it behaves as a second's pendulum when it oscillates such that its centre move in its own plane. The radius of the ring would be (g = $π^{2})$

Video Solution

Text Solution

Verified by Experts

Time period of second pendulum T = 2 cm.
$T = 2 π \sqrt{\frac{I}{M g d}}$
Moment of inertia with respect to axis O
$I = M R^{2} + M R^{2} = 2 M R^{2}$
the distance between centre of mass and the axis O
d= R
$2 = 2 π \sqrt{\frac{2 M R^{2}}{M g R}} \Rightarrow R = 0.5 m$

|

Updated on:21/07/2023

Class 12 PHYSICS SIMPLE HARONIC MOTION

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
A ring of diameter $2 m$ oscillates as a compound pendulum about a horizontal axis passing through a point at its rim. It oscillates such that its centre move in a plane which is perpendicular to the plane of the ring. The equibvalent length of the simple pendulum is
A $2 m$
B $4 m$
C $1.5 m$
D $3 m$
Question 2 - Select One

A uniform semicurcular ring having mass m and radius $r$ is hanging at one of its ends freely as shown if Fig. The ring is slightly disturbed so the it oscillates in its own plane. The time period of oscillation of the ring is
A $2 π \sqrt{\frac{r}{g (1 + \frac{1}{π^{2}})}}$
B $2 π \sqrt{\frac{r}{{g (1 + \frac{4}{π^{2}})}^{\frac{1}{2}}}}$
C $2 π \sqrt{\frac{r}{{g (1 - \frac{2}{π^{2}})}^{\frac{1}{2}}}}$
D $2 π \sqrt{\frac{2 r}{{g (1 + \frac{4}{π})}^{\frac{1}{2}}}}$
Question 3 - Select One
A second's pendulum is mounted in a rocket. Its period of oscillation decreases when the rocket
AMoves up with uniform accelertion
BMoves up with a uniform velocity
CComes down with uniform acceleration
DMoves round the earth in a geosterationary orbit.

MOTION-SIMPLE HARONIC MOTION -EXERCISE -4 (Leve-II) [ JEE 2011]

Home
Ask Doubt
Profile