A musical instrument is made using four different metal strings, 1, 2, 3 and 4 with mass per unit length
μ,2μ,3μand4μ respectively. The instrument is played by vibrating the strings by varying the free length in between the range
L0and2L0. It is found that in string-1
(μ) at free length L0 and tension T0 the fundamental mode frequency is f0 .
List-I gives the above four strings while list-II lists the magnitude of some quantity.
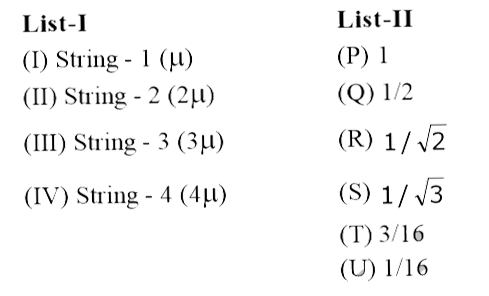
If the tension in each string is T0, the correct match for the highest fundamental frequency in f0 units will be -
I,Q,II-S,III-R,IV-P
I-P,II-R,III-S,IV-Q
I-P,II-Q,III-T,IV-S
I-Q,II-P,III-R,IV-T
The correct Answer is:B
Step by step video & image solution for A musical instrument is made using four different metal strings, 1, 2, 3 and 4 with mass per unit length mu,2mu,3mu and 4mu respectively. The instrument is played by vibrating the strings by varying the free length in between the range L_0 and 2L_0. It is found that in string-1 (mu) at free length L_0 and tension T_0 the fundamental mode frequency is f_0 . List-I gives the above four strings while list-II lists the magnitude of some quantity. If the tension in each string is T_0, the correct match for the highest fundamental frequency in f_0 units will be - by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- WAVESBOOK - MOTIONCHAPTER - WAVESEXERCISE - EXERCISE -4 (Level -I) (previous year JEE Main)
8 Videos
- WAVE OPTICS BOOK - MOTIONCHAPTER - WAVE OPTICS EXERCISE - EXERCISE - 4 (Level - II) Previous Year | JEE Main
16 Videos
- WORK, POWER & ENERGY BOOK - MOTIONCHAPTER - WORK, POWER & ENERGY EXERCISE - Exercise - 3 Section-B
22 Videos
Similar Questions
A musical instrument is made using four different metal strings, 1, 2, 3 and 4 with mass per unit length
μ,2μ,3μand4μ respectively. The instrument is played by vibrating the strings by varying the free length in between the range
L0and2L0. It is found that in string-1
(μ) at free length L0 and tension T0 the fundamental mode frequency is f0 .
List-I gives the above four strings while list-II lists the magnitude of some quantity.
The length of the strings 1,2,3 and 4 are kept fixed
at L0,3L02,5L04and7L04, respectively . S trings
1,2,3 and 4 are vibrated at their 1st,3rd,5thand14th harmonics, respectively such that all the strings have same frequency. The correct match for the tension in the four strings in the units of T0 will be -
View SolutionThe frequency of vibration of string depends on the length L between the nodes, the tension F in the string and its mass per unit length m. Guess the expression for its frequency from dimensional analysis.
View Solution
Knowledge Check
A compsits wire fixed at both ends consists of two uniform parts having length l and 4l and mass per unit lengths μand4μ respectively. Then in fundamental mode of vibration, wavelength in heavy wire, keeping junction as node, is
Al4Bl2ClDNone of theseWhen a string is divided into three segments of length l1,l2 and l3 the fundamental frequencies of these three segments are f1,f2 and f3 respectively. The original fundamental frequency f of the string is
A1v=1v1+1v2+1v3B1√v=1√v1+1√v2+1√v3C√v=√v1+√v2+√v3Dv=v1+v2+v3The linear mass density μ of the string is (where, mass of the string = m, length, of the string g = L)
AmLBmLCmL2DmL3
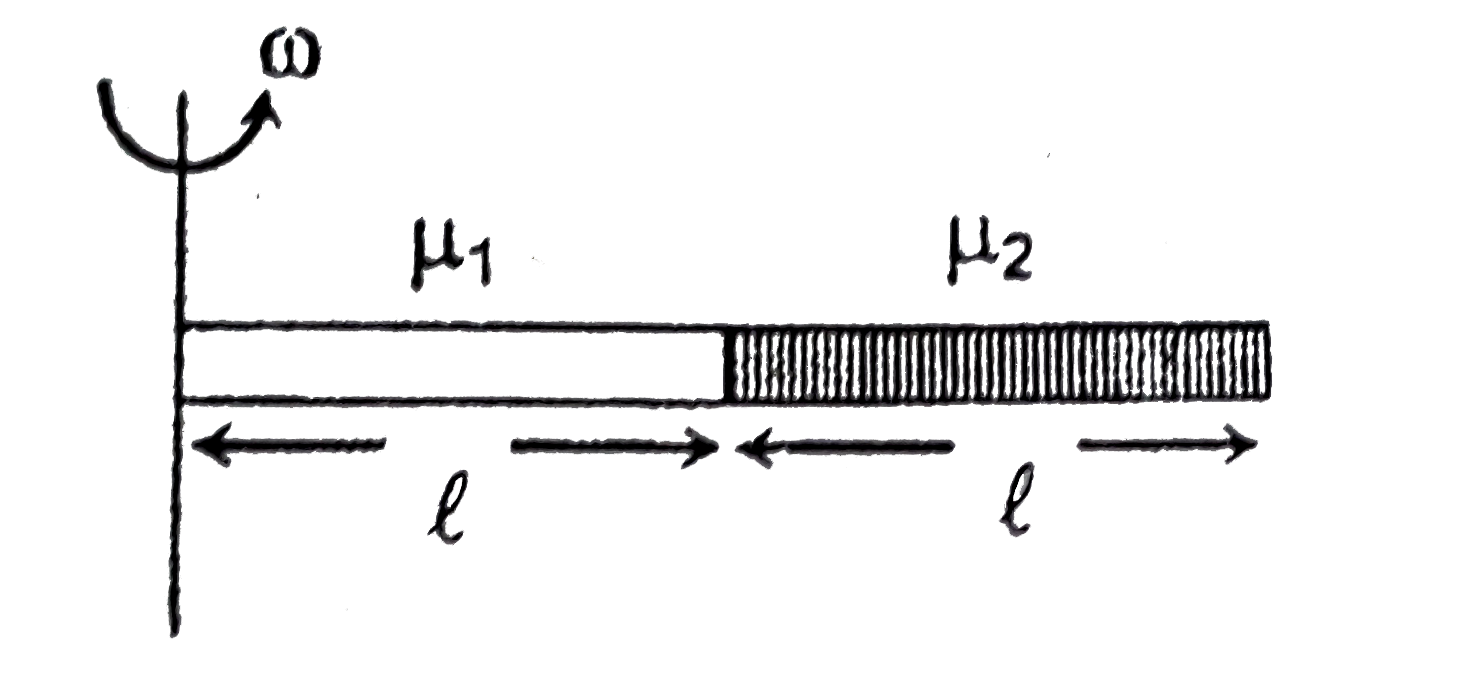
Two thin rods of same length l but of different uniform mass per unit length μ1 and μ2 respectively are joined together. The system is ortated on smooth horizontal plane as shown in figure. The tension at the joint will be
View SolutionWhen a stretched string of length L vibrating in a particular mode, the distance between two nodes on the string is l. The sound produced in this mode of vibration constitutes the nth overtone of the fundamental frequency of the string.
View SolutionString-1 is connected with string-2 The mass per unit length in string-1 is μ1 and mass per unit length in string-2 is 4μ1. The tension in the strings is T .A travelling wave is coming from the left .What fraction of the energy in the incident wave goes into string-2?
View SolutionWhen a string is divided into three segments of length l1,l2 and l3 the fundamental frequencies of these three segments are f1,f2 and f3 respectively. The original fundamental frequency f of the string is
View SolutionThe figure represents two snaps of a travelling wave on a string of mass per unit length μ=0.25kg/m. The first snap is taken at t=0 and the second is taken at t=0.05s.
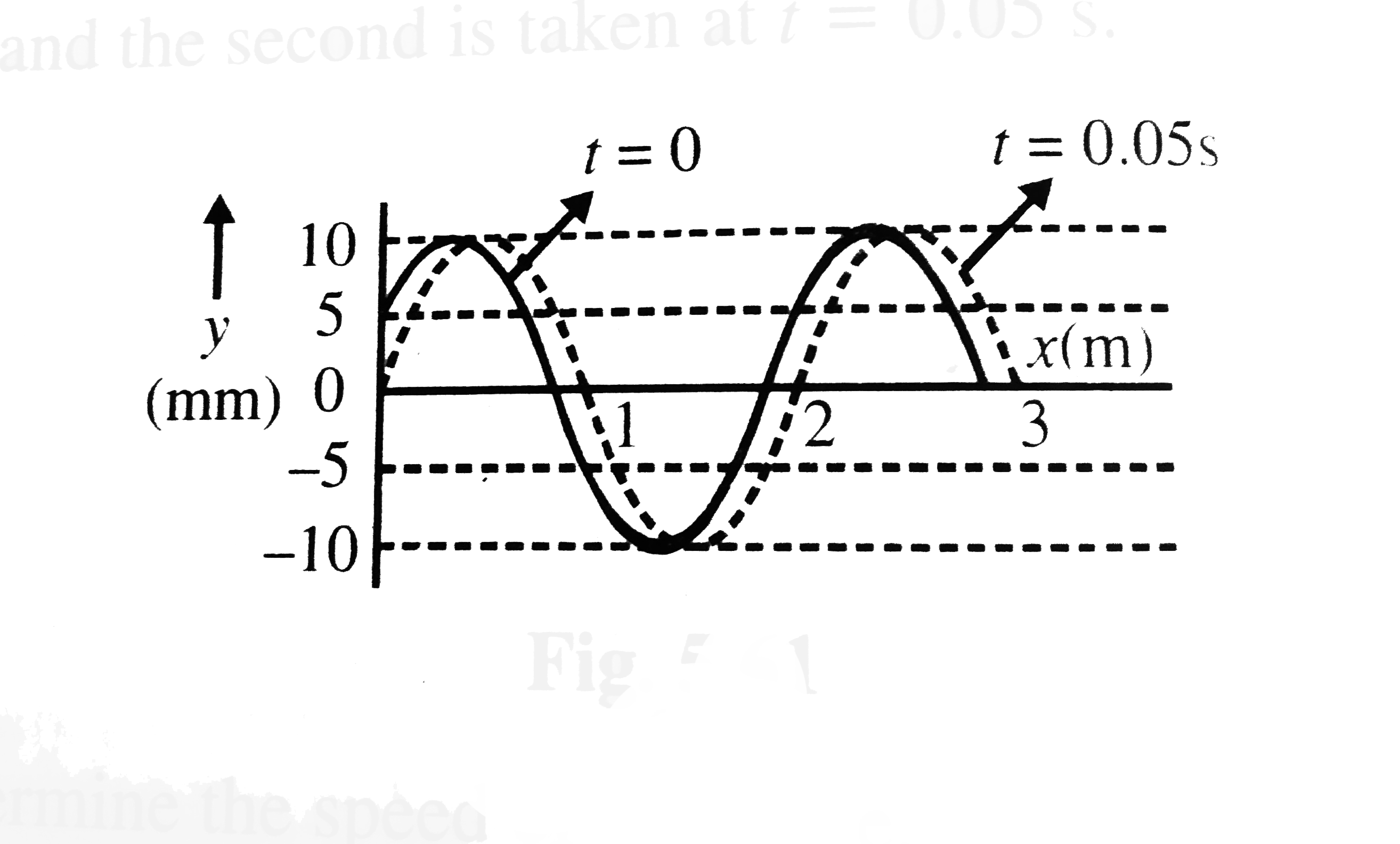
Determine the tension in the string.
View Solution
