Prove that the line joining the mid-points of the two sides of a triangle is parallel to the third side.
To prove that the line joining the midpoints of two sides of a triangle is parallel to the third side, we can follow these steps:
Step 1: Define the Triangle and Midpoints
Let triangle ABC have vertices A, B, and C with coordinates:
- A (x₁, y₁)
- B (x₂, y₂)
- C (x₃, y₃)
Let D and E be the midpoints of sides AB and AC, respectively.
Step 2: Find the Coordinates of the Midpoints
The coordinates of the midpoints D and E can be calculated as follows:
- Midpoint D of AB:
D=(x₁+x₂2,y₁+y₂2)
- Midpoint E of AC:
E=(x₁+x₃2,y₁+y₃2)
Step 3: Calculate the Slope of the Third Side BC
The slope of line segment BC can be calculated using the formula for slope:
slope of BC=y₃−y₂x₃−x₂
Step 4: Calculate the Slope of Line DE
The slope of line segment DE can be calculated as follows:
slope of DE=(y₁+y₃2)−(y₁+y₂2)(x₁+x₃2)−(x₁+x₂2)
Simplifying the numerator:
y₁+y₃−(y₁+y₂)2=y₃−y₂2
And simplifying the denominator:
x₁+x₃−(x₁+x₂)2=x₃−x₂2
Thus, the slope of DE becomes:
slope of DE=y₃−y₂2x₃−x₂2=y₃−y₂x₃−x₂
Step 5: Compare the Slopes
Now we compare the slopes of BC and DE:
- Slope of BC: y₃−y₂x₃−x₂
- Slope of DE: y₃−y₂x₃−x₂
Since the slopes of BC and DE are equal, we conclude that:
slope of DE=slope of BC
Conclusion
Since the slopes of DE and BC are equal, we can conclude that the line joining the midpoints D and E is parallel to the third side BC.
---
Let triangle ABC have vertices A, B, and C with coordinates:
- A (x₁, y₁)
- B (x₂, y₂)
- C (x₃, y₃)
The coordinates of the midpoints D and E can be calculated as follows:
- Midpoint D of AB:
D=(x₁+x₂2,y₁+y₂2)
- Midpoint E of AC:
E=(x₁+x₃2,y₁+y₃2)
The slope of line segment BC can be calculated using the formula for slope:
slope of BC=y₃−y₂x₃−x₂
The slope of line segment DE can be calculated as follows:
slope of DE=(y₁+y₃2)−(y₁+y₂2)(x₁+x₃2)−(x₁+x₂2)
Simplifying the numerator:
y₁+y₃−(y₁+y₂)2=y₃−y₂2
And simplifying the denominator:
x₁+x₃−(x₁+x₂)2=x₃−x₂2
Thus, the slope of DE becomes:
slope of DE=y₃−y₂2x₃−x₂2=y₃−y₂x₃−x₂
Now we compare the slopes of BC and DE:
- Slope of BC: y₃−y₂x₃−x₂
- Slope of DE: y₃−y₂x₃−x₂
slope of DE=slope of BC
Since the slopes of DE and BC are equal, we can conclude that the line joining the midpoints D and E is parallel to the third side BC.
Topper's Solved these Questions
Similar Questions
Knowledge Check
- Question 1 - Select One
Assertion : ABCD and PQRC are rectangles and Q is a midpoint of AC. Then DP = PC.
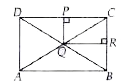
Reason : The line segment joining the midpoints of any two sides of a triangle is parallel to the third side and equal to half of it.AIf both assertion and reason are true and reason is the correct explanation of assertionBIf both assertion and reason are true but reason is not the correct explanation of assertion.CIf assertion is true but reason is false.DIf assertion is false but reason is true. - Question 2 - Select One
Assertion : If a line divides any two sides of a triangle in the same ratio , then the line is paralle to third side .
Reason : Line segment joining the mid -point of any two sides of a triangle is parallel to the third side .AIf both assertion and reason are true and reason is the correct explanation of assertion .BIf both assertion and reason are true but reason is not the correct explanation of assertion .CIf assertion is true reason is false .DIf assertion is false but reason is true .
The line joining the mid-points of two sides of a triangle is parallel to the third side.
View SolutionProve that the line segment joining the mid points of two side of a triangle is parallel to the third side and equal to half of it.
View SolutionProve that the line segment joining the mid points of two sides of a triangle is parallel to its third side.
View Solutionprove that the line segment joining the mid-points of two sides of a triangle is parallel to the third side.
View SolutionProve that the line segment joining the middle points of two sides of a triangle is half the third side.
View SolutionThe line segment joining the mid-points of any two sides of a triangle is parallel to the third side of a triangle.
View Solution

