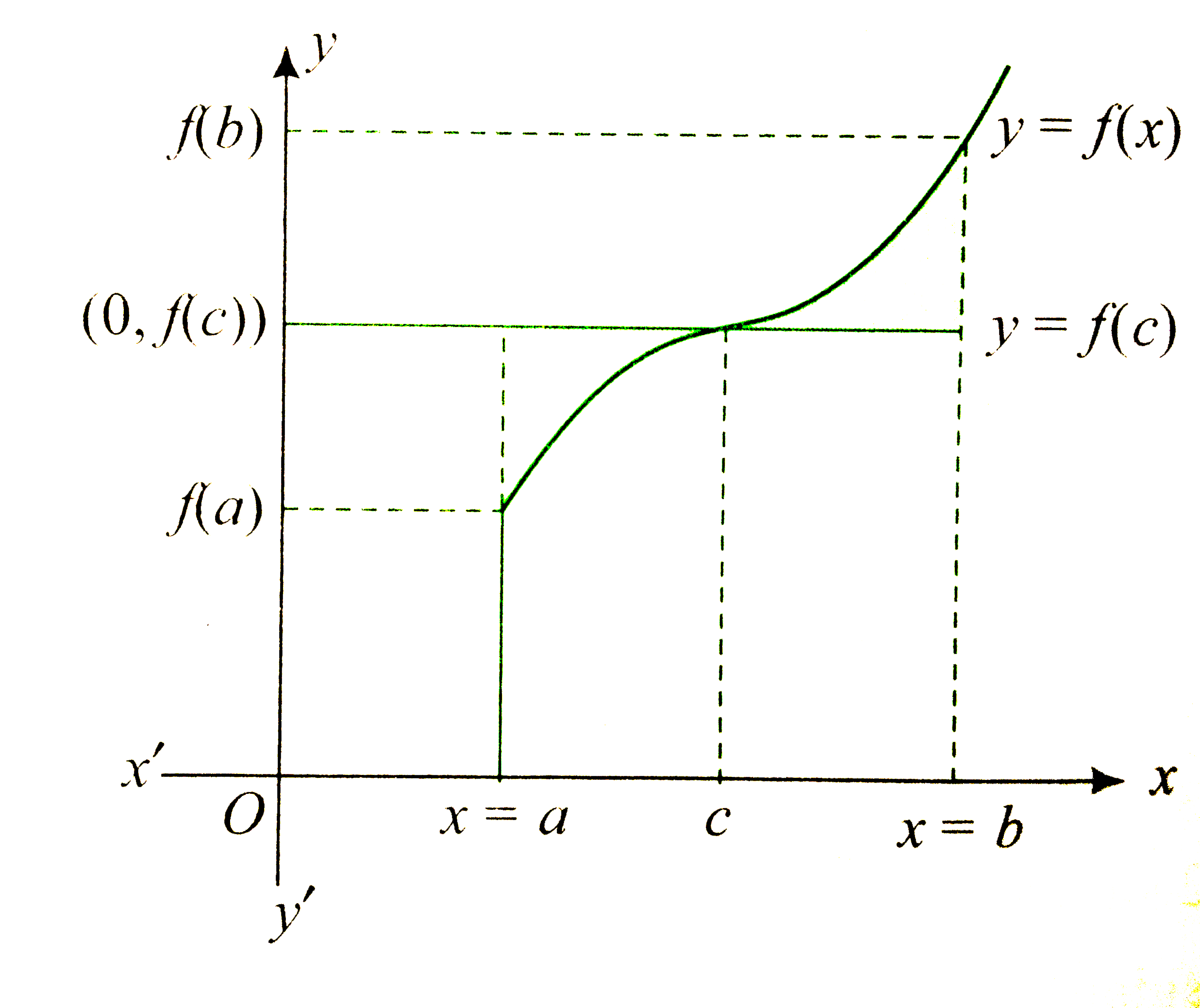
If y=f(x) is a monotonic function in (a,b), then the area bounded by the ordinates at x=a,x=b,y=f(x)andy=f(c)(where c∈(a,b)) is minimum when c=a+b2.
Proof : A=c∫a(f(c)−f(x))dx+b∫c(f(c))dx
=f(c)(c−a)−c∫a(f(x))dx+b∫a(f(x))dx−f(c)(b−c)
⇒ A=[2c−(a+b)]f(c)+b∫c(f(x))dx−c∫a(f(x))dx
Differentiating w.r.t. c, we get
dAdc=[2c−(a+b)]f'(c)+2f(c)+0−f(c)−(f(c)−0)
For maxima and minima , dAdc=0
⇒ f'(c)[2c−(a+b)]=0(asf'(c)≠0)
Hence, c=a+b2
Also for c<a+b2,dAdc<0 and for c>a+b2,dAdc>0
Hence, A is minimum when c=a+b2.
If the area bounded by f(x)=x33−x2+a and the straight lines x=0, x=2, and the x-axis is minimum, then the value of a is
The correct Answer is:a=23
Step by step video & text solution for If y=f(x) is a monotonic function in (a,b), then the area bounded by the ordinates at x=a, x=b, y=f(x) and y=f(c)("where "c in (a,b))" is minimum when "c=(a+b)/(2). "Proof : " A=overset(c)underset(a)int(f(c)-f(x))dx+overset(b)underset(c)int(f(c))dx =f(c)(c-a)-overset(c)underset(a)int(f(x))dx+overset(b)underset(a)int(f(x))dx-f(c)(b-c) rArr" "A=[2c-(a+b)]f(c)+overset(b)underset(c)int(f(x))dx-overset(c)underset(a)int(f(x))dx Differentiating w.r.t. c, we get (dA)/(dc)=[2c-(a+b)]f'(c)+2f(c)+0-f(c)-(f(c)-0) For maxima and minima , (dA)/(dc)=0 rArr" "f'(c)[2c-(a+b)]=0(as f'(c)ne 0) Hence, c=(a+b)/(2) "Also for "clt(a+b)/(2),(dA)/(dc)lt0" and for "cgt(a+b)/(2),(dA)/(dc)gt0 Hence, A is minimum when c=(a+b)/(2). If the area bounded by f(x)=(x^(3))/(3)-x^(2)+a and the straight lines x=0, x=2, and the x-axis is minimum, then the value of a is by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Topper's Solved these Questions
- BOOK - MOTIONCHAPTER - AREA UNDER THE CURVEEXERCISE - EXERCISE - 4 LEVEL - I11videos
AREA UNDER THE CURVE
- BOOK - MOTIONCHAPTER - AREA UNDER THE CURVEEXERCISE - EXERCISE - 4 LEVEL - II14videos
AREA UNDER THE CURVE
- BOOK - MOTIONCHAPTER - AREA UNDER THE CURVEEXERCISE - EXERCISE - 2 (LEVEL-II)4videos
AREA UNDER THE CURVE
- BOOK - MOTIONCHAPTER - BASIC MATHEMATIC & LOGARITHM EXERCISE - Exercise - 44videos
BASIC MATHEMATIC & LOGARITHM
Similar Questions
Knowledge Check
- Question 1 - Select One
If y=f(x) is a monotonic function in (a,b), then the area bounded by the ordinates at x=a,x=b,y=f(x)andy=f(c)(where c∈(a,b)) is minimum when c=a+b2.
Proof : A=∫ca(f(c)−f(x))dx+∫bc(f(c))dx
=f(c)(c−a)−∫ca(f(x))dx+∫ba(f(x))dx−f(c)(b−c)
⇒ A=[2c−(a+b)]f(c)+∫bc(f(x))dx−∫ca(f(x))dx

Differentiating w.r.t. c, we get
dAdc=[2c−(a+b)]f'(c)+2f(c)+0−f(c)−(f(c)−0)
For maxima and minima , dAdc=0
⇒ f'(c)[2c−(a+b)]=0(asf'(c)≠0)
Hence, c=a+b2
Also for c<a+b2,dAdc<0 and for c>a+b2,dAdc>0
Hence, A is minimum when c=a+b2.
If the area bounded by f(x)=x33−x2+a and the straight lines x=0, x=2, and the x-axis is minimum, then the value of a isA1/2B2C1D2/3 - Question 2 - Select One
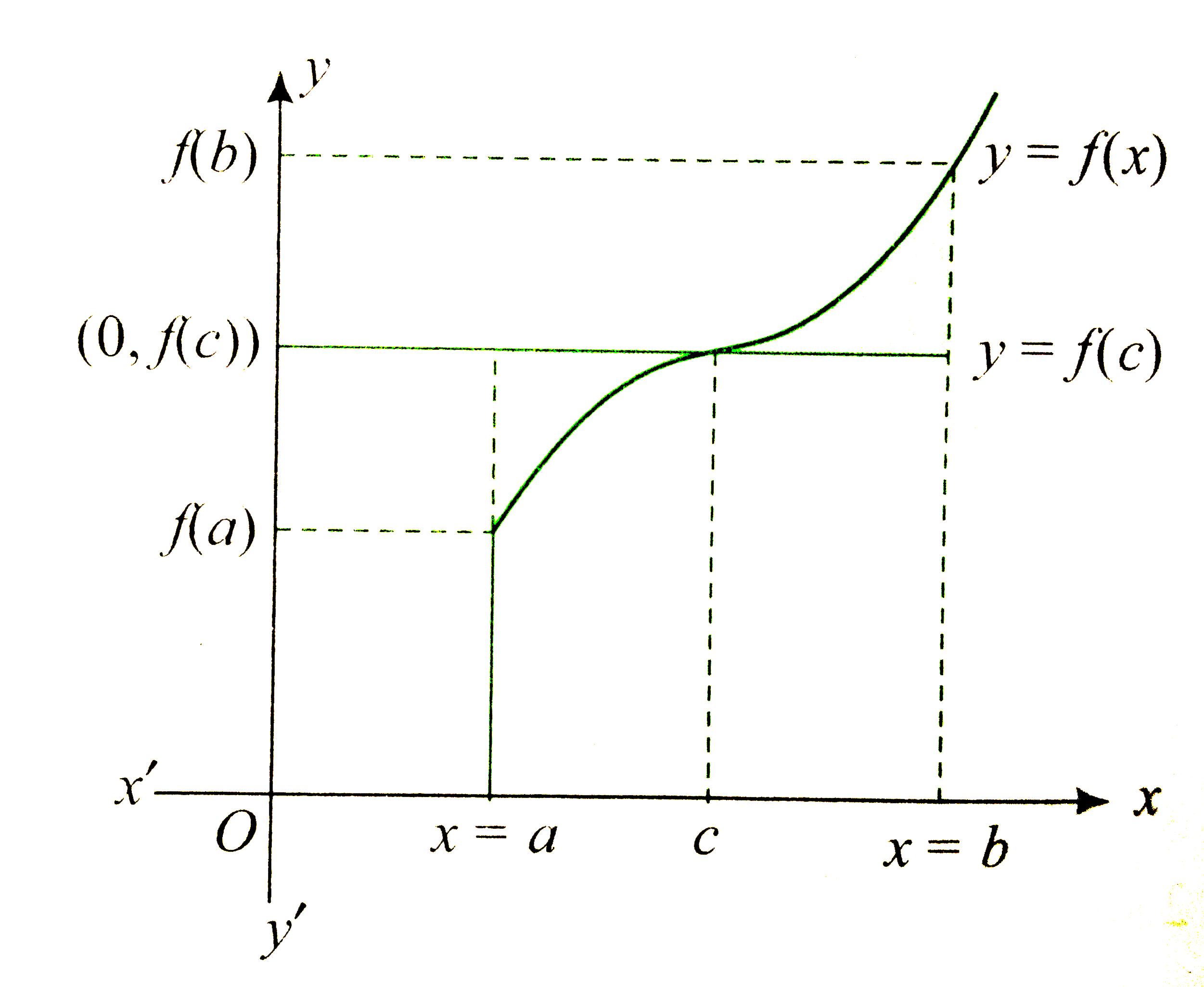
If y=f(x) is a monotonic function in (a,b), then the area bounded by the ordinates at x=a,x=b,y=f(x)andy=f(c)(where c∈(a,b)) is minimum when c=a+b2.
Proof : A=∫ca(f(c)−f(x))dx+∫bc(f(c))dx
=f(c)(c−a)−∫ca(f(x))dx+∫ba(f(x))dx−f(c)(b−c)
⇒ A=[2c−(a+b)]f(c)+∫bc(f(x))dx−∫ca(f(x))dx
Differentiating w.r.t. c, we get
dAdc=[2c−(a+b)]f'(c)+2f(c)+0−f(c)−(f(c)−0)
For maxima and minima , dAdc=0
⇒ f'(c)[2c−(a+b)]=0(asf'(c)≠0)
Hence, c=a+b2
Also for c<a+b2,dAdc<0 and for c>a+b2,dAdc>0
Hence, A is minimum when c=a+b2.
If the area enclosed by f(x)=sinx+cosx,y=a between two consecutive points of extremum is minimum, then the value of a isA0B-1C1D2 - Question 3 - Select One
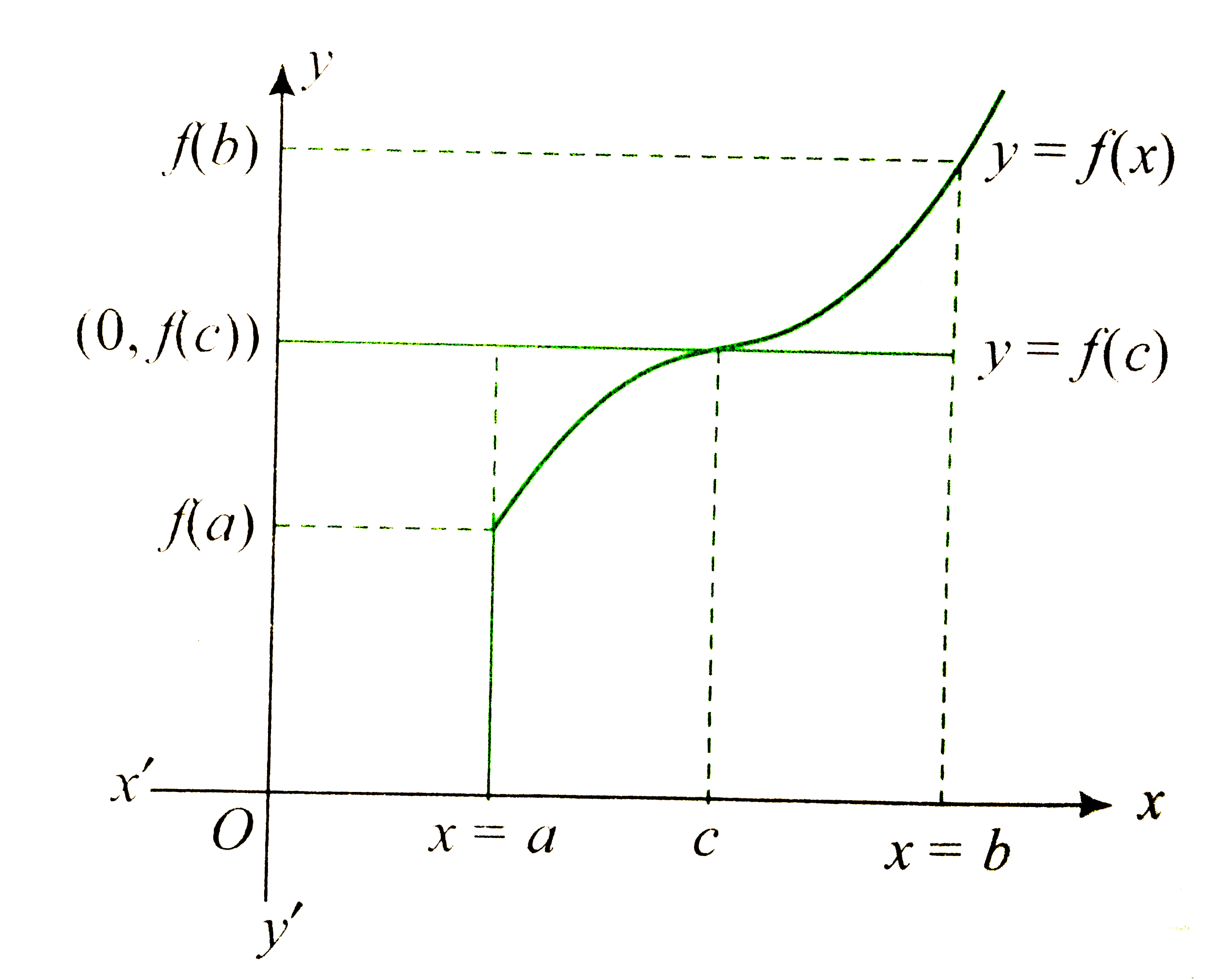
If y=f(x) is a monotonic function in (a,b), then the area bounded by the ordinates at x=a,x=b,y=f(x)andy=f(c)(where c∈(a,b)) is minimum when c=a+b2.
Proof : A=∫ca(f(c)−f(x))dx+∫bc(f(c))dx
=f(c)(c−a)−∫ca(f(x))dx+∫ba(f(x))dx−f(c)(b−c)
⇒ A=[2c−(a+b)]f(c)+∫bc(f(x))dx−∫ca(f(x))dx
Differentiating w.r.t. c, we get
dAdc=[2c−(a+b)]f'(c)+2f(c)+0−f(c)−(f(c)−0)
For maxima and minima , dAdc=0
⇒ f'(c)[2c−(a+b)]=0(asf'(c)≠0)
Hence, c=a+b2
Also for c<a+b2,dAdc<0 and for c>a+b2,dAdc>0
Hence, A is minimum when c=a+b2.
The value of the parameter a for which the area of the figure bounded by the abscissa axis, the graph of the function y=x3+3x2+x+a, and the straight lines, which are parallel to the axis of ordinates and cut the abscissa axis at the point of extremum of the function, which is the least, isA2B0C-1D1
if ∫dxf(x)=log(f(x))2+C then
View Solutionif ∫dxf(x)=log(f(x))2+C then
View SolutionCompare the stabilities of the following carbanions :
Cl−Cl∣C∣Cl−¯¯¯CH2(a)
F−Cl∣C∣F−¯¯¯CH2(b)View SolutionIf a∫0f(2a−x)dx=manda∫0f(x)dx=n, then 2a∫0f(x)dx is equal to
View SolutionLet ∫dx√x2+1−x=f(x)+C such that f(0)=0 and C is the constant of integration, then the value of f(1) is
View Solution

