All the surfaces shown in figure are assumed to be frictionless. The block of mass m slides on the prism which in turn slides backward on the horizontal surface. Find the aceleration of the smaller block with respect to the prism.
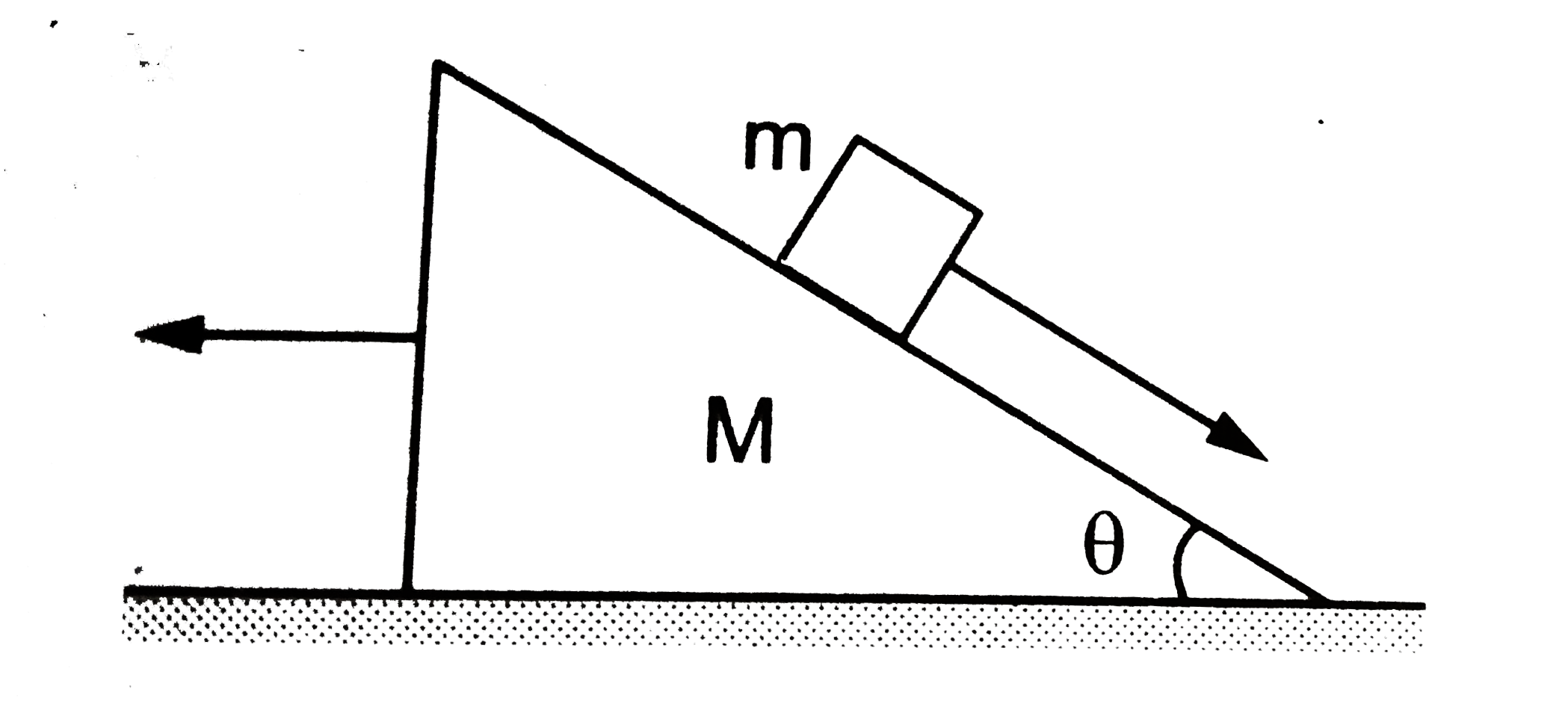
Let the acceleration of the prism be a0 in the backward direction. Consider the motion of the smaller block from the frame of the prism The forces on the block are ( figure )
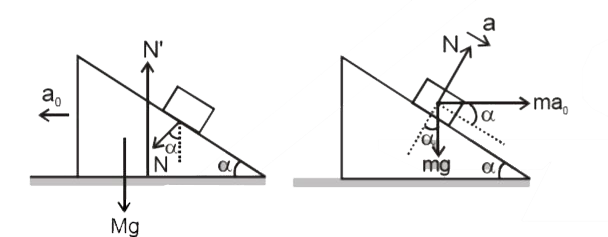
(i) N normal force
(ii) mg downward ( gravity ),
(iii) ma0 forward ( Pseudo Force)
The block slides down the the plane . Components of the forces parallel to the incline give
ma0cosα+mgsinα=ma
or, a=a0cosα+gsinα ....(i)
Components of the forces perpendicular to the incline give
N+ma0sinα=mgcosα .....(ii)
Now consider the motion of the prism from the ground frame. No pseudo force is needed as the frame used in inertial. The forces are (figure )
(i) Mg downward
(ii) N normal to the incline ( by the block )
(iii) N' upward ( by the horizontal surface )
Horizontal components give,
Nsinα=Ma0 or N=Ma0/sinα, .....(iii)
Putting in (ii)
Ma0sinα+ma0α=mgcosα
or,a0=mgsinαcosαM+msin2α
From (i) a=mgsinαcos2αM+msin2α+gsinα
=(M+m)gsinαM+msin2α
Topper's Solved these Questions
- NLM & FRICTIONBook:MOTIONChapter:NLM & FRICTIONExercise:SOLVED EXAMPLE
Explore 16 Videos
- NLM & FRICTIONBook:MOTIONChapter:NLM & FRICTIONExercise:EXERCIS-1
Explore 80 Videos
- NLM & FRICTIONBook:MOTIONChapter:NLM & FRICTIONExercise:EXERCISE-4 ( LEVEL-II)
Explore 15 Videos
- NEWTON'S LAWS OF MOTION & FRICTION Book:MOTIONChapter:NEWTON'S LAWS OF MOTION & FRICTION Exercise:Exercise - 3 Section-B
Explore 12 Videos
- ONE DIMENSION MOTIONBook:MOTIONChapter:ONE DIMENSION MOTIONExercise:Exercise - 3 |Section - B Previous Year Problems | JEE MAIN
Explore 12 Videos
Similar Questions
A block of mass m slides on the wooden wedge, which in turn slides backward on the horizontal surface. The acceleration of the block with respect to the wedge is :
Given m=8kg,M=16mg
Assume all the surfaces shown in the figure to be frictionless.
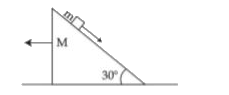
View SolutionA block of mass m slides on the wooden wedge, which in turn slides backward on the horizontal surface. The acceleration of the block with respect to the wedge is :
Given m=8kg,M=16mg
Assume all the surfaces shown in the figure to be frictionless.
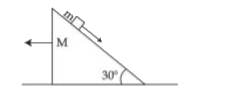
View Solution
Knowledge Check
Assuming all the surface to be frictionless. The smaller block m is moving horizontally with acceleration a and vertically downwards with acceleration a. Then magnitude of net acceleration of smaller block m with respect to ground
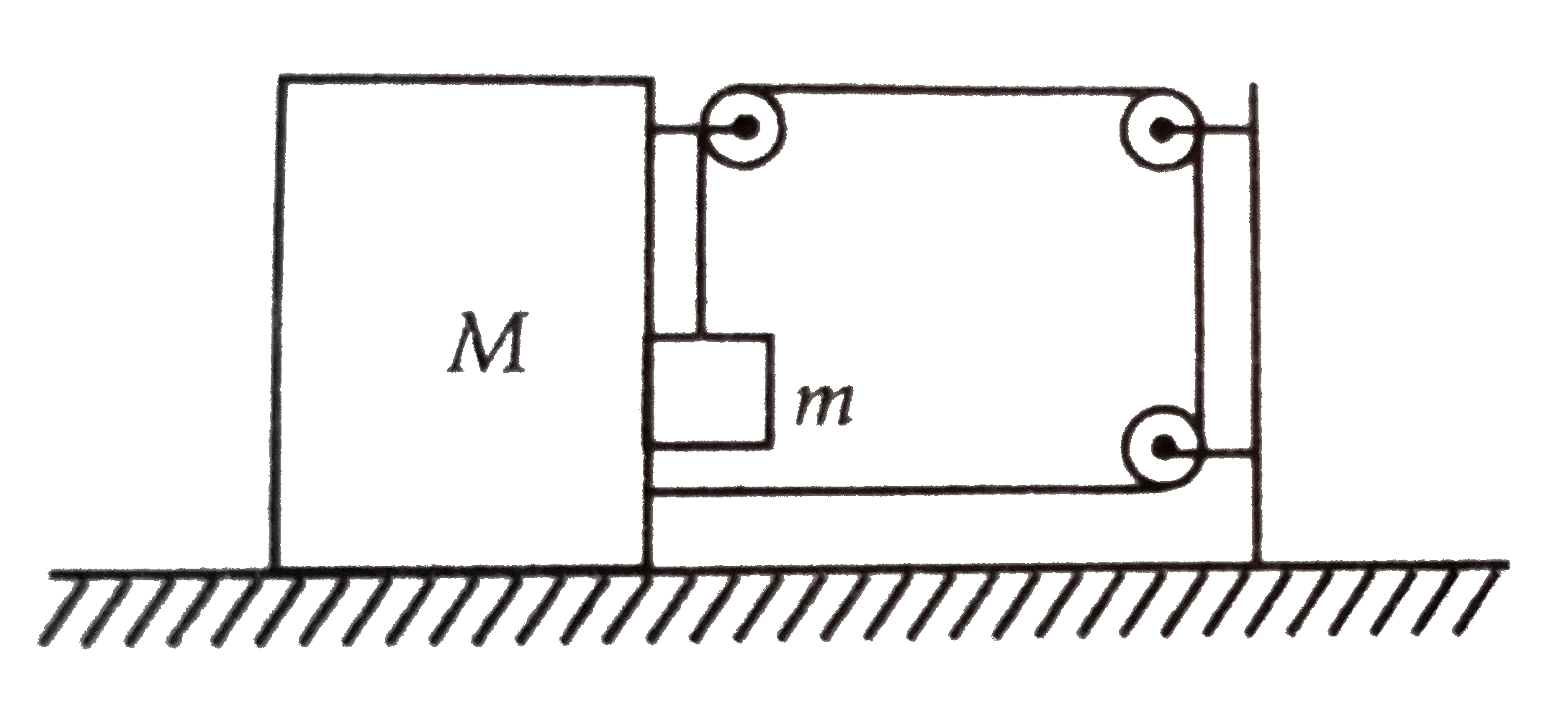 A2√5mg(5m+M)B2mg(5m+M)C7√5gDnone of these
A2√5mg(5m+M)B2mg(5m+M)C7√5gDnone of theseAssuming all the surfaces to be frictionless accelration of the block c shown in the figure is
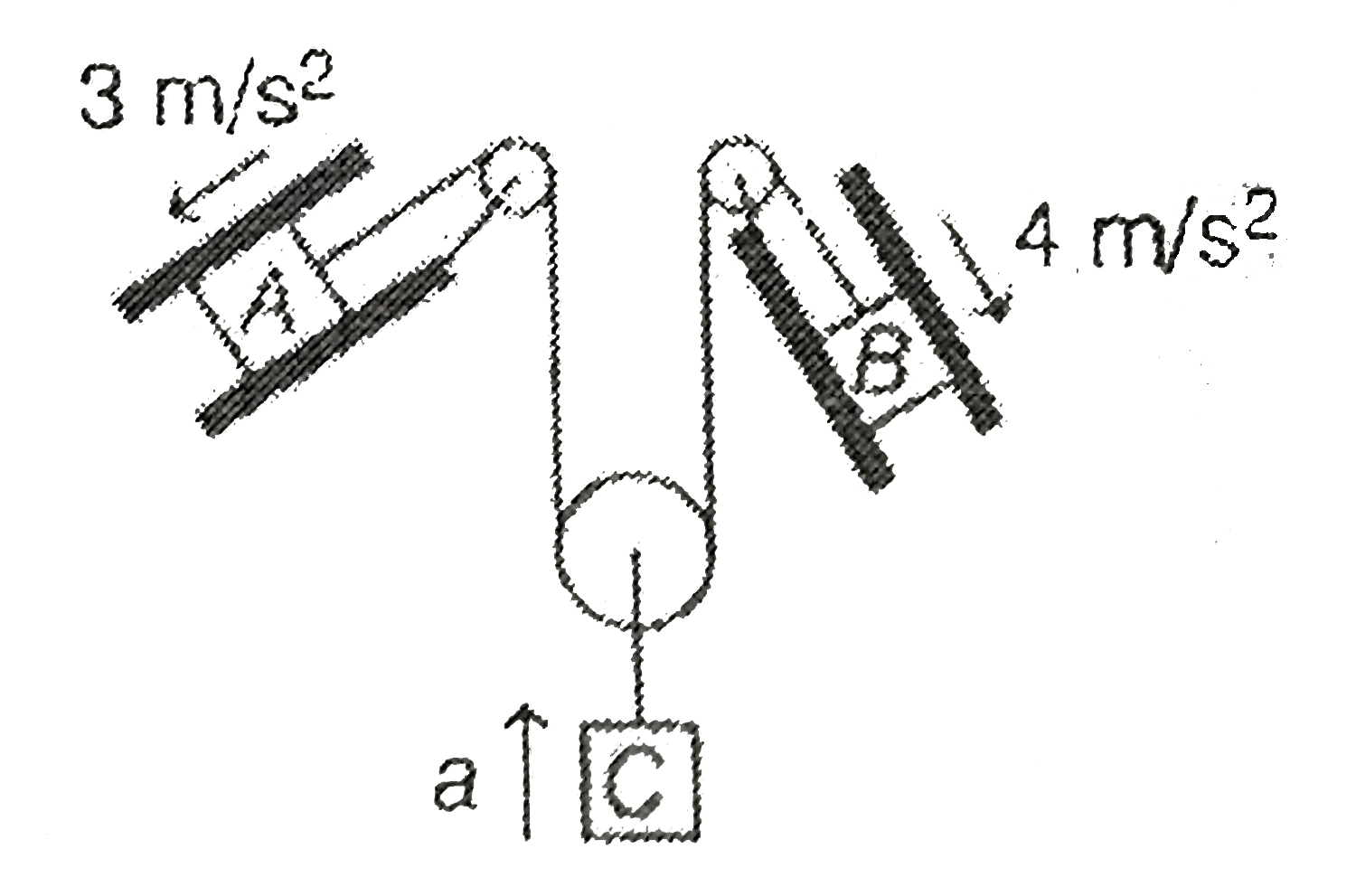 A5m/s2B7m/s2C3.5m/s2D4m/s2
A5m/s2B7m/s2C3.5m/s2D4m/s2A block of mass m rests on a stationary wedge of mass M. The wedge can slide freely on a smooth horizontal surface as shown in figure. If the block starts from rest
 A(a) the position of the centre of mass of the system will changeB(b) the position of centre of mass of the system will change along the vertical but not along the horizontalC(c) the total energy of the system will remain constantD(d) All of the above
A(a) the position of the centre of mass of the system will changeB(b) the position of centre of mass of the system will change along the vertical but not along the horizontalC(c) the total energy of the system will remain constantD(d) All of the above
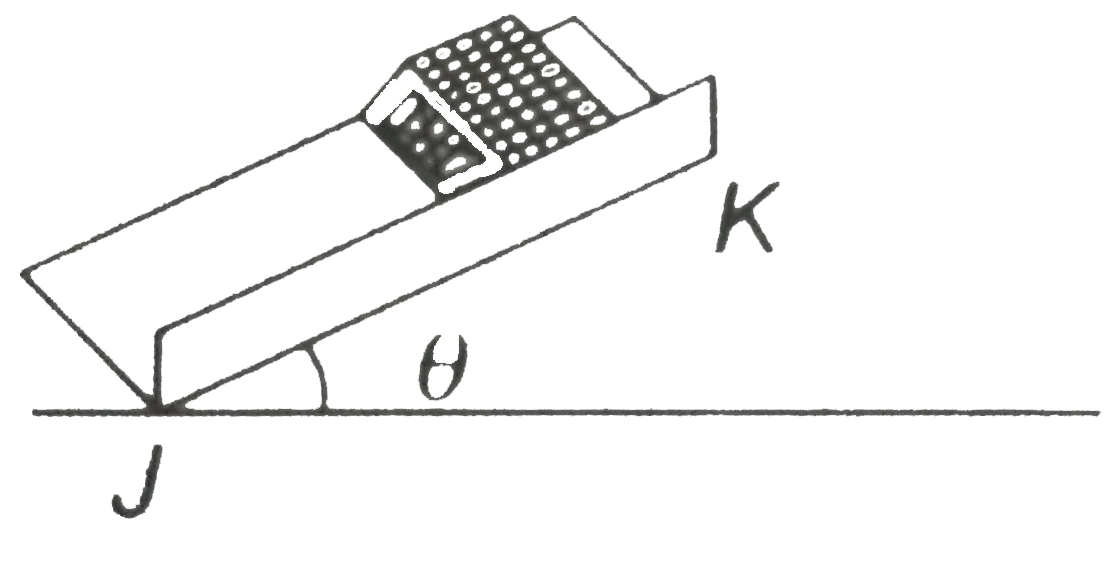
A cubical block of mass m is sliding on an inclined right angle trough as shown in the figure. If coefficient of friction is μ , find the acceleration of the block.
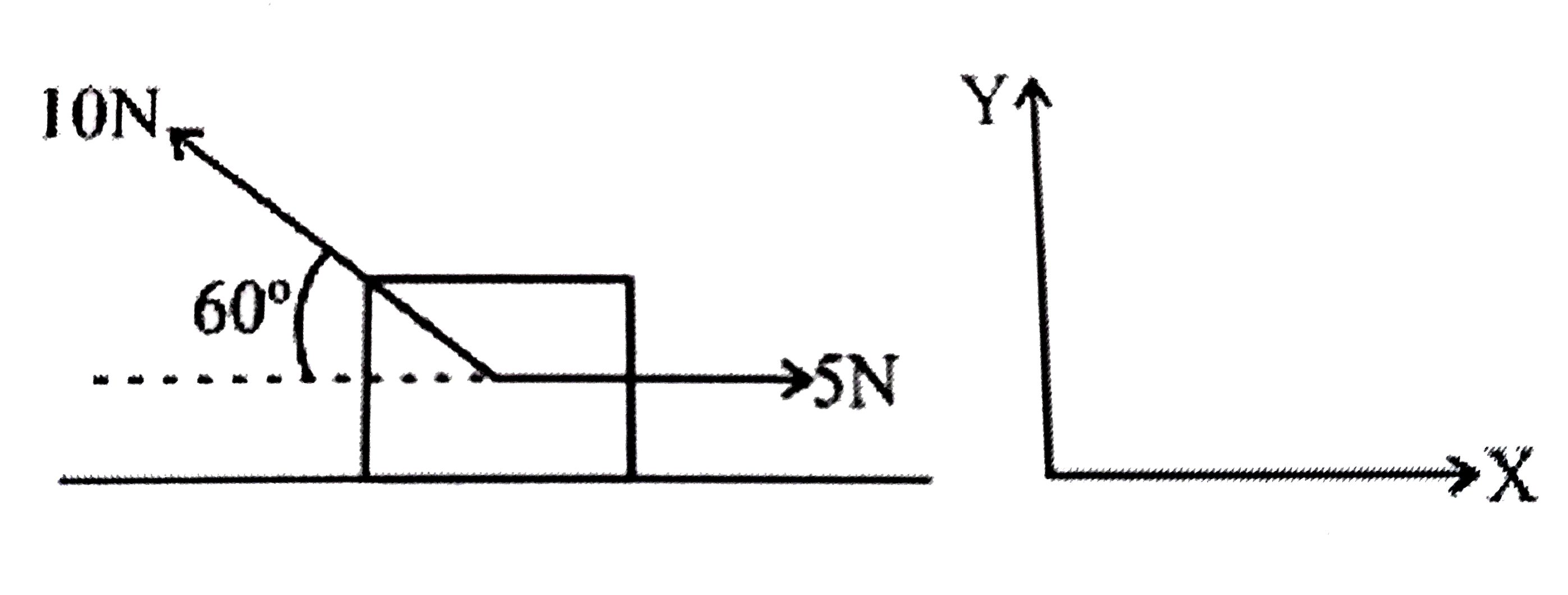
View SolutionA block of mass √3kg rests on a horizontal frictionless surface. What will be the acceleration of the block if it is subjected to two forces as shown in the figure.
View SolutionA block of mass M is placed on the top of a bigger block of mass 10 M as shown in figure. All the surfaces are frictionless. The system is released from rest, then the distance moved by the bigger block at the instant the smaller block reaches the ground :
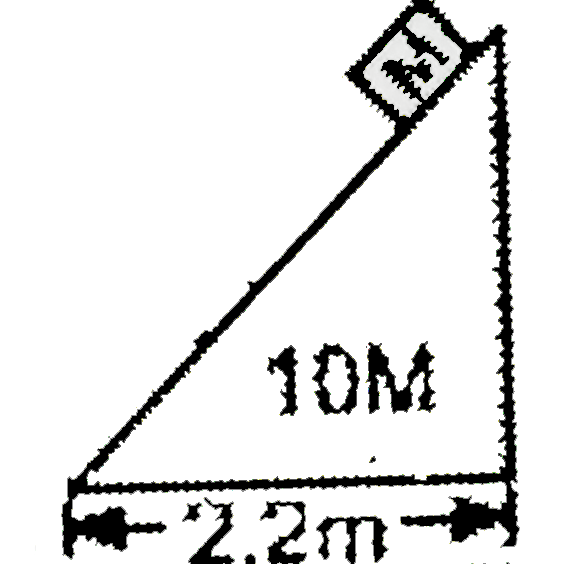
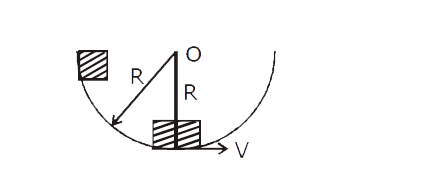
View SolutionA block of mass m slides down along the surface of the bowl from the rim to the bottom as shown in fig. The velocity of the block at the bottom will be-
View SolutionA block of mass 2kg slides down the face of a smooth 45∘ wedge of mass 9kg as shown in the figure. The wedge is placed on a frictionless horizontal surface. Determine the acceleration of the wedge.

View Solution