In the figure shown find moment of inertia of a plate having mass M, length l and width b about axis 1,2,3 and 4. Assume that C is centre and mass is uniformly distributed
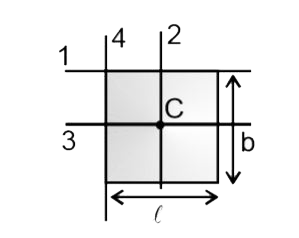
Moment of inertia of the plate about axis 1 (by taking rods perpendicular to axis 1)
11=Mb2/12
Moment of inertia of the plate about axis 2 (by taking rods perpendicular to axis 2)
I2=Ml2/12
Moment of inertia of the plate about axis 3 (by taking rods perpendicular to axis 3)
l3=Mb212
Moment of inertia of the plate about axis 4(by taking rods perpendicular to axis 4)
I4=Ml2/3
Topper's Solved these Questions
Similar Questions
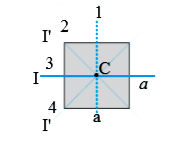
In the figure shown find the moment of inertia of square plate having mass m and sides a. About an axis 2 passing through point C (centre of mass) and in the plane of plate?
 View Solution
View SolutionThe moment of inertia of a thing uniform rod of length L and mass M, about axis CD (as shown in figure) is
 View Solution
View Solution
Knowledge Check
- Question 1 - Select One
The moment of inertia of a door of mass m, length 2l and width l about its longer side is.
A11ml224B5ml224Cml23Dnone of these - Question 2 - Select One
The moment of inertia of a cylinder of radius R, length L and mass M about an axis passing through its centre of mass and normal to its length is
AML212BMR24CM[L212+R24]DM[L212+R22] - Question 3 - Select One
The moment of inertia of a system of four rods each of length l and mass m about the axis shown is
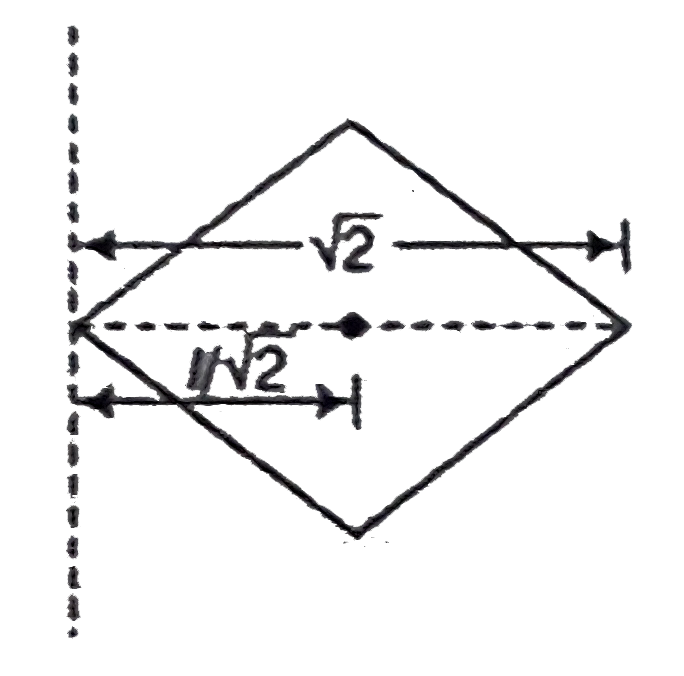 A23ml2B2ml2C3 ml2D83ml2
A23ml2B2ml2C3 ml2D83ml2
What is moment of inertia of a solid cylinder of mass m, length l and radiusr about the axis of the cylinder ?
View SolutionThe moment of inertia of a square plate of mass 4kg and side 1m about an axis passing through its centre and perpendicular to its plane is
View SolutionThe moment of inertia of a thin uniform rod of mass M, length L, about an axis passing through its centre and making an angle θ with the rod is
View SolutionFind out the centre of mass of an isosceles triangle of base length a and alititude b. Assume that the mass of the triangle is uniformly distributed over its area.
View SolutionMoment of inertia of a rod of mass M and length L about an axis passing through a point midway between centre and end is
View Solution

