A plate of mass 10gm is in equillibrium in air due to the force exterted by light beam on plate. Calculate powder of beam. Assume plates is perfectly absorbing.

2×107W
5×107W
3×103W
3×107W
The correct Answer is:D
Step by step video & image solution for A plate of mass 10 gm is in equillibrium in air due to the force exterted by light beam on plate. Calculate powder of beam. Assume plates is perfectly absorbing. by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- MODERN PHYSICS -1BOOK - MOTIONCHAPTER - MODERN PHYSICS -1EXERCISE - EXERCISE-2(LEVEL-II)
15 Videos
- MODERN PHYSICS -1BOOK - MOTIONCHAPTER - MODERN PHYSICS -1EXERCISE - EXERCISE-3( LEVEL-I)
25 Videos
- MODERN PHYSICS -1BOOK - MOTIONCHAPTER - MODERN PHYSICS -1EXERCISE - EXERCISE-1
50 Videos
- MODERN PHYSICS - 2BOOK - MOTIONCHAPTER - MODERN PHYSICS - 2EXERCISE - EXERCISE - 4 (Level -II) PREVIOUS YEAR - JEE ADVANCED
28 Videos
- NEWTON'S LAWS OF MOTION & FRICTION BOOK - MOTIONCHAPTER - NEWTON'S LAWS OF MOTION & FRICTION EXERCISE - Exercise - 3 Section-B
12 Videos
Similar Questions
A plate of mass 10g is in equilibrium in air due to the force exerted by a light beam on the plate. Calculate power of the beam. Assume that the plate is perfectly absorbing.
View SolutionA plate of mass 20mg is in equilibrium in air due to force exerted by light beam on the plate as shown is the figure. Calculate the power of the beam (in mega-watt) if the plate is perfectly absorbing.
'(##CEN_KSR_PHY_JEE_CO28_E01_018_Q01##)'
View Solution
Knowledge Check
A narrow beam of monochromatic light of wavelength λ emitted from a source of power P is propogating in the positive x-direction. After being reflected from a perfectly reflecting plane mirror of area vector →A=A(−ˆi−ˆj), the beam falls on a metal plate of surface area A[→A is along outward normal at mirror]. the force exerted by light beam on the mirror is →F and Δ→p is change in momentum of each photon then [cis speed of light in vacuum]
AΔ→F=Pc(ˆi+ˆj),Δ→p=hλ(−ˆi−ˆj)BΔ→F=Pc(ˆi+ˆj),Δ→p=hλ(ˆi+ˆj)CΔ→F=Pc(−ˆi−ˆj),Δ→p=hλ(−ˆi−ˆj)DΔ→F=Pc(−ˆi−ˆj),Δ→p=hλ(+ˆi+ˆj)In Young's double slit experiment, the fringes are displaced by a distance x when a glass plate of one refractive index 1.5 is introduced in the path of one of the beams. When this plate in replaced by another plate of the same thickness, the shift of fringes is (3/2)x. The refractive index of the second plate is
A1.75B1.5C1.25D1When a thin metal plate is placed in the path of one of the interfering beams of light
AFringe width increasesBFringes disappearCFringes become brighterDFringes becomes blurred
A parallel beam of monochromatic light of frequency v is incident on a surface. Intensity of the beam is I and area of the surface is A. Find the force exerted by the light beam on the surface for following cases–
(i) the surface is perfectly absorbing and the light beam is incident normally on it.
(ii) the surface is perfectly reflecting and the light beam is incident normally.
(iii) the surface is perfectly absorbing and the light beam is incident at an angle of incidence θ.
(iv) the surface is perfectly reflecting and the light beam is incident at an angle of incidence θ.
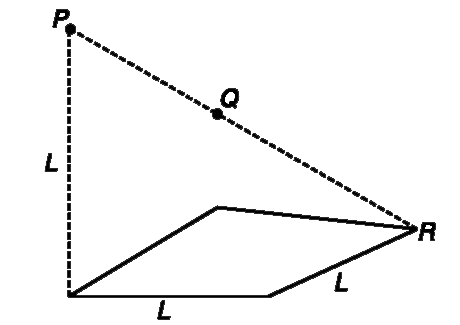
View SolutionA square plate of side length L absorbs all radiation incident on it. A point source of light is placed at a point P which is directly above a corner of the plate at a height L. The incident light on the plate produces a force of magnitude F0 on the plate. Calculate the magnitude of force on the plate if the source is moved to a point Q (Q is midpoint of the line PR).
View SolutionA beam of light travelling in water falls on a glass plate immersed in water. When the incidence angle is 51∘, then the reflected beam of light is found polarized. Calculate μ of glass. Given μ of water is 4/3.
View SolutionA Square metal plate of side 10 cm is moving parallel to another plate with a velocity of 10cm/s and both plates are immersed in water. If the viscosity of water is 0.01 poise and the distance between the plates is 0.05 cm then calculate the viscous force.
View SolutionA square plate of side 'a' and mass 'm' is lying on a horizontal floor. A force F is applied at the top. Find the maximum force that can be applied on the square plate so that the plate does not topple about A.
View Solution
