Show that the points (2a, 6a) (2a,4a) and (2a+√3a,5a)are the vertices of an equilateral triangle of side 2a units. Solution in Bengali
Step by step video, text & image solution for Show that the points (2a, 6a) (2a,4a) and (2a + sqrt3 a, 5a) are the vertices of an equilateral triangle of side 2a units. by Maths experts to help you in doubts & scoring excellent marks in Class 9 exams.
Topper's Solved these Questions
- BOOK - CALCUTTA BOOK HOUSECHAPTER - DISTANCE FORMULAS EXERCISE - Short answer type questions5videos
DISTANCE FORMULAS
- BOOK - CALCUTTA BOOK HOUSECHAPTER - CONSTRUCTION - DRAWING OF TRIANGLES EQUAL TO THE AREA OF A GIVEN QUADRILATERAL EXERCISE - Exercise -612videos
CONSTRUCTION - DRAWING OF TRIANGLES EQUAL TO THE AREA OF A GIVEN QUADRILATERAL
- BOOK - CALCUTTA BOOK HOUSECHAPTER - FACTORISATIONEXERCISE - EXERCISE 2.4 (Long -answer type questions )11videos
FACTORISATION
Similar Questions
Find the distance between the points (-13,-11) and (-2,-9)
03:01View SolutionAn urn contains 10 red and 8 white balls. One ball is drawn at random. Find the probability that the ball drawn is white.
04:46View SolutionFactorise : a(b2−c2)+b(c2−a2)+c(a2−b2)
08:14View SolutionProve that the points (2a,4a),(2a,6a) and (2a+sqrt(3)a,5a) are the vertices of an equilaterall triangle whose side is 2a
05:49View SolutionProve that the points (2a,4a),(2a,6a)and(2a+√3a,5a) are the vertices of an equilateral triangle.
02:59View Solutionसिद्ध कीजिये की बिंदु (2a,4a),(2a,6a) व (2a+a√3,5a) एक समबाहु त्रिभुज के शीर्ष है|
03:14View Solutionसिद्ध कीजिए कि बिंदु (2a , 4a) , (2a , 6a) तथा (2a + √3a , 5a) एक समबाहु त्रिभुज के शीर्ष हैं । समबाहु त्रिभुज के क्षेत्रफल का सूत्र लिखिए ।
03:29View Solutionसिद्ध कीजिए कि बिन्दु (2a,4a),(2a,6a) तथा (2a+√3a,5a) एक समबाहु त्रिभुज के शीर्ष हैं ।
05:49View Solutionसिद्ध कीजिय की बिंदुएँ (2a,4a),(2a,6a) और (2a+√3a,5a) एक समबाहु त्रिभुज के शीर्ष है | जिसकी प्रत्येक भुजा की लम्बाई 2a इकाइयां है|
03:04View SolutionA(2a,4a),B(2a,6a),C(2a+√3a,5a) then ΔABC is .......triangle.
04:24View SolutionProve that:cos2Acos3A−cos2Acos7A+cosAcos10Asin4Asin3A−sin2Asin5A+sin4Asin7A
=cot6Acot5A08:15View SolutionProve that (2a, 4a), (2a, 6a), and (2a +√3a, 5a) are the vertices of an equilateral triangle.
05:35View SolutionProve that the points (2a,4a),(2a,6a)and(2a+√3a,5a) are the vertices of an equilaterall triangle whose side is 2a
01:37View SolutionIf A(2a,4a),B(2a,6a) are two vartices of a ΔABC then match the vertex C given by triangle
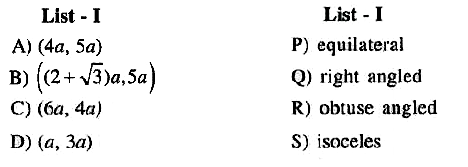 04:13View Solution
04:13View Solutionवक्तव्य । यदि A(2a,4a) तथा B(2a,6a) समबाहु ΔABC के दो शीर्ष । हैं, तब शीर्ष के निर्देशांक (2a+a√3,5a) होंगे।
वक्तव्य || एक समबाहु त्रिभुज के सभी शीर्षों के निर्देशांक परिमेय हो । सकते हैं।02:28View Solution

