What is the maximum possible area of a parallelogram with one side of length 2 meters and a perimeter of 24 meters?
To find the maximum possible area of a parallelogram with one side of length 2 meters and a perimeter of 24 meters, we can follow these steps:
Step 1: Understand the properties of the parallelogram
A parallelogram has opposite sides that are equal in length. If one side is given as 2 meters, then the opposite side is also 2 meters. Let the other two sides be denoted as x.
Step 2: Set up the equation for the perimeter
The perimeter P of a parallelogram is given by the formula:
P=2(length+breadth)
In this case, substituting the known values:
24=2(2+x)
Step 3: Solve for x
Now, we can simplify the equation:
24=4+2x
Subtract 4 from both sides:
20=2x
Now, divide by 2:
x=10
Thus, the lengths of the sides of the parallelogram are 2 meters and 10 meters.
Step 4: Determine the maximum area
The area A of a parallelogram is given by the formula:
A=base×height
To maximize the area, the parallelogram should be a rectangle since a rectangle has the maximum area for a given perimeter. Here, we can consider the base as 10 meters and the height as 2 meters.
Step 5: Calculate the area
Now, we can calculate the area:
A=10×2=20square meters
Conclusion
The maximum possible area of the parallelogram is:
20square meters
---
A parallelogram has opposite sides that are equal in length. If one side is given as 2 meters, then the opposite side is also 2 meters. Let the other two sides be denoted as x.
The perimeter P of a parallelogram is given by the formula:
P=2(length+breadth)
In this case, substituting the known values:
24=2(2+x)
Now, we can simplify the equation:
24=4+2x
Subtract 4 from both sides:
20=2x
Now, divide by 2:
x=10
Thus, the lengths of the sides of the parallelogram are 2 meters and 10 meters.
The area A of a parallelogram is given by the formula:
A=base×height
To maximize the area, the parallelogram should be a rectangle since a rectangle has the maximum area for a given perimeter. Here, we can consider the base as 10 meters and the height as 2 meters.
Now, we can calculate the area:
A=10×2=20square meters
The maximum possible area of the parallelogram is:
20square meters
Similar Questions
Knowledge Check
If the unit of length is x meter then an area of 1m2 will become
AxB1/xCx2D1/x2In the figure below, △ABCand△DEF are similar triangles with the given side lengths in meters. What is the perimeter, in meters, of △DEF ?
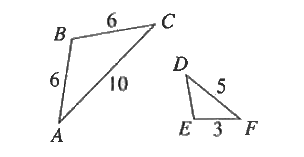 A3B8C11D12
A3B8C11D12
What is the minimum possible perimeter of a quadrilateral with an area of 1,600 square feet?
View SolutionWhat is the perimeter of the parallelogram ?
View SolutionWhat is the greatest possible perimeter of a right angled triangle with integer side lengths if one of the sides has length 12?
View SolutionThe perimeter of a rectangle is 32 cm. Find its maximum possible area if the sides of the rectangle are whole numbers only.
View SolutionWhat is the minimum possible possible perimeter for a rectangle whose area is 4m2 ?
View SolutionThe diagonal of a square is 15 m, find the length of its one side and perimeter.
View Solution
