Eight particles A,B,C,D,E,F,G and H are originally located at the vertices of regular octagon of side length l.At time t=0 second all of them begin to move with constant speed v such that A moves towards B, B moves towards C And so on.They will meet after a time interval of
Step by step video & image solution for Eight particles A,B,C,D,E,F,G and H are originally located at the vertices of regular octagon of side length l.At time t=0 second all of them begin to move with constant speed v such that A moves towards B, B moves towards C And so on.They will meet after a time interval of by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Similar Questions
Three boys A,B and C are situated at the vertices of an equilateral triangle of side d at t=0. Each of the boys moves with constant speed v. A always moves towards C and C towards A, At what time and where they meet each other?
View SolutionThree particles A,B,andC are situated at the vertices of an equilateral triangle of side r at t=0. The particle A heads towards B, B towards C, C towards A with constant speeds v. Find the time of their meeting.
View Solution
Knowledge Check
- Question 1 - Select One or More
Three particles A,B and C and situated at the vertices of an equilateral triangle ABC of side of length l at time t=0,Each of the particles move with constant speed u. A always has its velocity along AB,B along BC and C along CA.
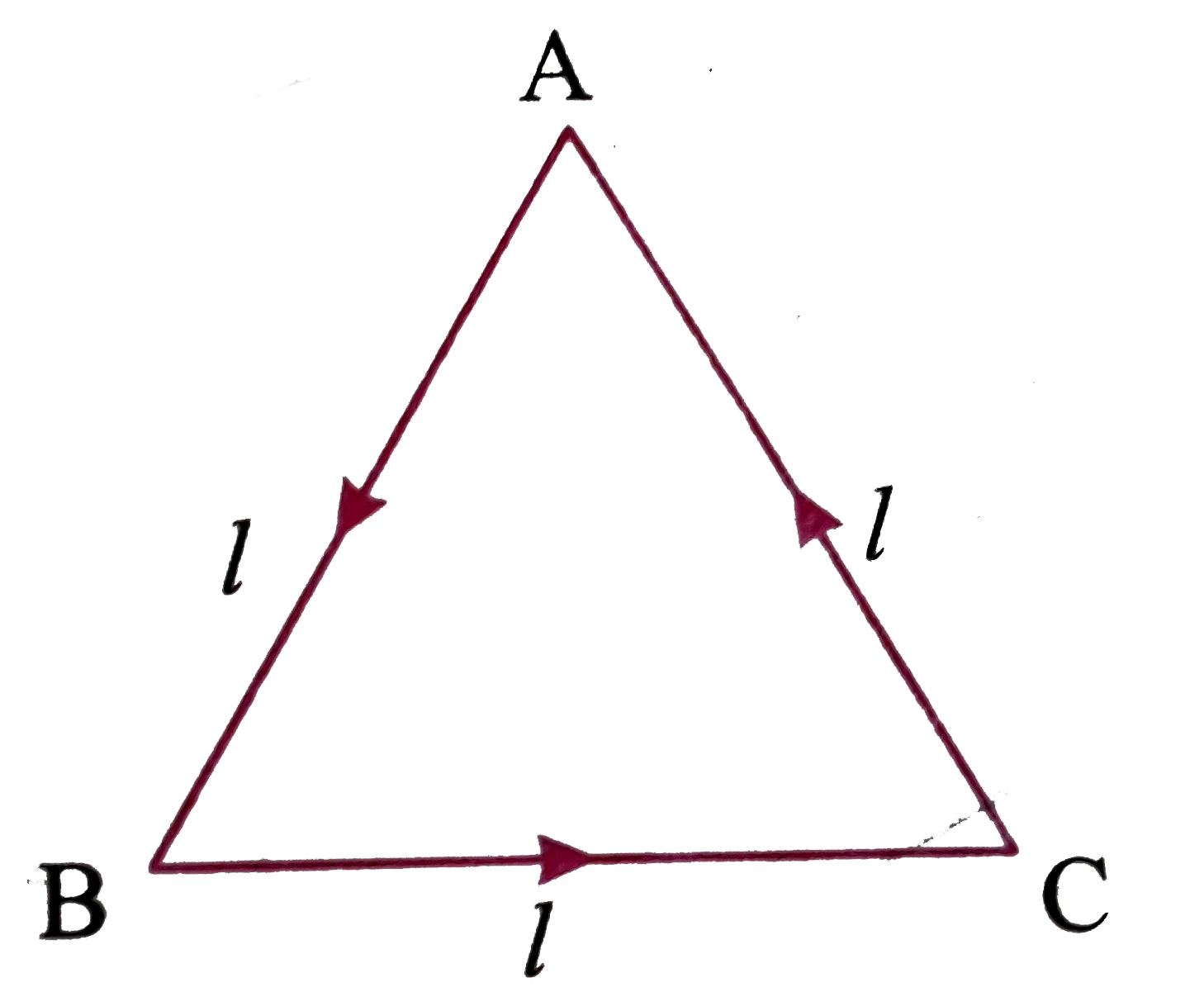 AThe time after which they meet is 2l3uBTotal distance travelled by each particle before they meet is 2l3CAverage velocity during the motion is √3u2DRelative velocity of apporach between any two particles is 3u2
AThe time after which they meet is 2l3uBTotal distance travelled by each particle before they meet is 2l3CAverage velocity during the motion is √3u2DRelative velocity of apporach between any two particles is 3u2 - Question 2 - Select One
Three particles A, B and C are situated at the vertices of an equilateral triangle ABC of side d at time t=0. Each of the particles moves with constant speed v. A always has its velocity along AB, B along BC and C along CA. At what time will the particles meet each other?
At=dvBt=3d2vCt=2d3vDt=0 - Question 3 - Select One
Three particles A,B and C situated at vertices of an equilateral triangle, all moving with same constant speed such that A always move towards B,B always towards C and C always towards A. Initial seperation between each of the particle is a. O is the centroid of the triangle. Distance covered by particle A when it completes one revolution around O is
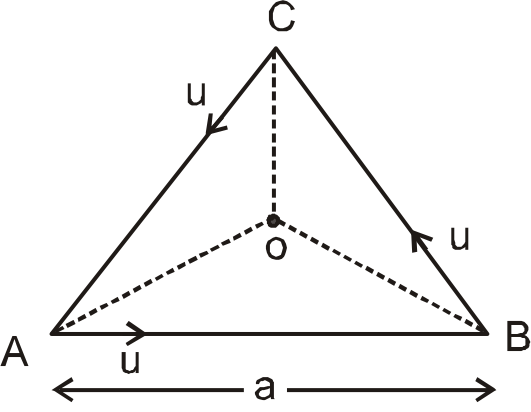 A2a(1−e−2√3π)B2a3(1−e−2√3π)Ca(1+e−2√3π)D2a3(1−e−√3π)
A2a(1−e−2√3π)B2a3(1−e−2√3π)Ca(1+e−2√3π)D2a3(1−e−√3π)
Four particles A,B,CandD are situated at the cornerst of a square ABCD of side aatt−0. Each of particles moves with constant speed (v). A always has its velocity along AB,B along BC,C along CB~andD along DA. At what time will these particles meet each other ?
View Solutionn' number of particles are located at the verticles of a regular polygon of n sides having the edge length 'a'. They all start moving simultaneously with equal constant speed 'v' heading towards each other all the time. How long will the particles take to collide?
View SolutionThree insects A,B and C are situated at the vertices of an equillateral triangle of side 1. the insect A heads towards B,B towards C,C towards A with constant speeds v such that they always remain at the vertices of an equilateral triangle. Find the (a) time of their meeting (b) equation of path traced by one insect relative to the other.
View SolutionFour person K,L,M and N are initally at the corners of a square of side of length d. If every person starts moving, such that K always heads towards L, L heads towards M, M heads directly towards N and N heads towards K, then the four perons will meet after
View SolutionEight people A, B, C, D, E, F, G and Hare placed as shown in the diagram. All are facing in the outward direction. If all of them move anticlockwise to three places then.
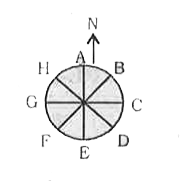 View Solution
View Solution
